Fracture Behavior and Energy Analysis of 3D Concrete Mesostructure under Uniaxial Compression
Abstract
:1. Introduction
2. Numerical Model
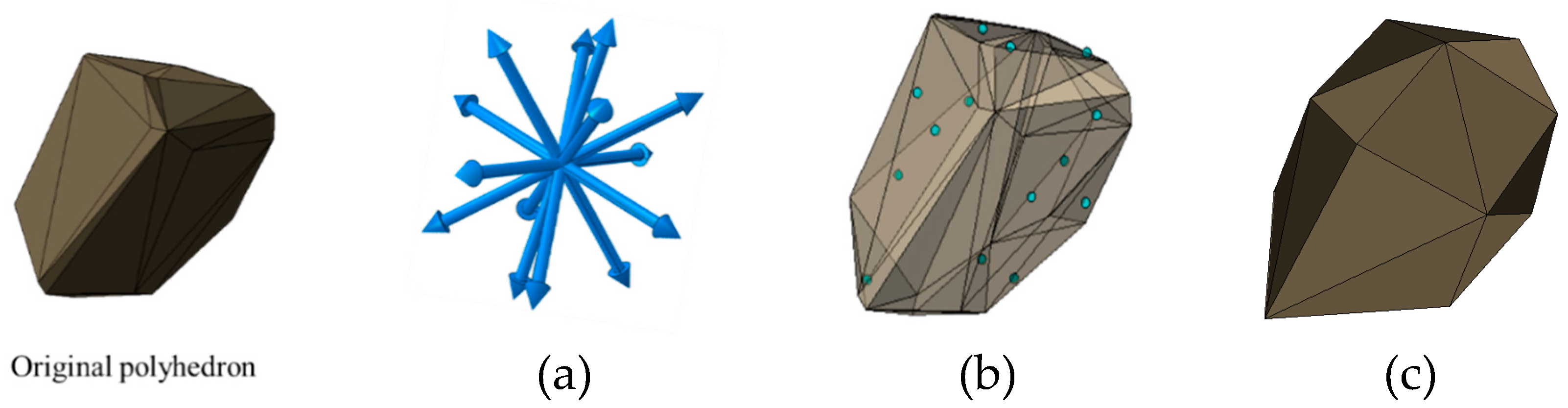
2.1. Generation of Aggregates Based on 3D Voronoi Tessellation
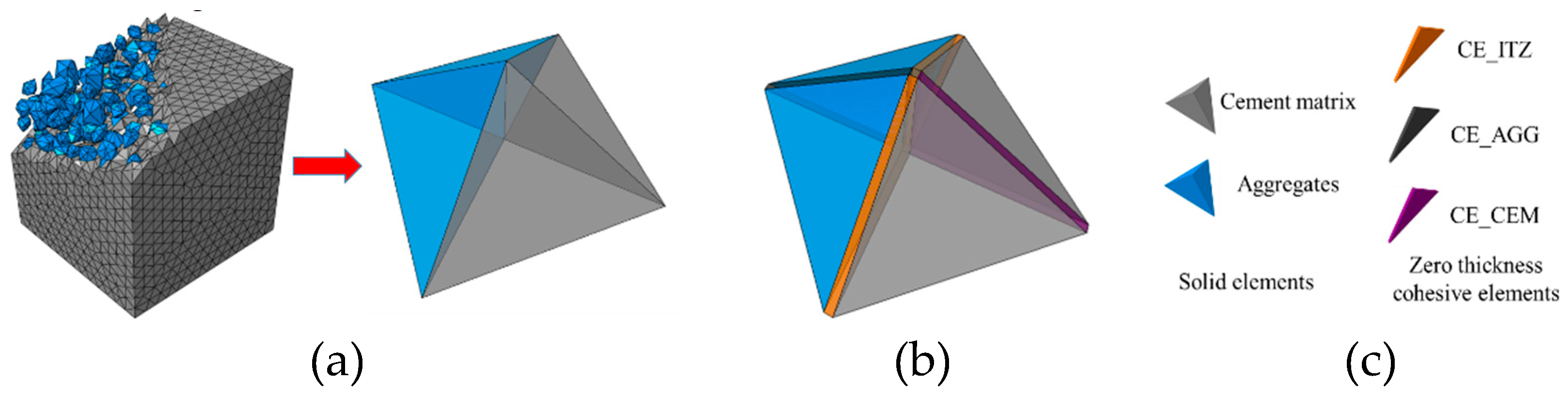
2.2. Application of Cohesive Elements
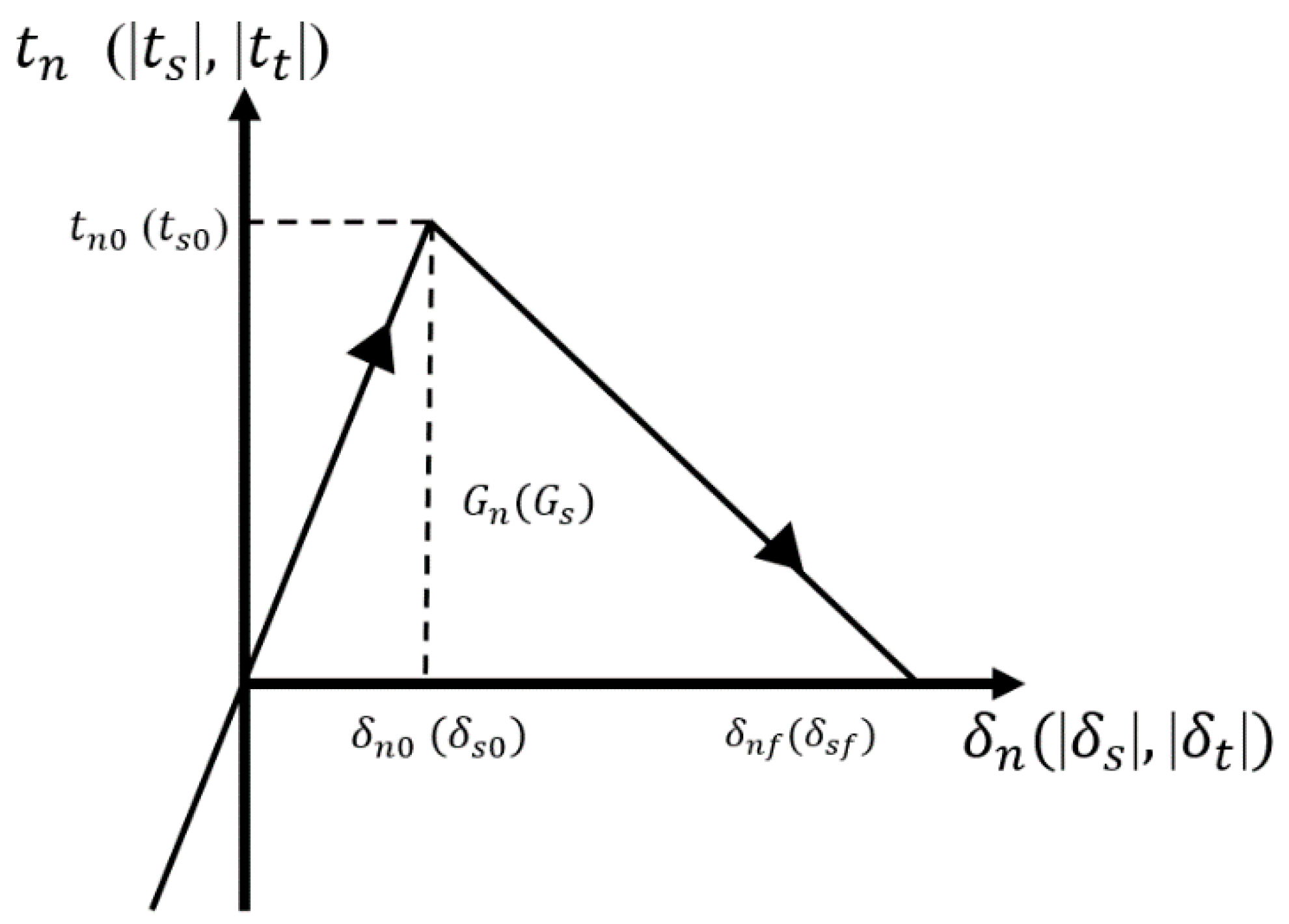
2.3. Modified Constitutive Applied in Cohesive Elements
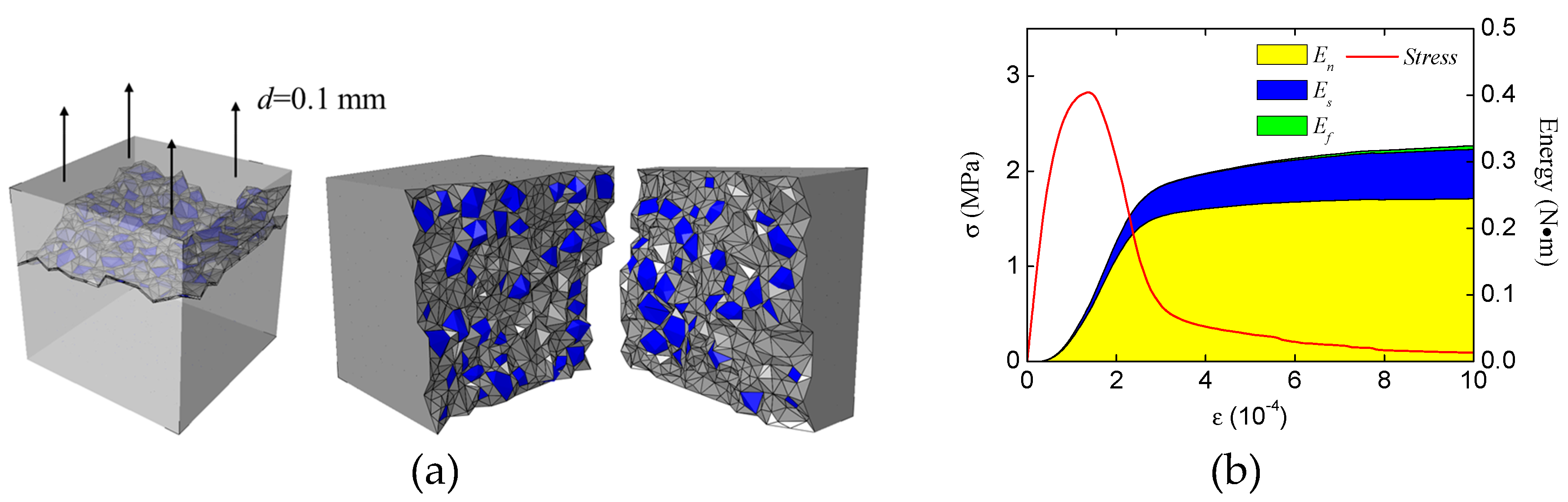
2.4. Calculation of Three Different Types of Energy
3. Numerical Examples and Results
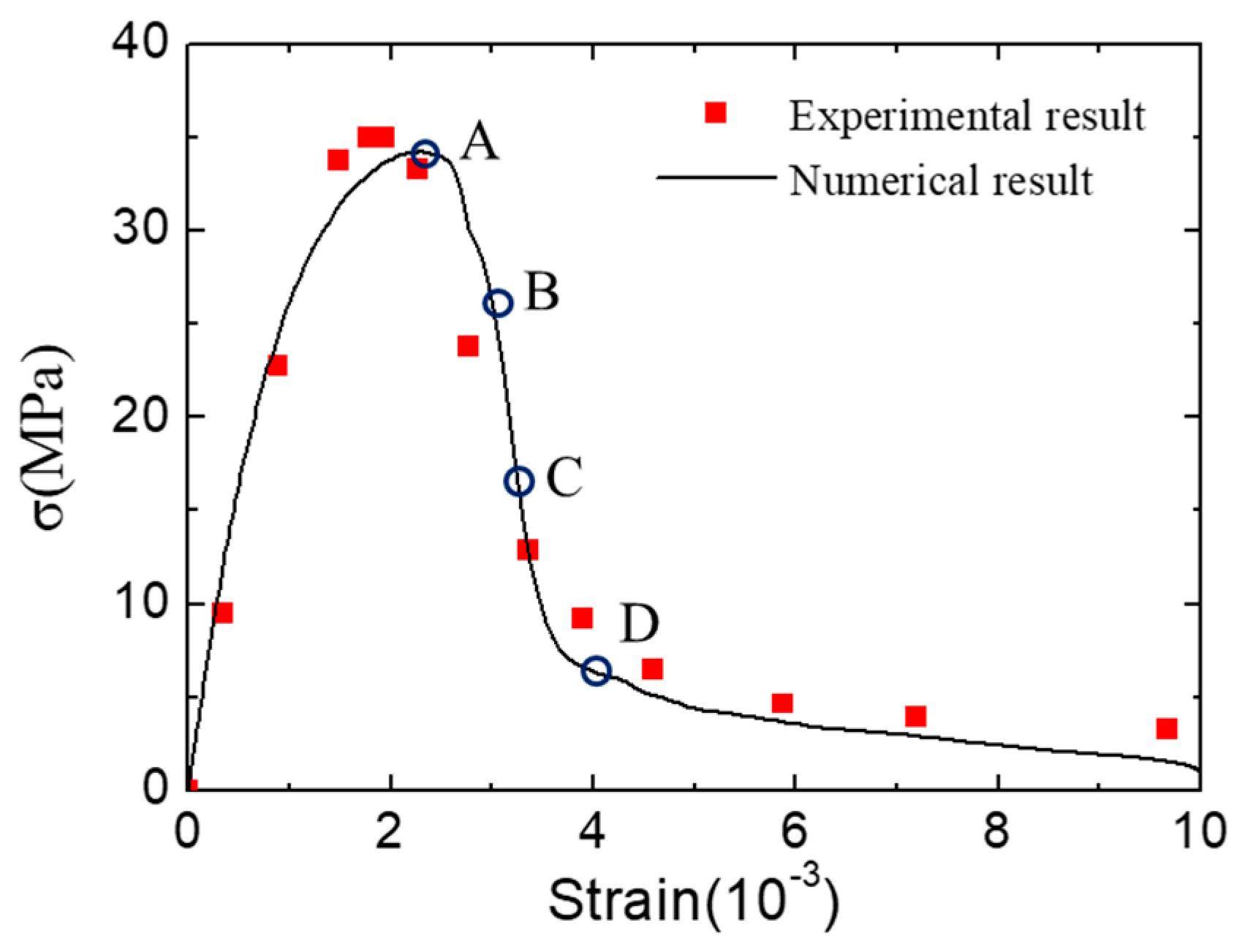
3.1. Input Data of the Finite Element Model
3.2. Effect of Loading Surface Friction Coefficient under Compression
3.2.1. Low-Friction (LF) Condition
3.2.2. High-Friction (HF) Condition
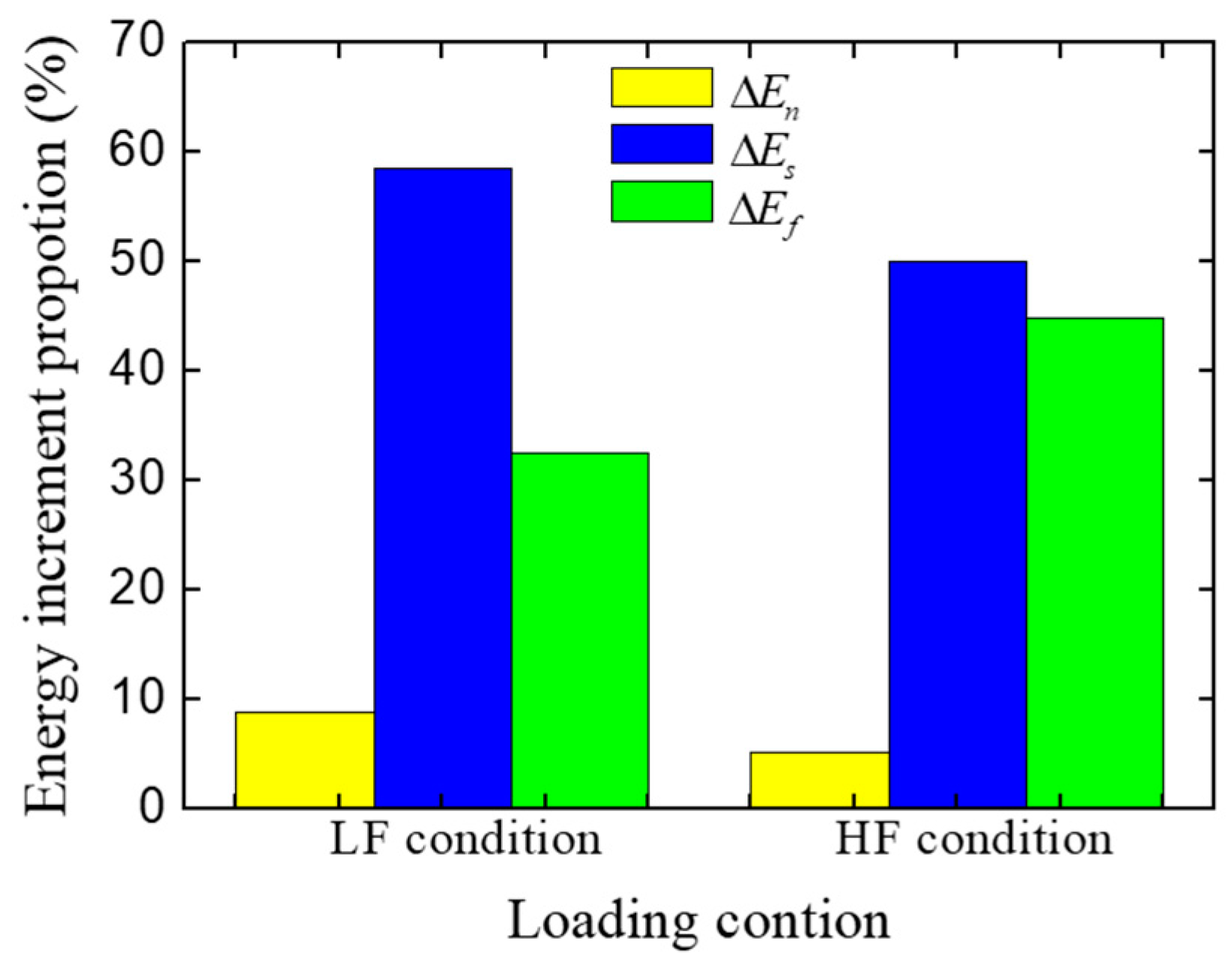
3.2.3. Energy Analysis
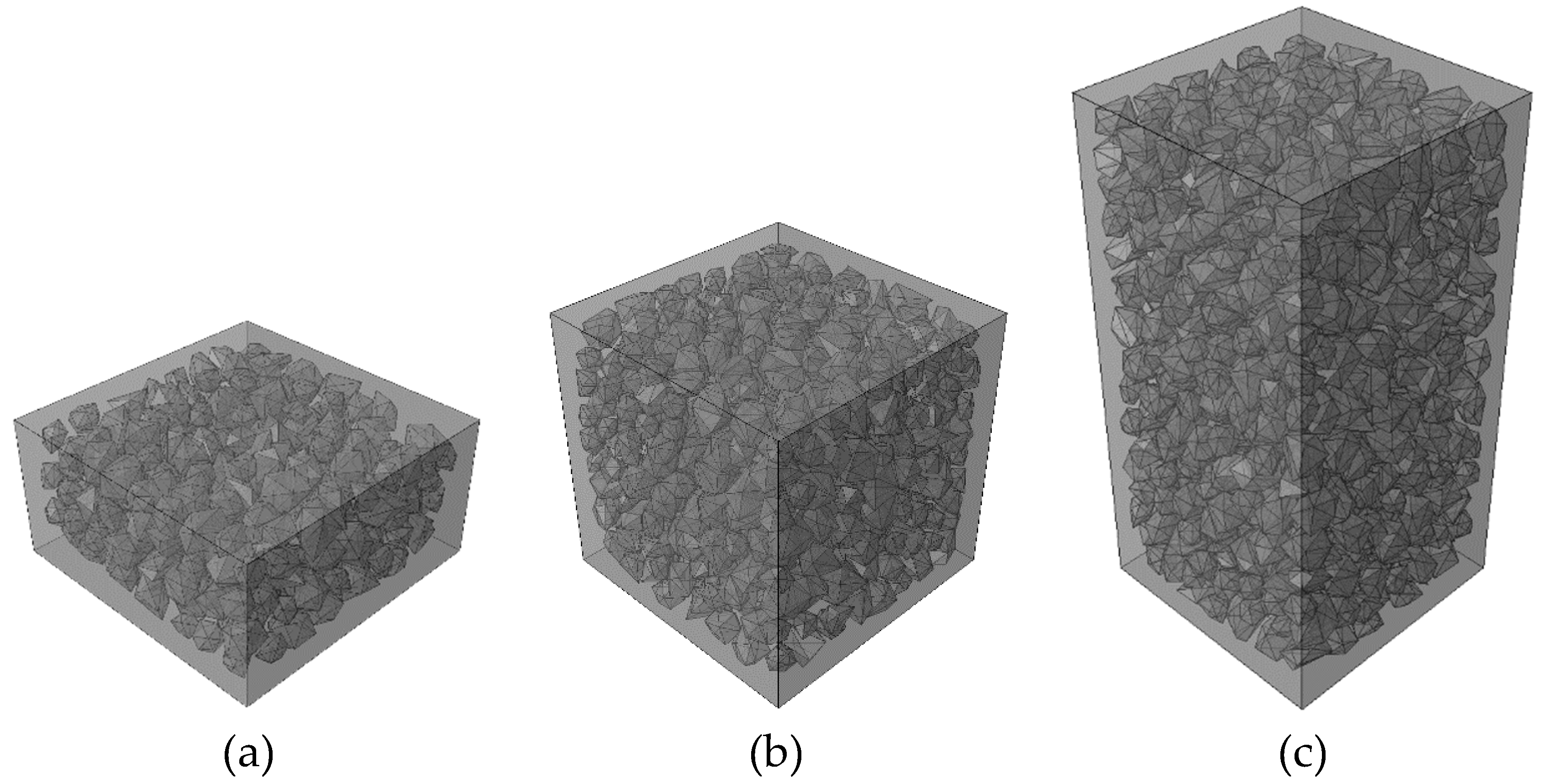
3.3. Effect of Specimen Height
3.3.1. Fracture Pattern and Mechanical Behavior
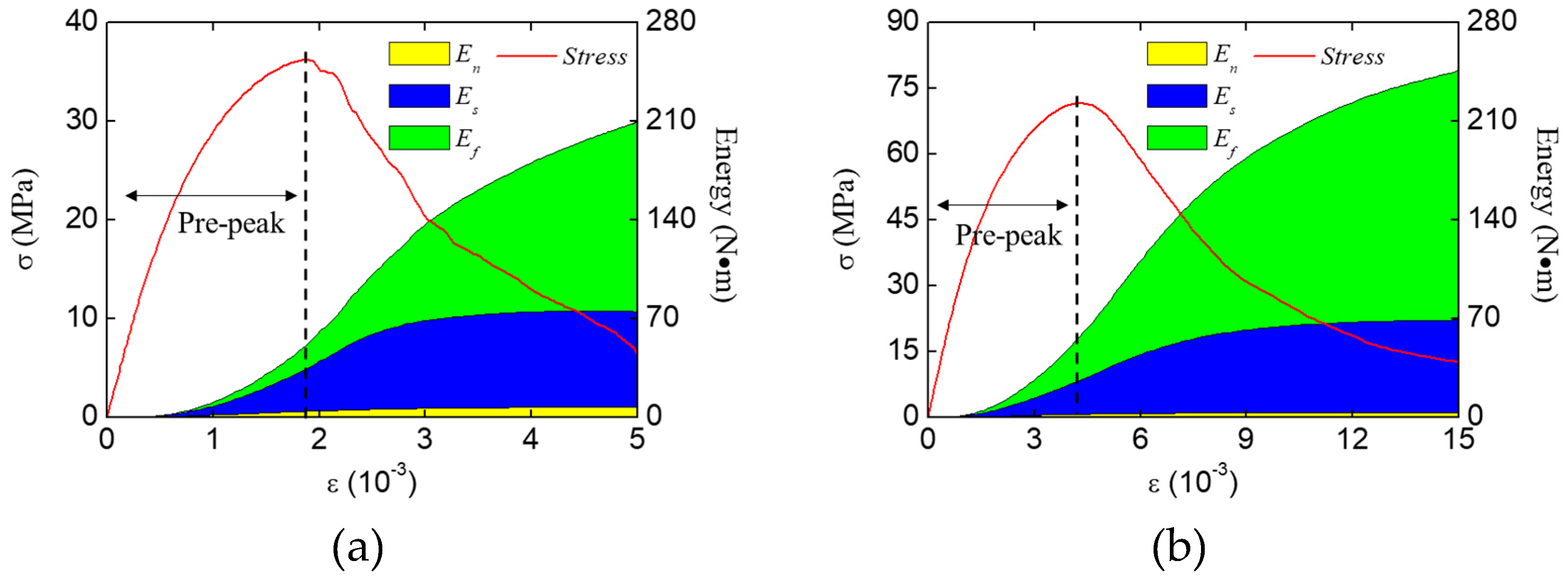
3.3.2. Energy Analysis
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Chaudhuri, P. Multi-scale modeling of fracture in concrete composites. Compos. Part B Eng. 2013, 47, 162–172. [Google Scholar] [CrossRef]
- Jin, L.; Xu, C.; Han, Y.; Du, X. Effect of End Friction on the Dynamic Compressive Mechanical Behavior of Concrete under Medium and Low Strain Rates. Shock Vib. 2016, 2016, 1–20. [Google Scholar] [CrossRef] [Green Version]
- Contrafatto, L.; Cuomo, M.; Greco, L. Meso-scale simulation of concrete multiaxial behaviour. Eur. J. Environ. Civ. Eng. 2017, 21, 896–911. [Google Scholar] [CrossRef]
- Peng, Y.; Wang, Q.; Ying, L.; Kamel, M.M.A.; Peng, H. Numerical Simulation of Dynamic Mechanical Properties of Concrete under Uniaxial Compression. Materials 2019, 12, 643. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.M.; Kwan, A.; Chan, H.C. Mesoscopic study of concrete I: Generation of random aggregate structure and finite element mesh. Comput. Struct. 1999, 70, 533–544. [Google Scholar] [CrossRef]
- Snozzi, L.; Caballero, A.; Molinari, J.F. Influence of the meso-structure in dynamic fracture simulation of concrete under tensile loading. Cem. Concr. Res. 2011, 41, 1130–1142. [Google Scholar] [CrossRef] [Green Version]
- Li, G.; Yu, J.; Cao, P.; Ren, Z. Experimental and numerical investigation on I–II mixed-mode fracture of concrete based on the Monte Carlo random aggregate distribution. Constr. Build. Mater. 2018, 191, 523–534. [Google Scholar] [CrossRef]
- Saksala, T. Numerical modelling of concrete fracture processes under dynamic loading: Meso-mechanical approach based on embedded discontinuity finite elements. Eng. Fract. Mech. 2018, 201, 282–297. [Google Scholar] [CrossRef]
- Yang, Z.; Ren, W.; Sharma, R.; McDonald, S.; Mostafavi, M.; Vertyagina, Y.; Marrow, T.J. In-situ X-ray computed tomography characterisation of 3D fracture evolution and image-based numerical homogenisation of concrete. Cem. Concr. Compos. 2017, 75, 74–83. [Google Scholar] [CrossRef]
- du Plessis, A.; Boshoff, W.P. A review of X-ray computed tomography of concrete and asphalt construction materials. Constr. Build. Mater. 2019, 199, 637–651. [Google Scholar] [CrossRef]
- Bernachy-Barbe, F.; Bary, B. Effect of aggregate shapes on local fields in 3D mesoscale simulations of the concrete creep behavior. Finite Elem. Anal. Des. 2019, 156, 13–23. [Google Scholar] [CrossRef]
- Shen, L.; Ren, Q.; Xia, N.; Sun, L.; Xia, X. Mesoscopic numerical simulation of effective thermal conductivity of tensile cracked concrete. Constr. Build. Mater. 2015, 95, 467–475. [Google Scholar] [CrossRef]
- Zhang, C.; Zhou, W.; Ma, G.; Hu, C.; Li, S. A meso-scale approach to modeling thermal cracking of concrete induced by water-cooling pipes. Comput. Concr. 2015, 15, 485–501. [Google Scholar] [CrossRef]
- Chen, G.; Hao, Y.; Hao, H. 3D meso-scale modelling of concrete material in spall tests. Mater. Struct. 2015, 48, 1887–1899. [Google Scholar] [CrossRef]
- Chen, H.; Xu, B.; Mo, Y.L.; Zhou, T. Behavior of meso-scale heterogeneous concrete under uniaxial tensile and compressive loadings. Constr. Build. Mater. 2018, 178, 418–431. [Google Scholar] [CrossRef]
- Jin, L.; Yu, W.; Du, X.; Zhang, S.; Li, D. Meso-scale modelling of the size effect on dynamic compressive failure of concrete under different strain rates. Int. J. Impact Eng. 2019, 125, 1–12. [Google Scholar] [CrossRef]
- Van Mier, J.G.; van Vliet, M.R.; Wang, T.K. Fracture mechanisms in particle composites: Statistical aspects in lattice type analysis. Mech. Mater. 2002, 34, 705–724. [Google Scholar] [CrossRef]
- Cusatis, G.; Bažant, Z.P.; Cedolin, L. Confinement-shear lattice model for concrete damage in tension and compression: I. Theory. J. Eng. Mech. 2003, 129, 1439–1448. [Google Scholar] [CrossRef]
- Grassl, P.; Grégoire, D.; Rojas Solano, L.; Pijaudier-Cabot, G. Meso-scale modelling of the size effect on the fracture process zone of concrete. Int. J. Solids Struct. 2012, 49, 1818–1827. [Google Scholar] [CrossRef]
- Montero-Chacón, F.; Cifuentes, H.; Medina, F. Mesoscale Characterization of Fracture Properties of Steel Fiber-Reinforced Concrete Using a Lattice–Particle Model. Materials 2017, 10, 207. [Google Scholar] [CrossRef]
- Karavelić, E.; Nikolić, M.; Ibrahimbegovic, A.; Kurtović, A. Concrete meso-scale model with full set of 3D failure modes with random distribution of aggregate and cement phase. Part I: Formulation and numerical implementation. Comput. Methods Appl. Mech. Eng. 2019, 344, 1051–1072. [Google Scholar] [Green Version]
- Wriggers, P.; Moftah, S.O. Mesoscale models for concrete: Homogenisation and damage behaviour. Finite Elem. Anal. Des. 2006, 42, 623–636. [Google Scholar] [CrossRef]
- Jin, L.; Du, X.; Ma, G. Macroscopic effective moduli and tensile strength of saturated concrete. Cem. Concr. Res. 2012, 42, 1590–1600. [Google Scholar] [CrossRef]
- Du, X.; Jin, L.; Ma, G. Meso-element equivalent method for the simulation of macro mechanical properties of concrete. Int. J. Damage Mech. 2013, 22, 617–642. [Google Scholar] [CrossRef]
- Du, X.; Jin, L.; Ma, G. Macroscopic effective mechanical properties of porous dry concrete. Cem. Concr. Res. 2013, 44, 87–96. [Google Scholar] [CrossRef]
- Montero-Chacón, F.; Marín-Montín, J.; Medina, F. Mesomechanical characterization of porosity in cementitious composites by means of a voxel-based finite element model. Comput. Mater. Sci. 2014, 90, 157–170. [Google Scholar] [CrossRef]
- Camacho, G.T.; Ortiz, M. Computational modelling of impact damage in brittle materials. Int. J. Solids Struct. 1996, 33, 2899–2938. [Google Scholar] [CrossRef]
- Caballero, A.; Carol, I.; López, C.M. A meso-level approach to the 3D numerical analysis of cracking and fracture of concrete materials. Fatigue Fract. Eng. Mater. Struct. 2006, 29, 979–991. [Google Scholar] [CrossRef]
- Caballero, A.; López, C.M.; Carol, I. 3D meso-structural analysis of concrete specimens under uniaxial tension. Comput. Methods Appl. Mech. Eng. 2006, 195, 7182–7195. [Google Scholar] [CrossRef]
- López, C.M.; Carol, I.; Aguado, A. Meso-structural study of concrete fracture using interface elements. I: Numerical model and tensile behavior. Mater. Struct. 2008, 41, 583–599. [Google Scholar]
- Yang, Z.J.; Su, X.T.; Chen, J.F.; Liu, G.H. Monte Carlo simulation of complex cohesive fracture in random heterogeneous quasi-brittle materials. Int. J. Solids Struct. 2009, 46, 3222–3234. [Google Scholar] [CrossRef]
- Su, X.T.; Yang, Z.J.; Liu, G.H. Monte Carlo simulation of complex cohesive fracture in random heterogeneous quasi-brittle materials: A 3D study. Int. J. Solids Struct. 2010, 47, 2336–2345. [Google Scholar] [CrossRef]
- Wang, X.F.; Yang, Z.J.; Yates, J.R.; Jivkov, A.P.; Zhang, C. Monte Carlo simulations of mesoscale fracture modelling of concrete with random aggregates and pores. Constr. Build. Mater. 2015, 75, 35–45. [Google Scholar] [CrossRef]
- Wang, X.; Yang, Z.; Jivkov, A.P. Monte Carlo simulations of mesoscale fracture of concrete with random aggregates and pores: A size effect study. Constr. Build. Mater. 2015, 80, 262–272. [Google Scholar] [CrossRef]
- Wang, X.; Zhang, M.; Jivkov, A.P. Computational technology for analysis of 3D meso-structure effects on damage and failure of concrete. Int. J. Solids Struct. 2016, 80, 310–333. [Google Scholar] [CrossRef]
- Nitka, M.; Tejchman, J. Modelling of concrete behaviour in uniaxial compression and tension with DEM. Granul. Matter 2015, 17, 145–164. [Google Scholar] [CrossRef]
- Rangari, S.; Murali, K.; Deb, A. Effect of meso-structure on strength and size effect in concrete under compression. Eng. Fract. Mech. 2018, 195, 162–185. [Google Scholar] [CrossRef]
- Nguyen, T.T.; Bui, H.H.; Ngo, T.D.; Nguyen, G.D.; Kreher, M.U.; Darve, F. A micromechanical investigation for the effects of pore size and its distribution on geopolymer foam concrete under uniaxial compression. Eng. Fract. Mech. 2019, 209, 228–244. [Google Scholar] [CrossRef]
- Bolander, J.E.; Saito, S. Fracture analyses using spring networks with random geometry. Eng. Fract. Mech. 1998, 61, 569–591. [Google Scholar] [CrossRef]
- Bolander, J.E., Jr.; Berton, S. Simulation of shrinkage induced cracking in cement composite overlays. Cem. Concr. Compos. 2004, 26, 861–871. [Google Scholar] [CrossRef]
- Ueda, T.; Hasan, M.; Nagai, K.; Sato, Y.; Wang, L. Mesoscale simulation of influence of frost damage on mechanical properties of concrete. J. Mater. Civ. Eng. 2009, 21, 244–252. [Google Scholar] [CrossRef]
- Abaqus, I. Abaqus documentation. Version 2014, 6, 1–5. [Google Scholar]
- Du, Q.; Gunzburger, M. Grid generation and optimization based on centroidal Voronoi tessellations. Appl. Math. Comput. 2002, 133, 591–607. [Google Scholar] [CrossRef]
- Falco, S.; Jiang, J.; De Cola, F.; Petrinic, N. Generation of 3D polycrystalline microstructures with a conditioned Laguerre-Voronoi tessellation technique. Comput. Mater. Sci. 2017, 136, 20–28. [Google Scholar] [CrossRef]
- Zhang, J.; Wang, Z.; Yang, H.; Wang, Z.; Shu, X. 3D meso-scale modeling of reinforcement concrete with high volume fraction of randomly distributed aggregates. Constr. Build. Mater. 2018, 164, 350–361. [Google Scholar] [CrossRef]
- van Vliet, M.A.; van Mier, J.M. Experimental investigation of concrete fracture under uniaxial compression. Mech. Cohesive-Frict. Mater. Int. J. Exp. Model. Comput. Mater. Struct. 1996, 1, 115–127. [Google Scholar] [CrossRef]





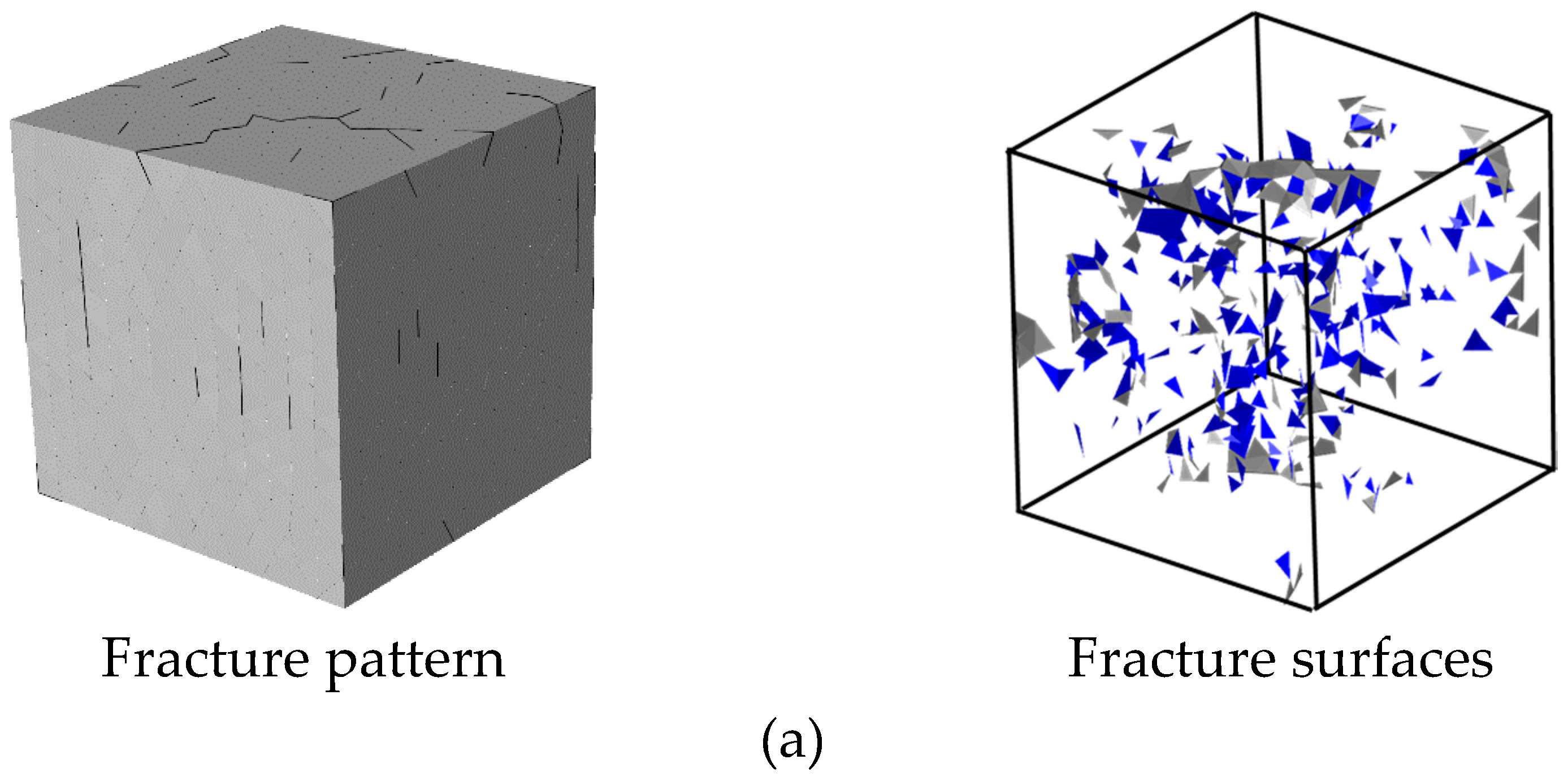



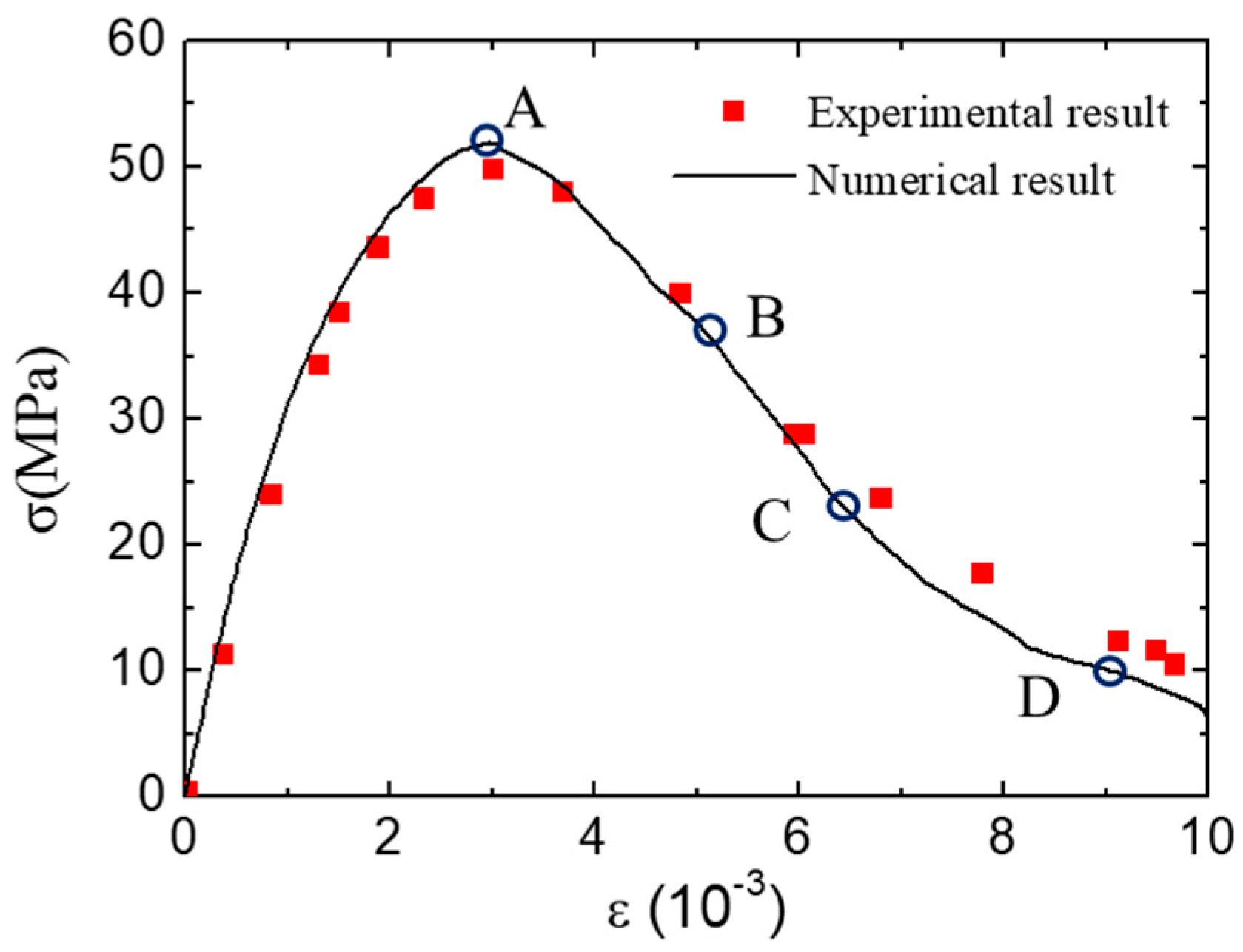
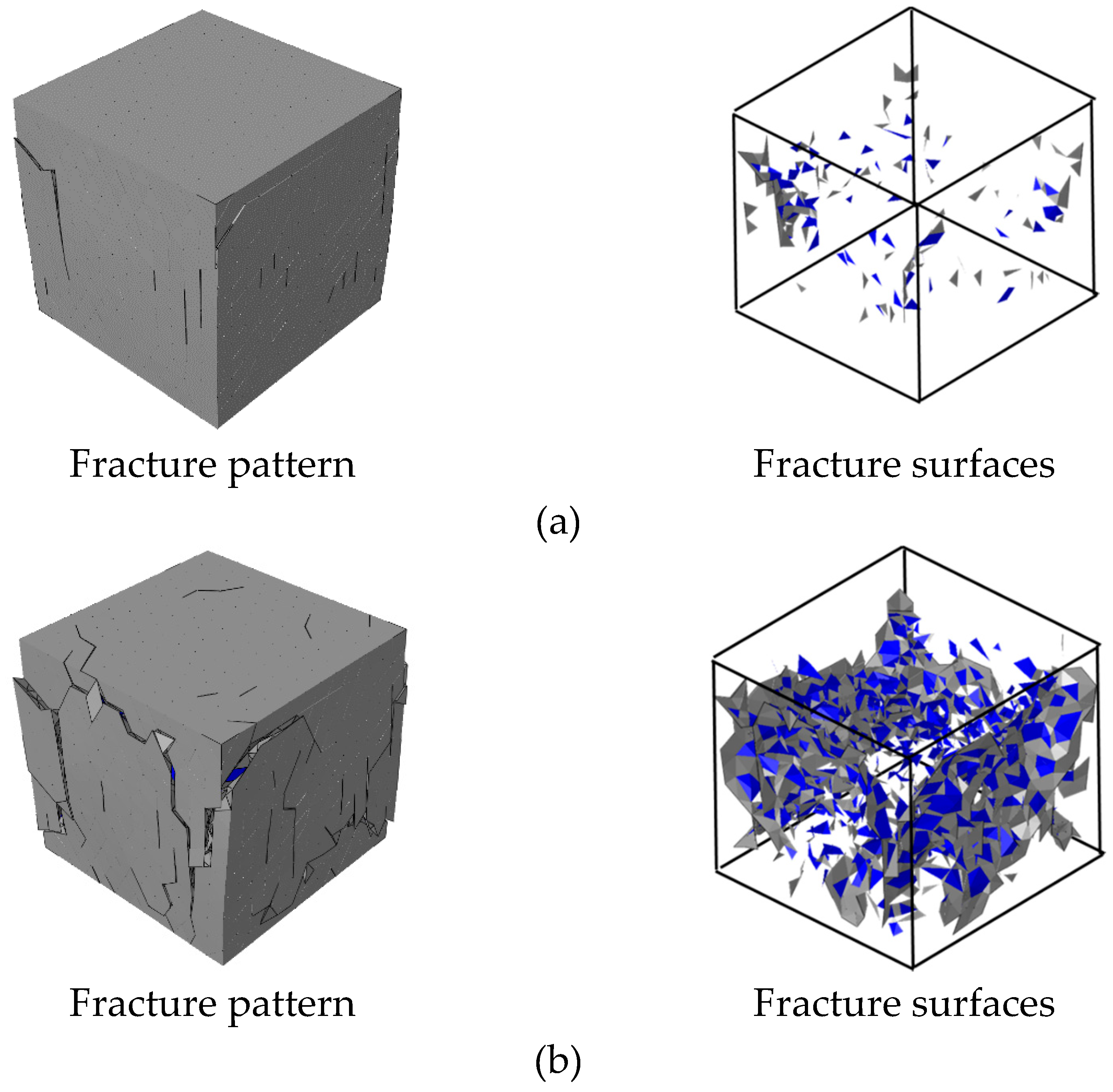



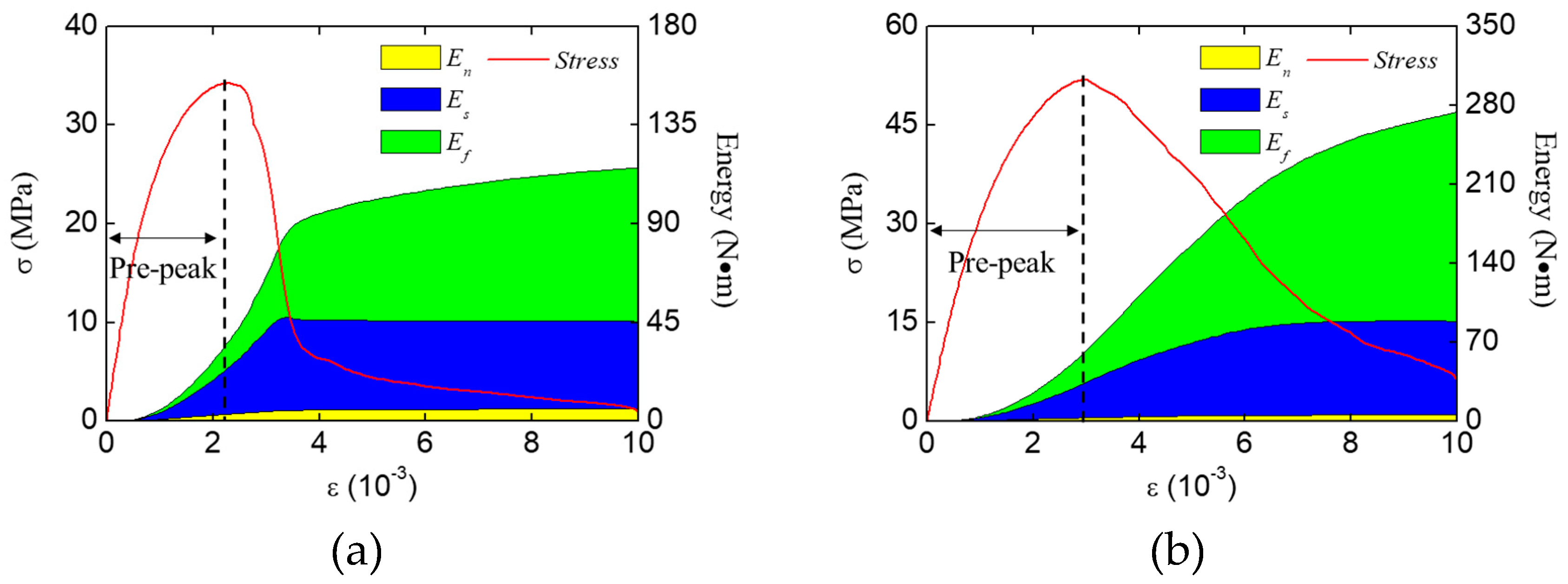




| Grain Size (mm) | Unit Content (kg/m3) | Volume Content (%) |
|---|---|---|
| 8~4 | 540 | 19.29 |
| 4~2 | 363 | 12.91 |
| 2~1 | 272 | 9.71 |
| 1~0.5 | 272 | 9.71 |
| 0.5~0.25 | 234 | 8.35 |
| Loading Condition | Energy Type | Energy Value (N∙m) | Energy Proportion (%) |
|---|---|---|---|
| LF condition | 3.31 | 8.88 | |
| 21.85 | 58.57 | ||
| 12.14 | 32.55 | ||
| HF condition | 3.12 | 5.12 | |
| 30.47 | 49.98 | ||
| 27.37 | 44.90 |
| Height of Specimen | Energy Type | Energy Value (N∙m) | Energy Proportion (%) |
|---|---|---|---|
| 200 mm | 4.31 | 8.49 | |
| 29.72 | 58.51 | ||
| 16.77 | 33.00 | ||
| 50 mm | 2.26 | 3.96 | |
| 24.05 | 42.14 | ||
| 30.77 | 53.90 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, Y.; Hu, S.; Gu, Z.; Sun, Y. Fracture Behavior and Energy Analysis of 3D Concrete Mesostructure under Uniaxial Compression. Materials 2019, 12, 1929. https://doi.org/10.3390/ma12121929
Huang Y, Hu S, Gu Z, Sun Y. Fracture Behavior and Energy Analysis of 3D Concrete Mesostructure under Uniaxial Compression. Materials. 2019; 12(12):1929. https://doi.org/10.3390/ma12121929
Chicago/Turabian StyleHuang, Yiqun, Shaowei Hu, Zi Gu, and Yueyang Sun. 2019. "Fracture Behavior and Energy Analysis of 3D Concrete Mesostructure under Uniaxial Compression" Materials 12, no. 12: 1929. https://doi.org/10.3390/ma12121929






