Grain-Size Distribution Effects on the Attenuation of Laser-Generated Ultrasound in α-Titanium Alloy
Abstract
:1. Introduction
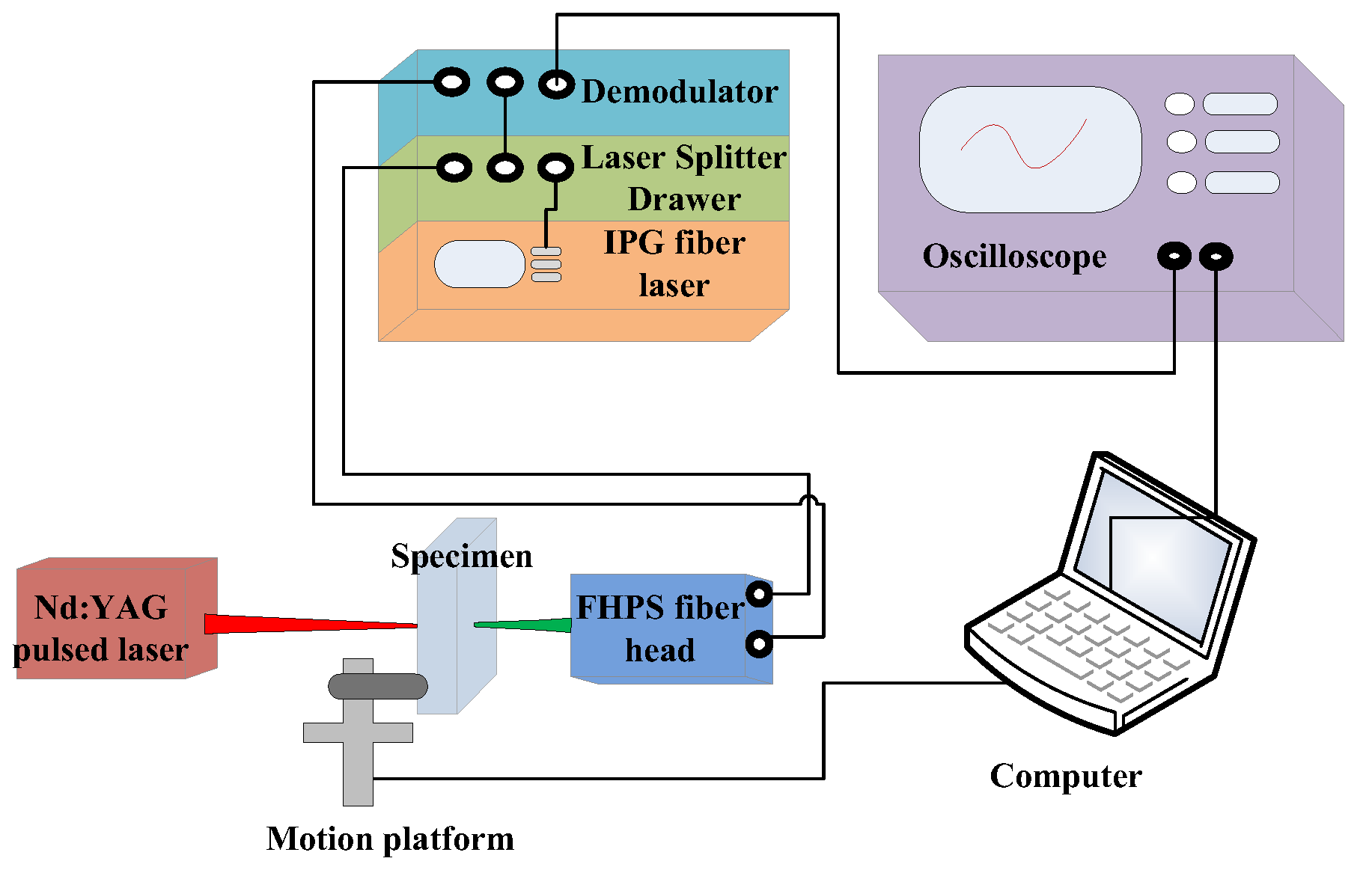
2. Materials and Methods
3. Results
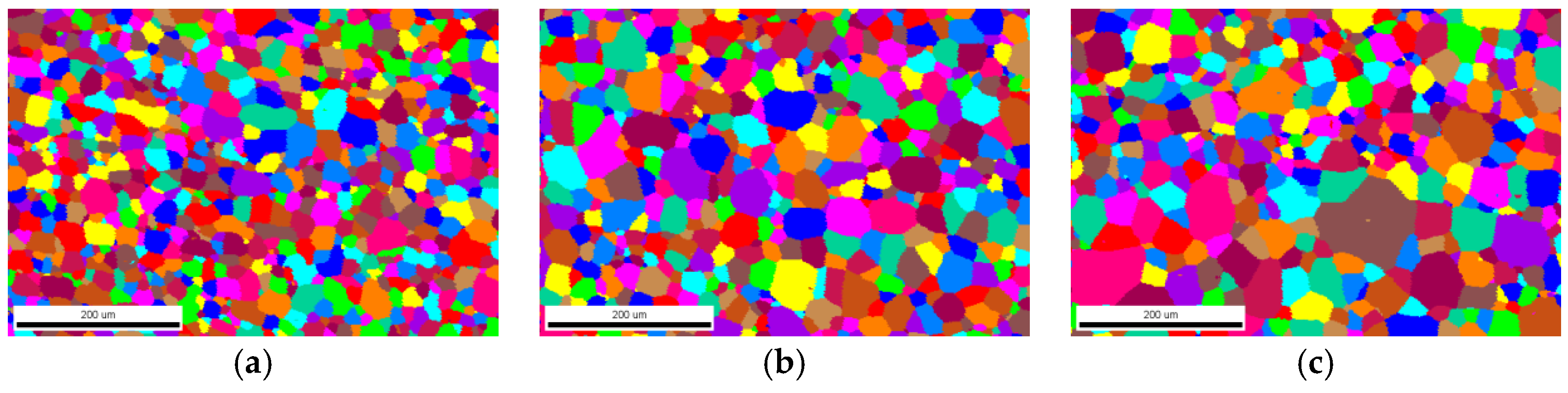
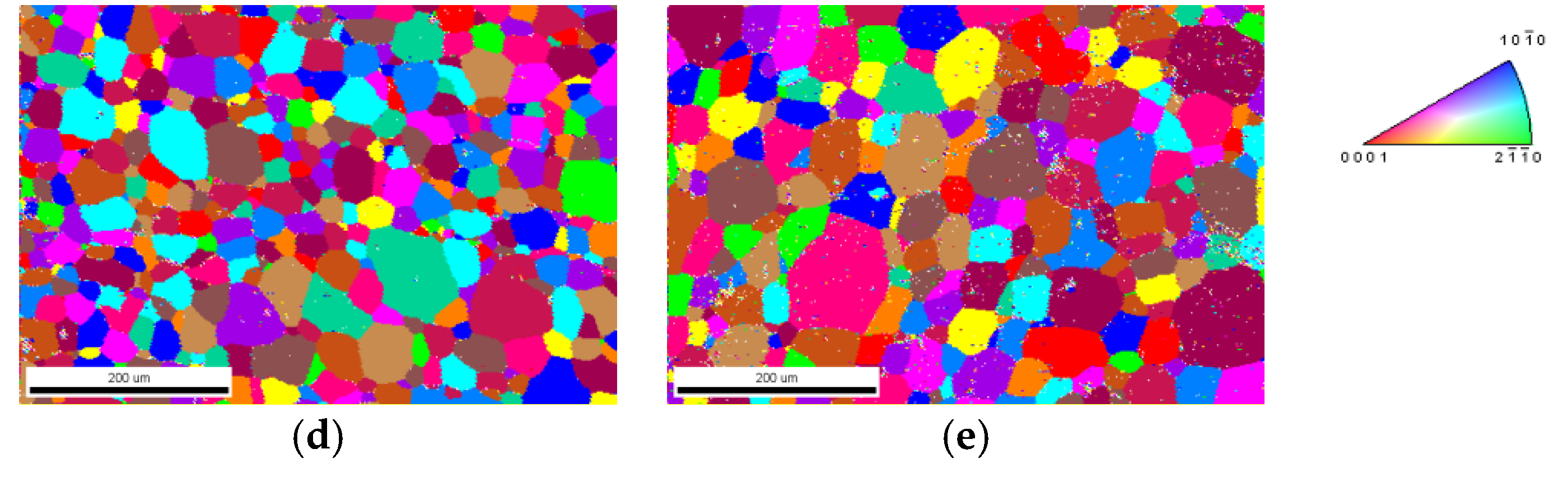
3.1. Metallographical Observations
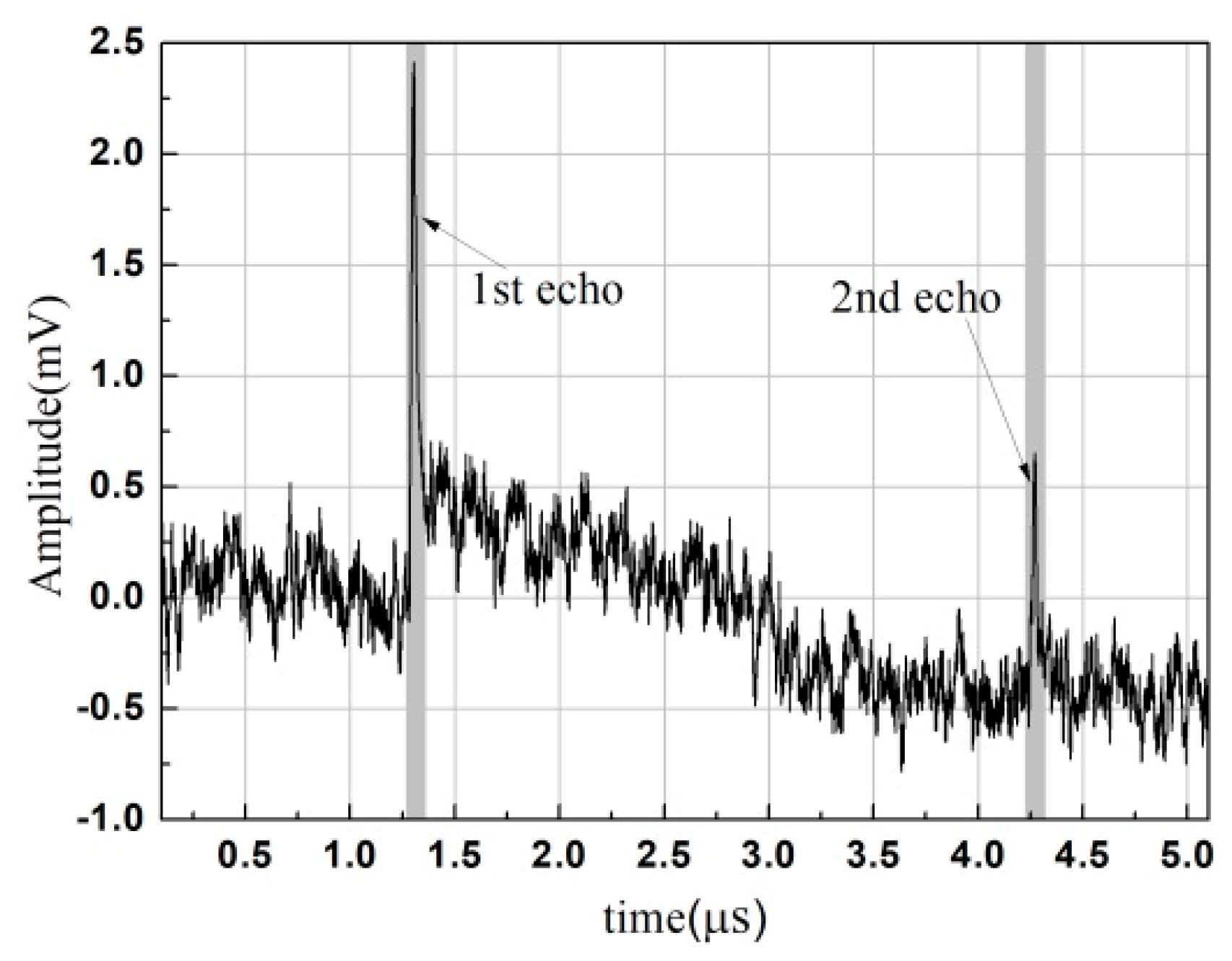
3.2. Ultrasonic Attenuation Measurements
3.3. Grain Size Characterization
4. Discussions
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Richeton, T.; Wagner, F.; Chen, C.; Toth, L. Combined effects of texture and grain size distribution on the tensile behavior of α-titanium. Materials 2018, 11, 1088. [Google Scholar] [CrossRef] [PubMed]
- Berbenni, S.; Favier, V.; Berveiller, M. Micro-macro modelling of the effects of the grain size distribution on the plastic flow stress of heterogeneous materials. Comput. Mater. Sci. 2007, 39, 96–105. [Google Scholar] [CrossRef]
- Berbenni, S.; Favier, V.; Berveiller, M. Impact of the grain size distribution on the yield stress of heterogeneous materials. Int. J. Plast. 2007, 23, 114–142. [Google Scholar] [CrossRef]
- Bucki, J.J.; Kurzydłowski, K.J. Analysis of the effect of grain size uniformity on the flow stress of polycrystals: Part I: Studies of the relationship between the variance of grain volume and the variance of the grain area on polycrystal cross section. Mater. Charact. 1992, 29, 365–374. [Google Scholar] [CrossRef]
- Smith, C.J.; Stephens, J.D.; Hancock, B.C.; Vahdat, A.S.; Cetinkaya, C. Acoustic assessment of mean grain size in pharmaceutical compacts. Int. J. Pharm. 2011, 419, 137–146. [Google Scholar] [CrossRef] [PubMed]
- Gil, F.J.; Planell, J.A. Behaviour of normal grain growth kinetics in single phase titanium and titanium alloys. Mater. Sci. Eng. A-Struct. 2000, 283, 17–24. [Google Scholar] [CrossRef]
- Zeng, F.; Agnew, S.R.; Raeisinia, B.; Myneni, G.R. Ultrasonic attenuation due to grain boundary scattering in pure niobium. J. Nondestruct. Eval. 2010, 29, 93–103. [Google Scholar] [CrossRef]
- Stanke, F.E.; Kino, G.S. A unified theory for elastic wave propagation in polycrystalline materials. J. Acoust. Soc. Am. 1984, 75, 665–681. [Google Scholar] [CrossRef]
- Karal, F.C., Jr.; Keller, J.B. Elastic, electromagnetic, and other waves in a random medium. J. Math. Phys. 1964, 5, 537–547. [Google Scholar] [CrossRef]
- Bhatia, A.B. Scattering of High-Frequency Sound Waves in Polycrystalline Materials. J. Acoust. Soc. Am. 1959, 31, 16–23. [Google Scholar] [CrossRef]
- Hirsekorn, S. The scattering of ultrasonic waves by polycrystals. J. Acoust. Soc. Am. 1982, 72, 1021–1031. [Google Scholar] [CrossRef]
- Weaver, R.L. Diffusivity of ultrasound in polycrystals. J. Mech. Phys. Solids 1990, 38, 55–86. [Google Scholar] [CrossRef]
- Kube, C.M. Iterative solution to bulk wave propagation in polycrystalline materials. J. Acoust. Soc. Am. 2017, 141, 1804–1811. [Google Scholar] [CrossRef] [PubMed]
- Turner, J.A. Elastic wave propagation and scattering in heterogeneous, anisotropic media: Textured polycrystalline materials. J. Acoust. Soc. Am. 1999, 106, 541–552. [Google Scholar] [CrossRef] [Green Version]
- Yang, L.; Lobkis, O.I.; Rokhlin, S.I. Shape effect of elongated grains on ultrasonic attenuation in polycrystalline materials. Ultrasonics 2011, 51, 697–708. [Google Scholar] [CrossRef] [PubMed]
- Yang, L.; Lobkis, O.I.; Rokhlin, S.I. Explicit model for ultrasonic attenuation in equiaxial hexagonal polycrystalline materials. Ultrasonics 2011, 51, 303–309. [Google Scholar] [CrossRef]
- Papadakis, E.P. Grain-Size Distribution in Metals and Its Influence on Ultrasonic Attenuation Measurements. J. Acoust. Soc. Am. 1961, 33, 1616–1621. [Google Scholar] [CrossRef]
- Papadakis, E.P. From micrograph to grain-size distribution with ultrasonic applications. J. Appl. Phys. 1964, 35, 1586–1594. [Google Scholar] [CrossRef]
- Nicoletti, D.; Kasper, D. Ultrasonic attenuation based on the roney generalized theory and multiple power-law grain-size distributions. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 1994, 41, 144–149. [Google Scholar] [CrossRef]
- Anderson, A.; Nicoletti, D. Determination of Grain-Size Distributions from Ultrasonic Attenuation. In Review of Progress in QNDE; Springer: Boston, MA, USA, 1996; pp. 1503–1507. [Google Scholar] [Green Version]
- Smith, R.L. The effect of grain size distribution on the frequency dependence of the ultrasonic attenuation in polycrystalline materials. Ultrasonics 1982, 20, 211–214. [Google Scholar] [CrossRef]
- Arguelles, A.P.; Turner, J.A. Ultrasonic attenuation of polycrystalline materials with a distribution of grain sizes. J. Acoust. Soc. Am. 2017, 141, 4347–4353. [Google Scholar] [CrossRef] [PubMed]
- Rhines, F.N.; Patterson, B.R. Effect of the degree of prior cold work on the grain volume distribution and the rate of grain growth of recrystallized aluminum. Metall. Trans. A 1982, 13, 985–993. [Google Scholar] [CrossRef]
- Thompson, R.B.; Margetan, F.J.; Haldipur, P.; Yu, L.; Li, A.; Panetta, P.; Wasan, H. Scattering of elastic waves in simple and complex polycrystals. Wave Motion 2008, 45, 655–674. [Google Scholar] [CrossRef]
- Lindh-Ulmgren, E.; Ericsson, M.; Artymowicz, D.; Hutchinson, W.B. Laser-ultrasonics as a technique to study recrystallisation and grain growth. Mater. Sci. Forum 2004, 467, 1353–1362. [Google Scholar] [CrossRef]
- Kube, C.M. Attenuation of laser generated ultrasound in steel at high temperatures: Comparison of theory and experimental measurements. Ultrasonics 2016, 70, 238–240. [Google Scholar] [CrossRef] [PubMed]
- Zhang, X.G.; Simpson, W.A.; Vitek, J.M.; Barnard, D.J.; Tweed, L.J.; Foley, J. Ultrasonic attenuation due to grain boundary scattering in copper and copper-aluminum. J. Acoust. Soc. Am. 2004, 116, 109–116. [Google Scholar] [CrossRef]
- Garcin, T.; Schmitt, J.H.; Militzer, M. In-situ laser ultrasonic grain size measurement in superalloy INCONEL 718. J. Alloy. Compd. 2016, 670, 329–336. [Google Scholar] [CrossRef]
- Okulov, I.V.; Volegov, A.S.; Attar, H.; Bönisch, M.; Ehtemam-Haghighi, S.; Calin, M.; Eckert, J. Composition optimization of low modulus and high-strength TiNb-based alloys for biomedical applications. J. Mech. Behav. Biomed. Mater. 2017, 65, 866–871. [Google Scholar] [CrossRef] [PubMed]
- Ehtemam-Haghighi, S.; Prashanth, K.G.; Attar, H.; Chaubey, A.K.; Cao, G.H.; Zhang, L.C. Evaluation of mechanical and wear properties of TixNb7Fe alloys designed for biomedical applications. Mater. Des. 2016, 111, 592–599. [Google Scholar] [CrossRef]
- Okazaki, K.; Conrad, H. Grain size distribution in recrystallized alpha-titanium. Trans. Jpn. Inst. Met. 1972, 13, 198–204. [Google Scholar] [CrossRef]
- Sarkar, S.; Moreau, A.; Militzer, M.; Poole, W.J. Evolution of austenite recrystallization and grain growth using laser ultrasonics. Metall. Mater. Trans. A 2008, 39, 897–907. [Google Scholar] [CrossRef]
- Du, H.; Turner, J.A. Ultrasonic attenuation in pearlitic steel. Ultrasonics 2014, 54, 882–887. [Google Scholar] [CrossRef] [PubMed]
- Krüger, S.; Lamouche, G.; Monchalin, J.P.; Kolarik, R.; Jeskey, G.; Choquet, M. On-line monitoring of wall thickness and austenite grain size on a seamless tubing production line at the timken company. Iron Steel Technol. 2005, 2, 1–44. [Google Scholar]
- Zhang, C.; Li, X.; Song, Y.; Han, X.; Han, J. Evaluating the grain size in curved components using the ultrasonic attenuation method with diffraction correction. NDT & E Int. 2016, 84, 20–26. [Google Scholar] [CrossRef]
- Margetan, F.J.; Yu, L.; Thompson, R.B. Computation of Grain-Noise Scattering Coefficients for Ultrasonic Pitch/Catch Inspections of Metals. AIP Conf. Proc. 2005, 760, 1300–1307. [Google Scholar]



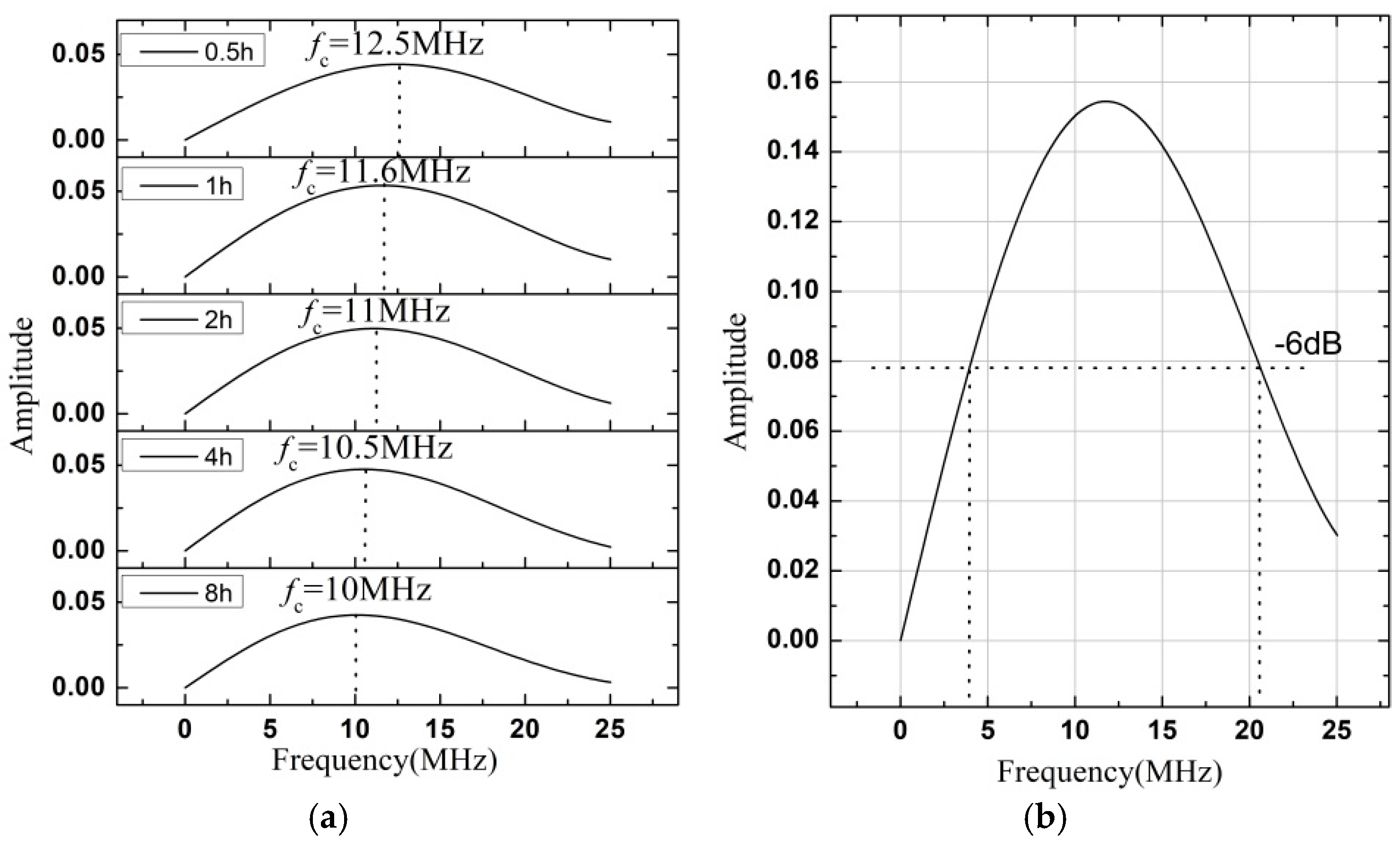
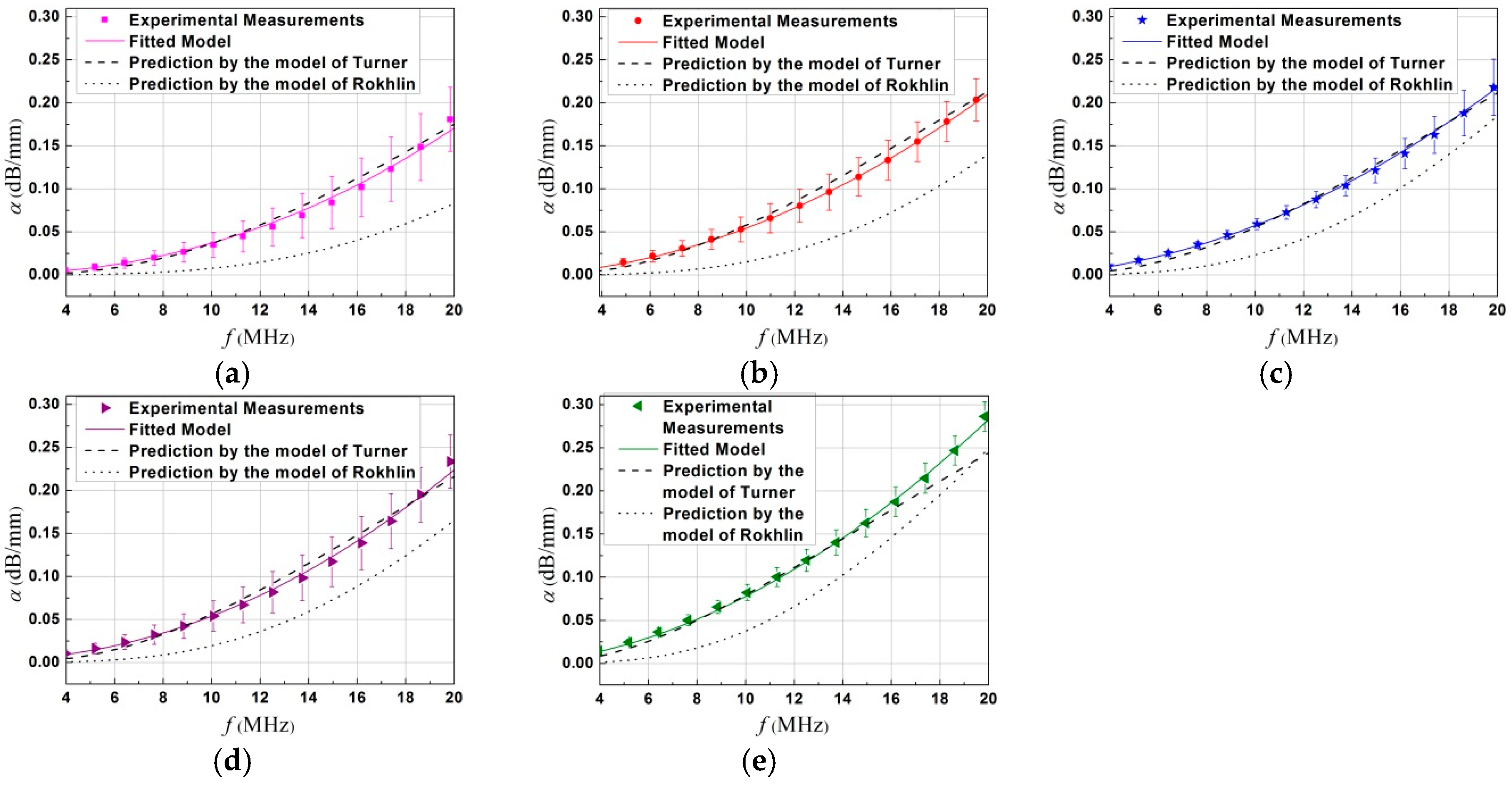
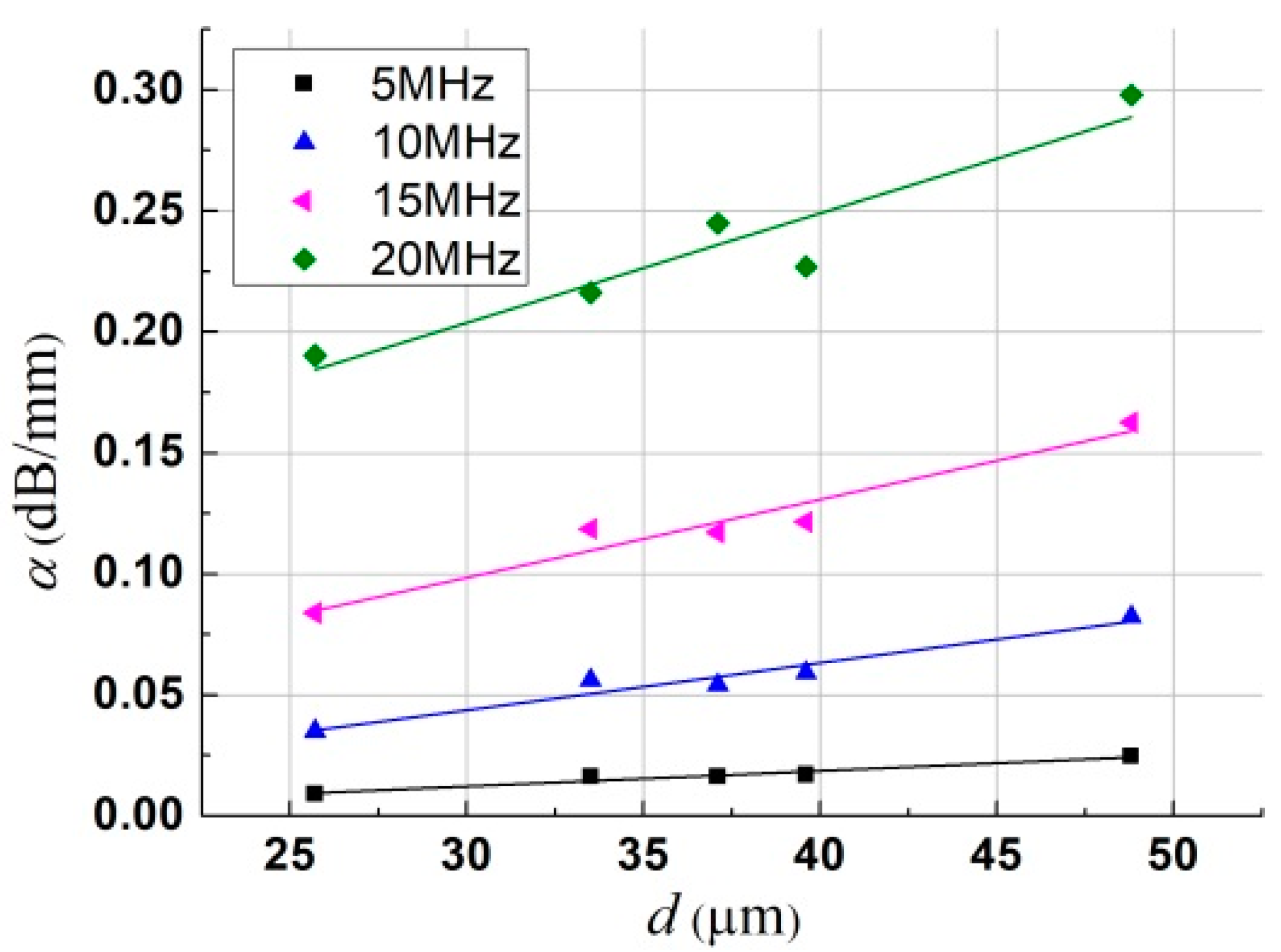
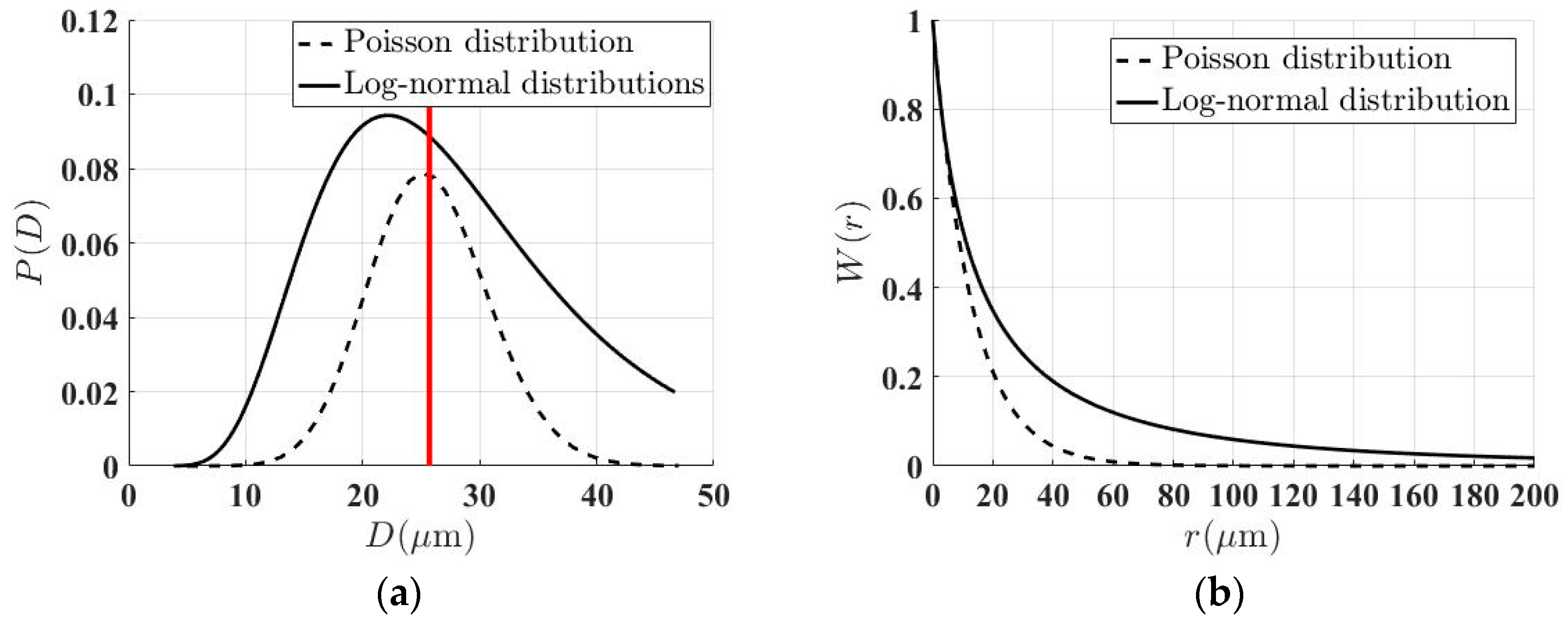

| Annealed Temperature/Holding Time | Data Obtained by OIM Analysis | Best-fit Log-normal Distributions | ||||||
|---|---|---|---|---|---|---|---|---|
| Fraction of α-phase | (μm) | Distribution Width | Grain Sampled | (μm) | RMSE (10−2) | |||
| 800 °C/0.5 h | 1.000 | 26 | 9.3 | 1668 | 0.42 | −10.24 | 29 | 1.44 |
| 800 °C/1.0 h | 0.999 | 33 | 12.5 | 1050 | 0.50 | −10.54 | 40 | 1.34 |
| 800 °C/2.0 h | 0.999 | 39 | 14 | 1154 | 0.46 | −10.27 | 39 | 1.51 |
| 800 °C/4.0 h | 0.992 | 37 | 12.3 | 1150 | 0.43 | −10.25 | 39 | 1.40 |
| 800 °C/8.0 h | 0.987 | 49 | 10.7 | 916 | 0.48 | −9.95 | 53 | 2.04 |
| Samples | (μm) | a (10−3 dB/mm) | b* (10−15 dB·Sn/mm) | n | R-square | RMSE (10−2 dB/mm) |
|---|---|---|---|---|---|---|
| 0.5 h | 26 | 1.60 | 0.015 | 2.20 | 0.9932 | 0.44 |
| 1.0 h | 33 | 0.59 | 2.38 | 1.90 | 0.9996 | 0.14 |
| 2.0 h | 39 | 1.08 | 1.86 | 1.93 | 0.9992 | 0.18 |
| 4.0 h | 37 | 1.53 | 0.13 | 2.09 | 0.9964 | 0.41 |
| 8.0 h | 49 | 1.59 | 7.07 | 1.86 | 0.9991 | 0.27 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bai, X.; Zhao, Y.; Ma, J.; Liu, Y.; Wang, Q. Grain-Size Distribution Effects on the Attenuation of Laser-Generated Ultrasound in α-Titanium Alloy. Materials 2019, 12, 102. https://doi.org/10.3390/ma12010102
Bai X, Zhao Y, Ma J, Liu Y, Wang Q. Grain-Size Distribution Effects on the Attenuation of Laser-Generated Ultrasound in α-Titanium Alloy. Materials. 2019; 12(1):102. https://doi.org/10.3390/ma12010102
Chicago/Turabian StyleBai, Xue, Yang Zhao, Jian Ma, Yunxi Liu, and Qiwu Wang. 2019. "Grain-Size Distribution Effects on the Attenuation of Laser-Generated Ultrasound in α-Titanium Alloy" Materials 12, no. 1: 102. https://doi.org/10.3390/ma12010102




