Simulation of Sloped-Bed Tuned Liquid Dampers Using a Nonlinear Shallow Water Model
Abstract
:1. Introduction
2. Governing Equations
3. Numerical Model
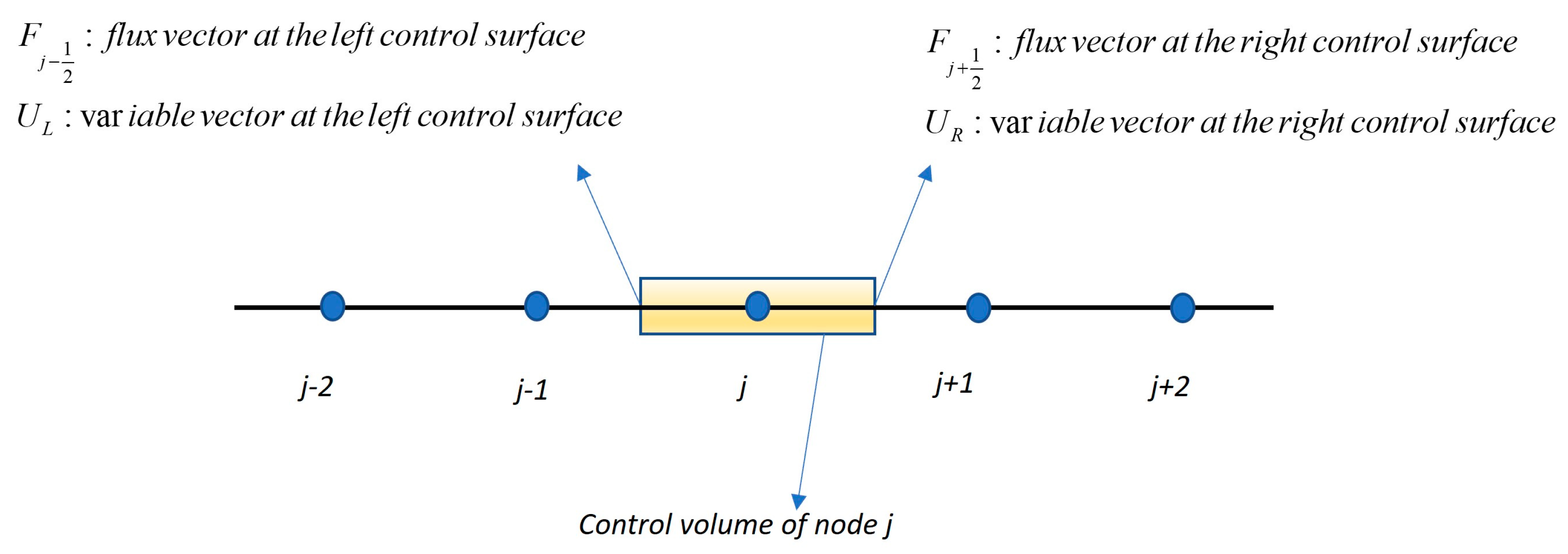
3.1. Flux Approximation
3.1.1. Roe Method
3.1.2. Central Upwind Method
3.2. Variable Vector Approximation
3.3. Source Terms
3.3.1. Explicit Terms
3.3.2. Implicit Term
3.4. Time Integration
3.5. Boundary Conditions and Courant Number
3.6. Dynamic Equation of SDOF
3.7. Coupling Schemes
4. Results
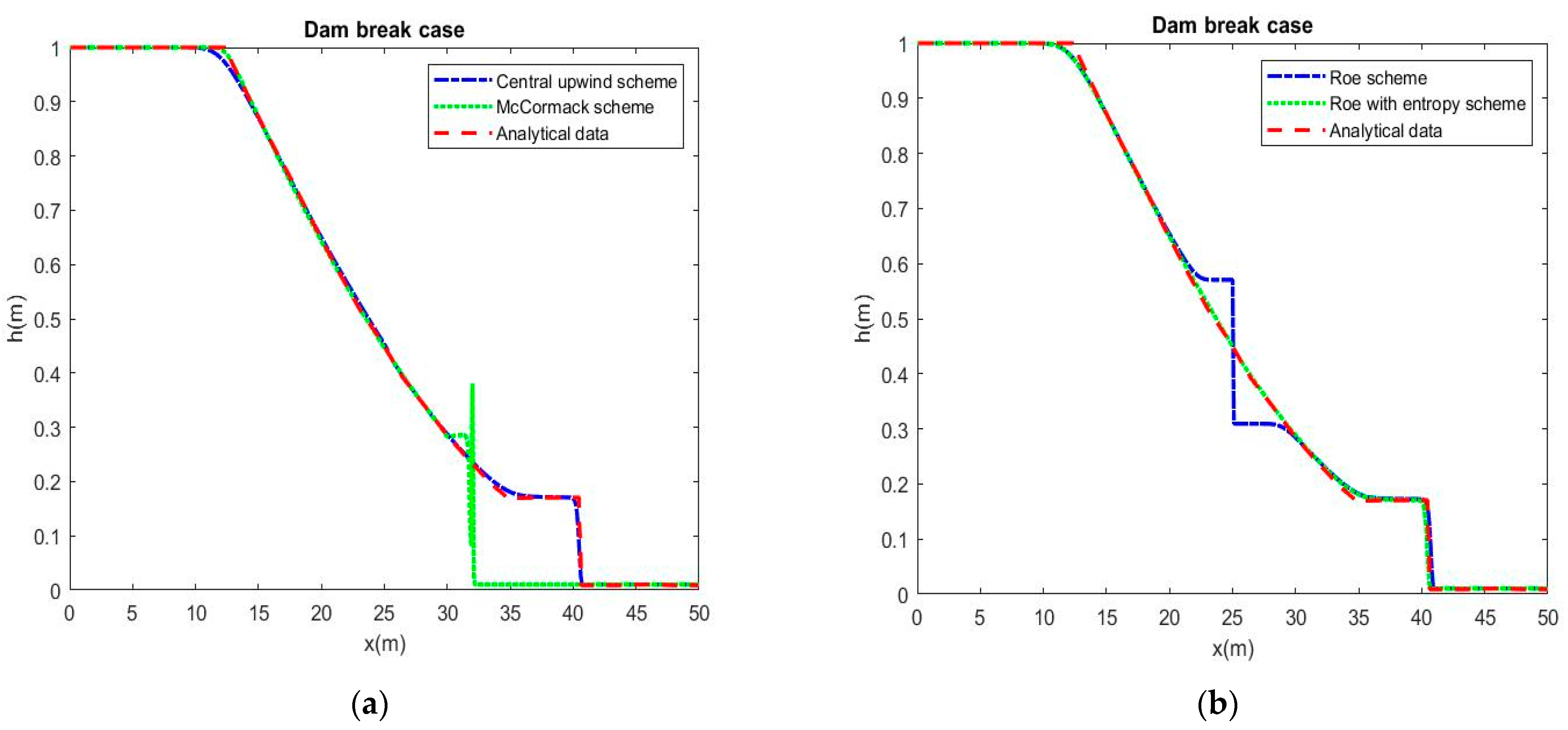
4.1. Test Case 1: Dam Break on the Dry Bed
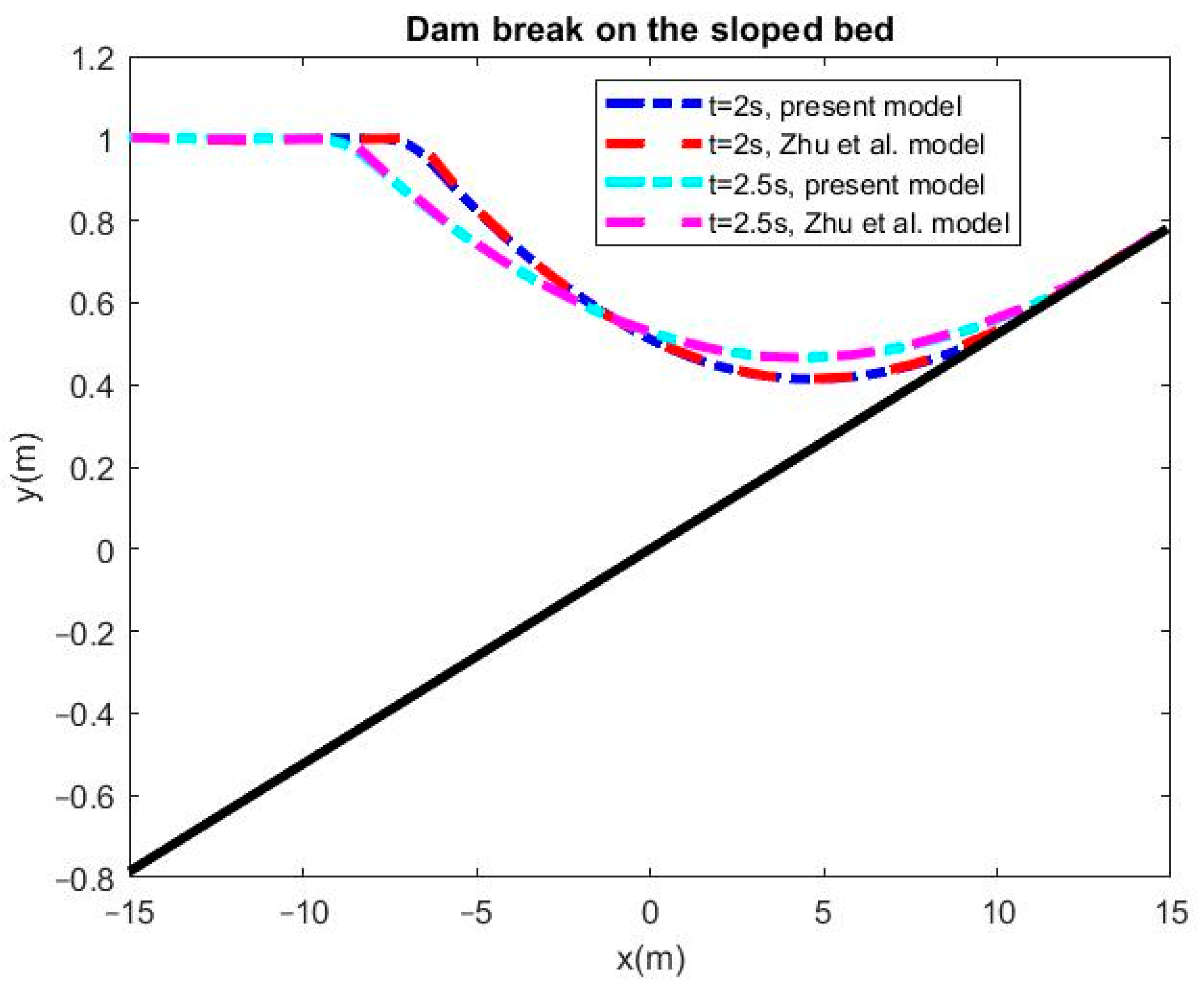
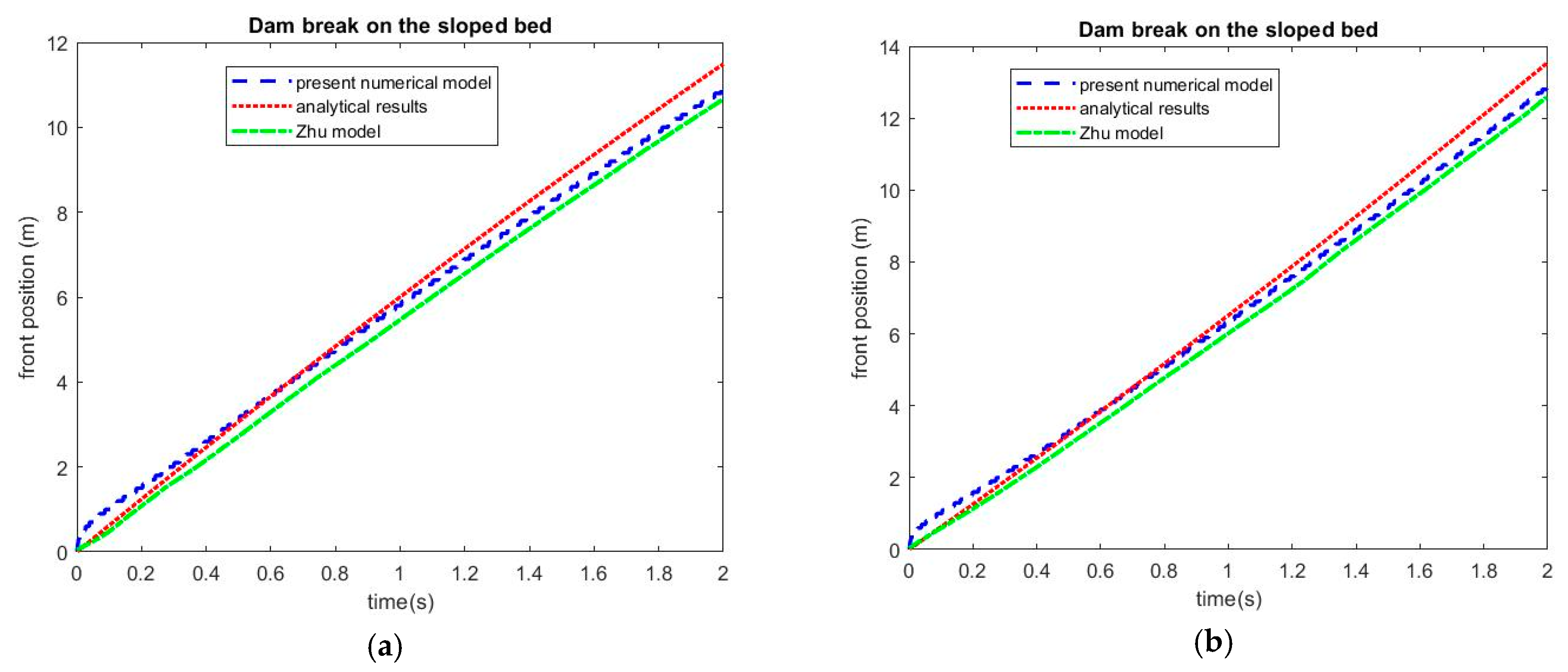
4.2. Test Case 2: Dam Break on the Sloped Bed
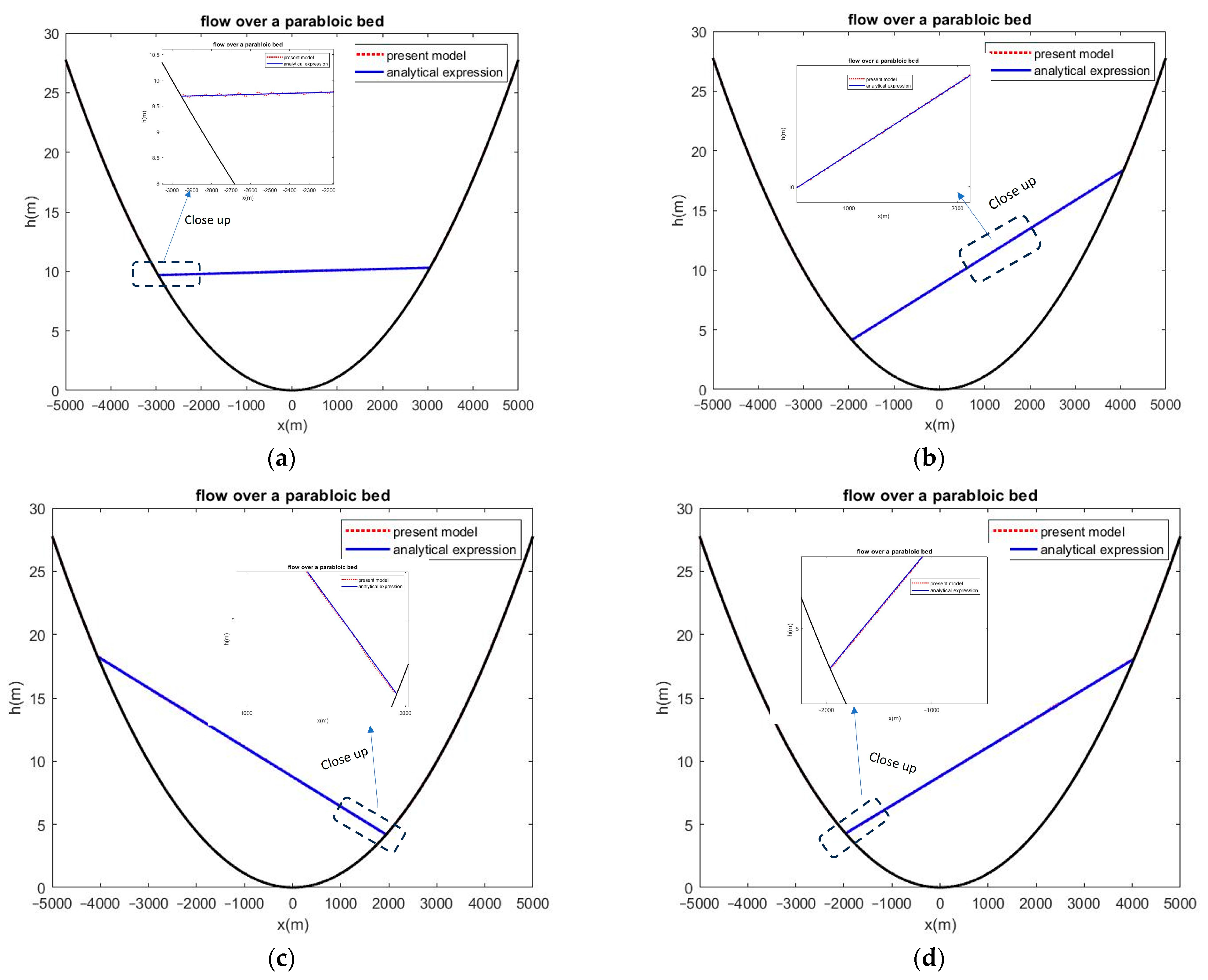
4.3. Test Case 3: Perturbated Flow over a Parabolic Topography
4.4. Test Case 4: Sloshing Simulation of the Box Tank
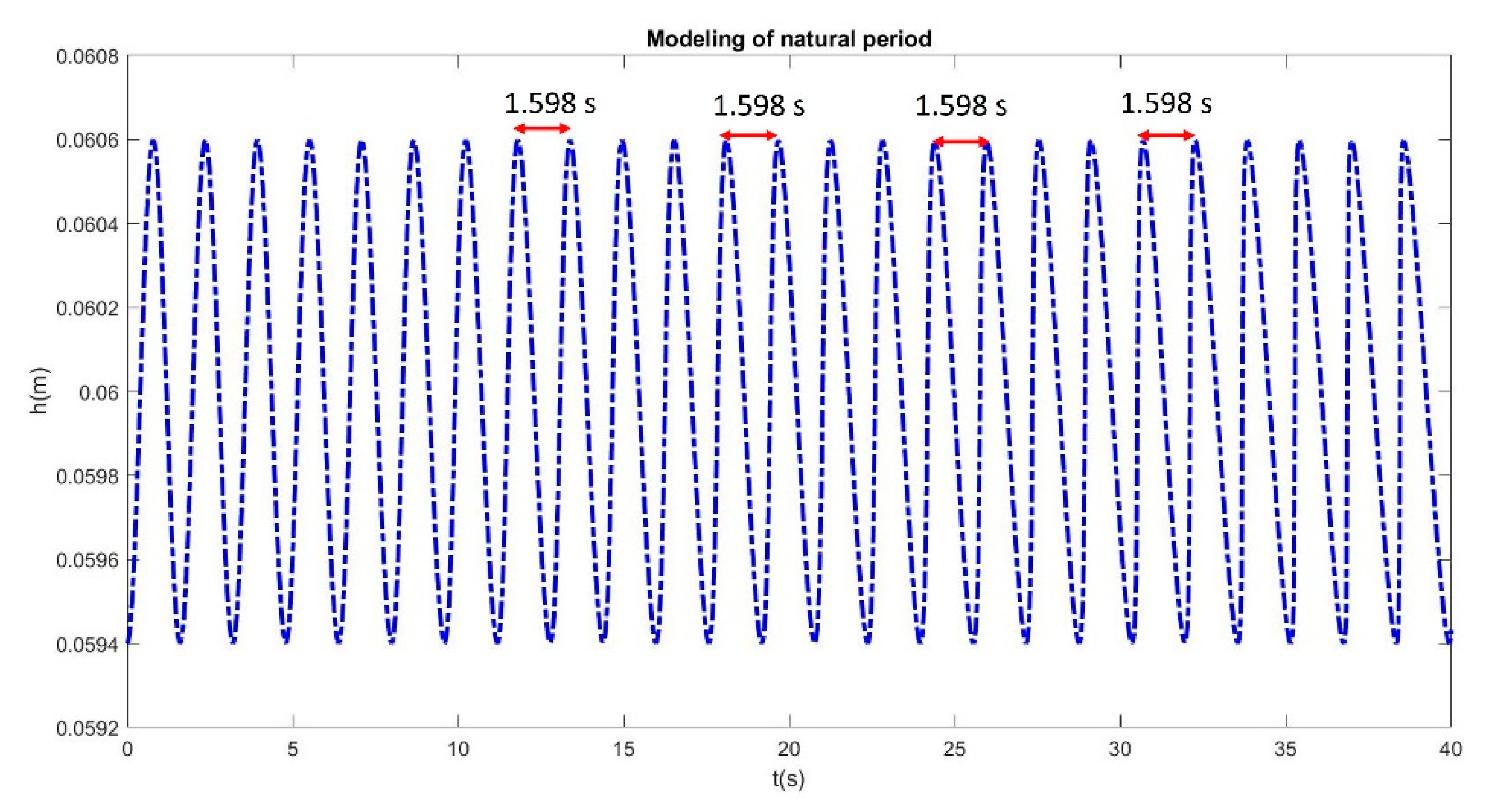
4.4.1. Natural Period Evaluation
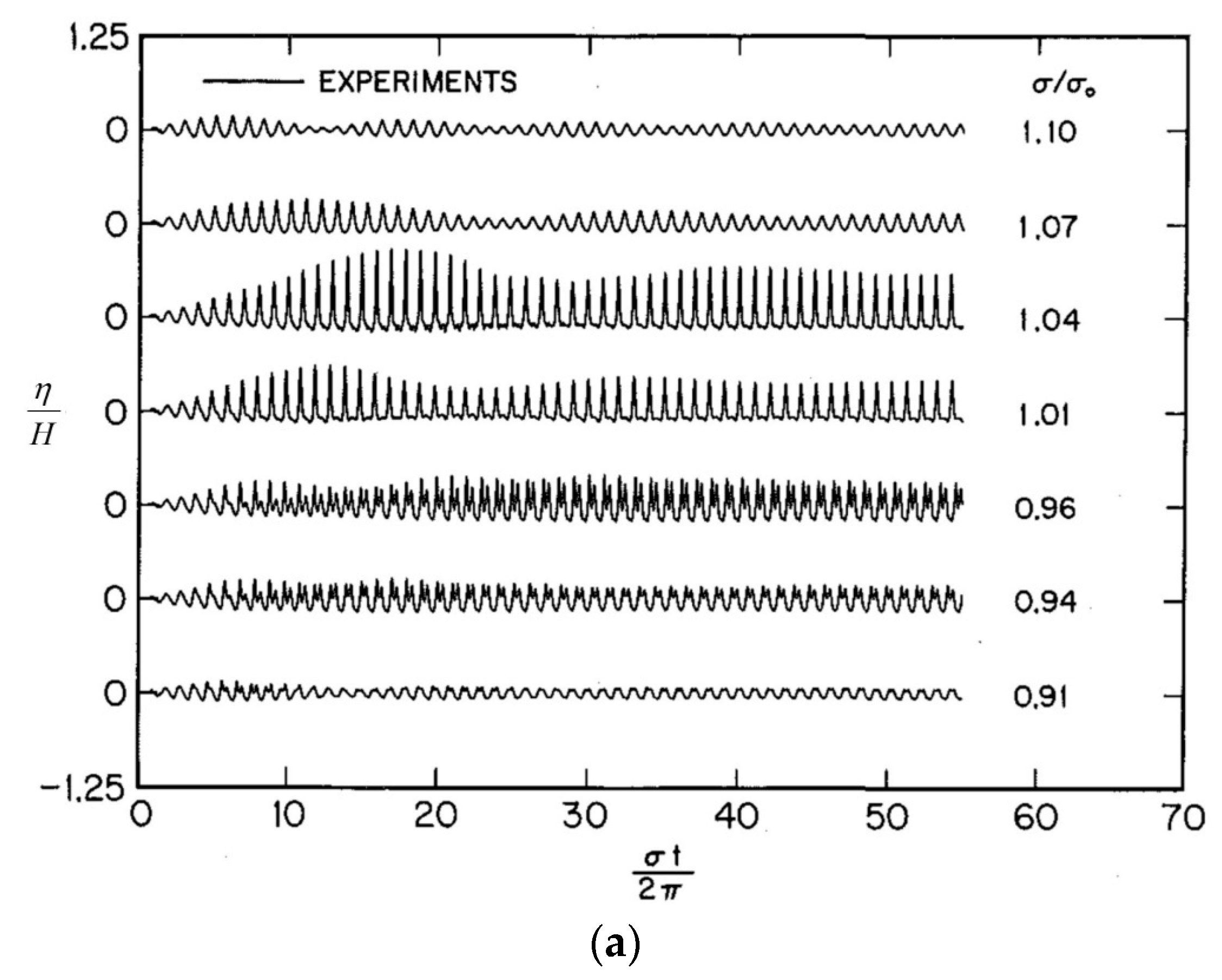
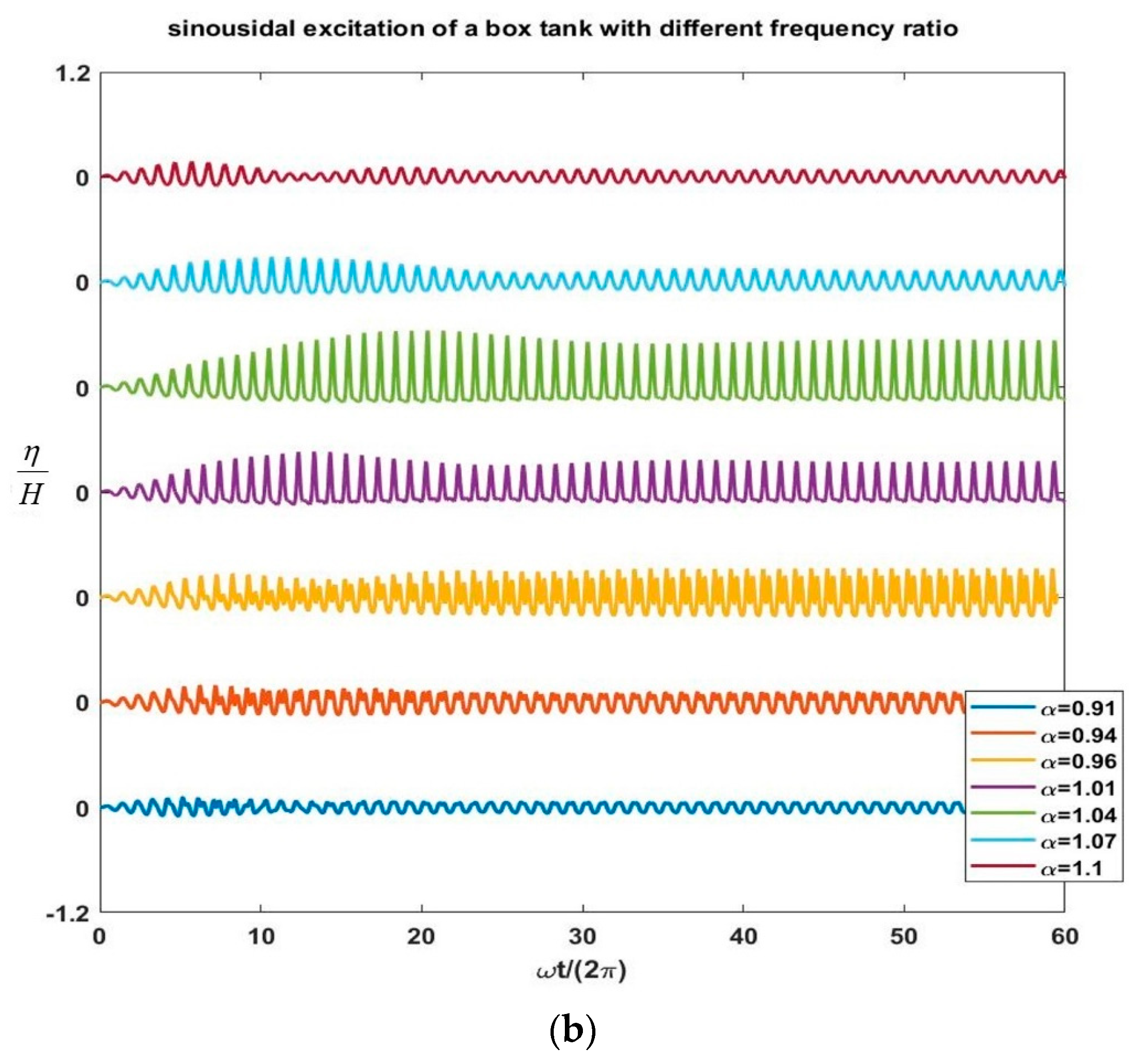
4.4.2. Harmonic Excitation of the Box Tank
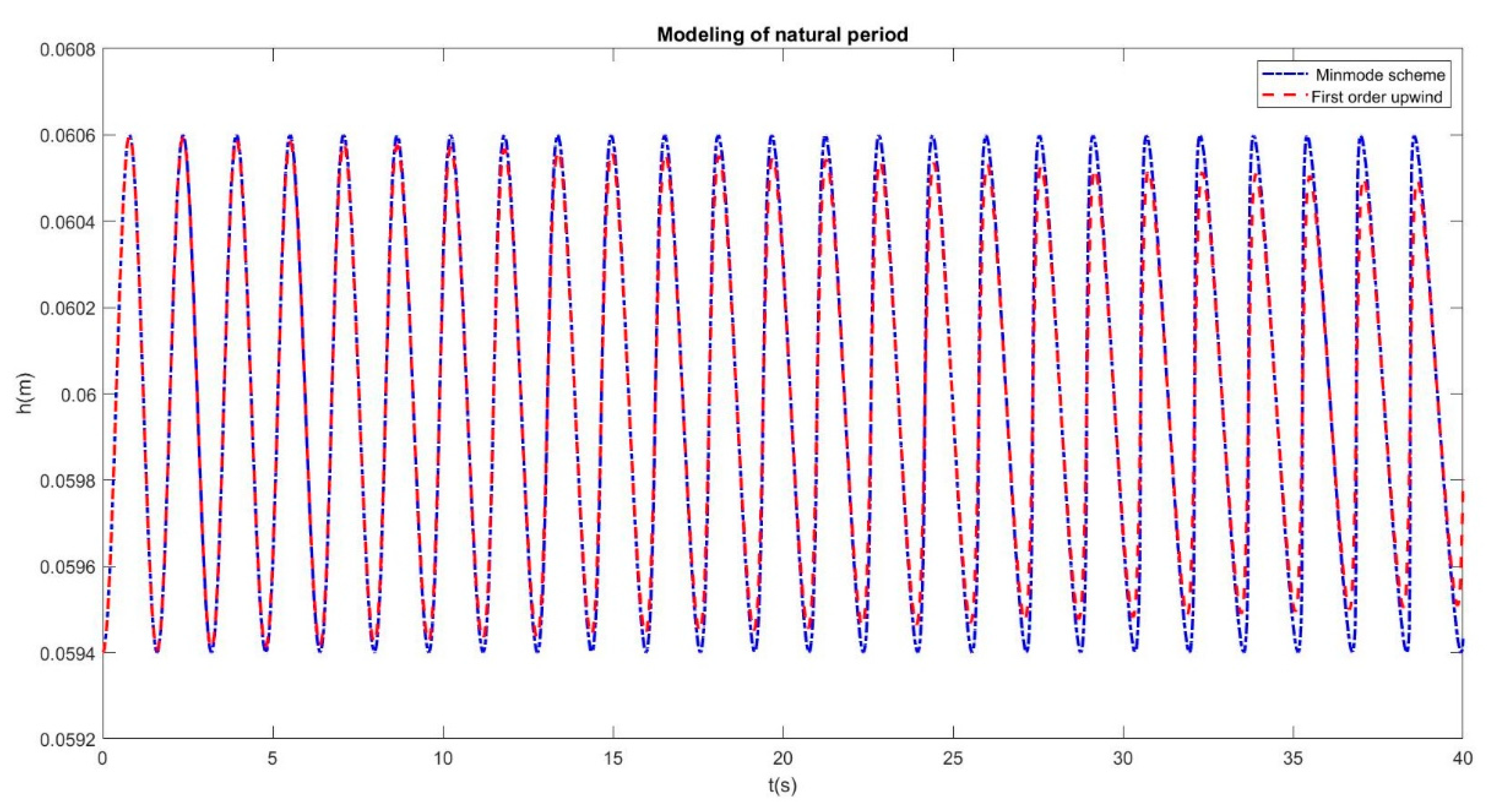
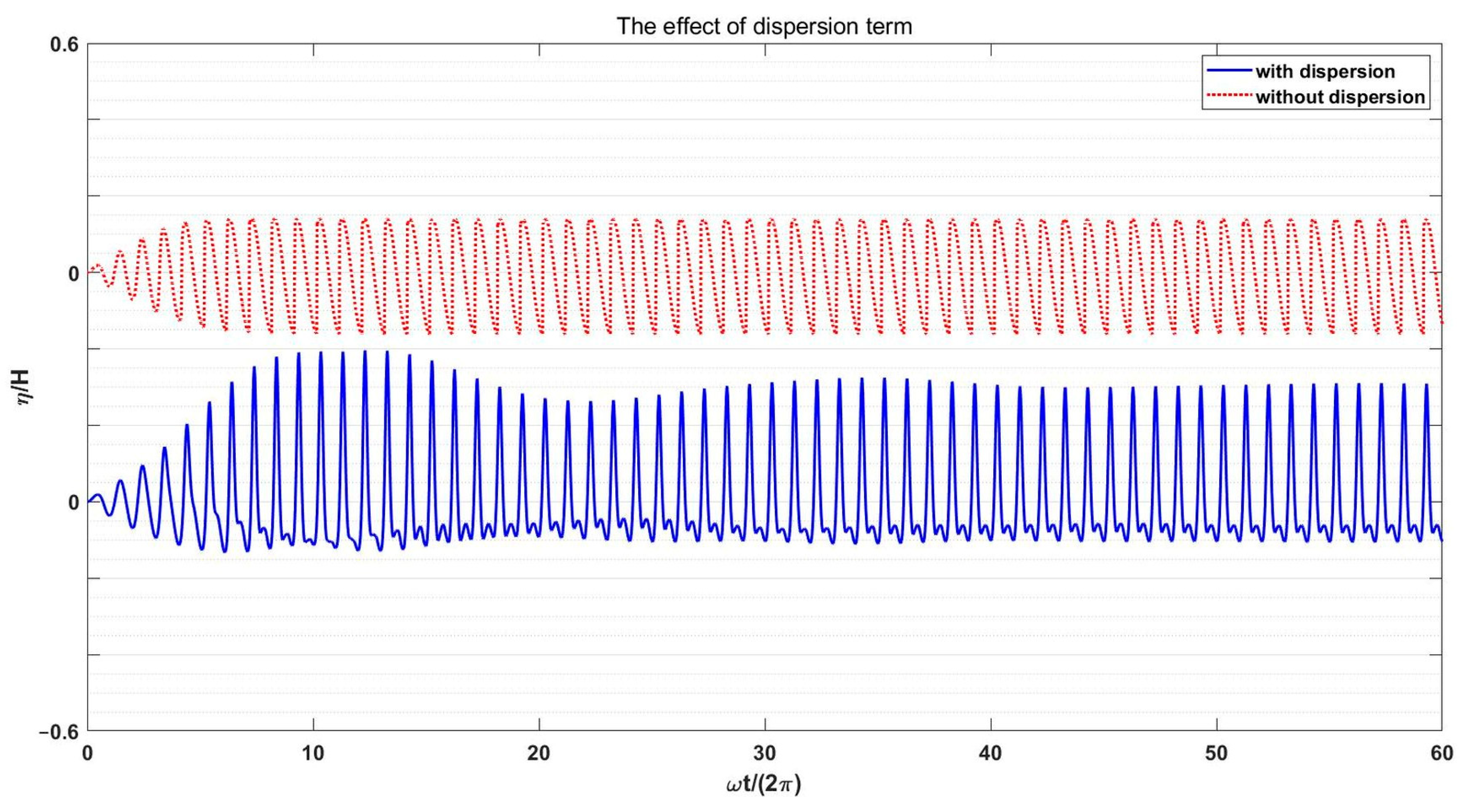
4.4.3. The Role of the Dispersion Term
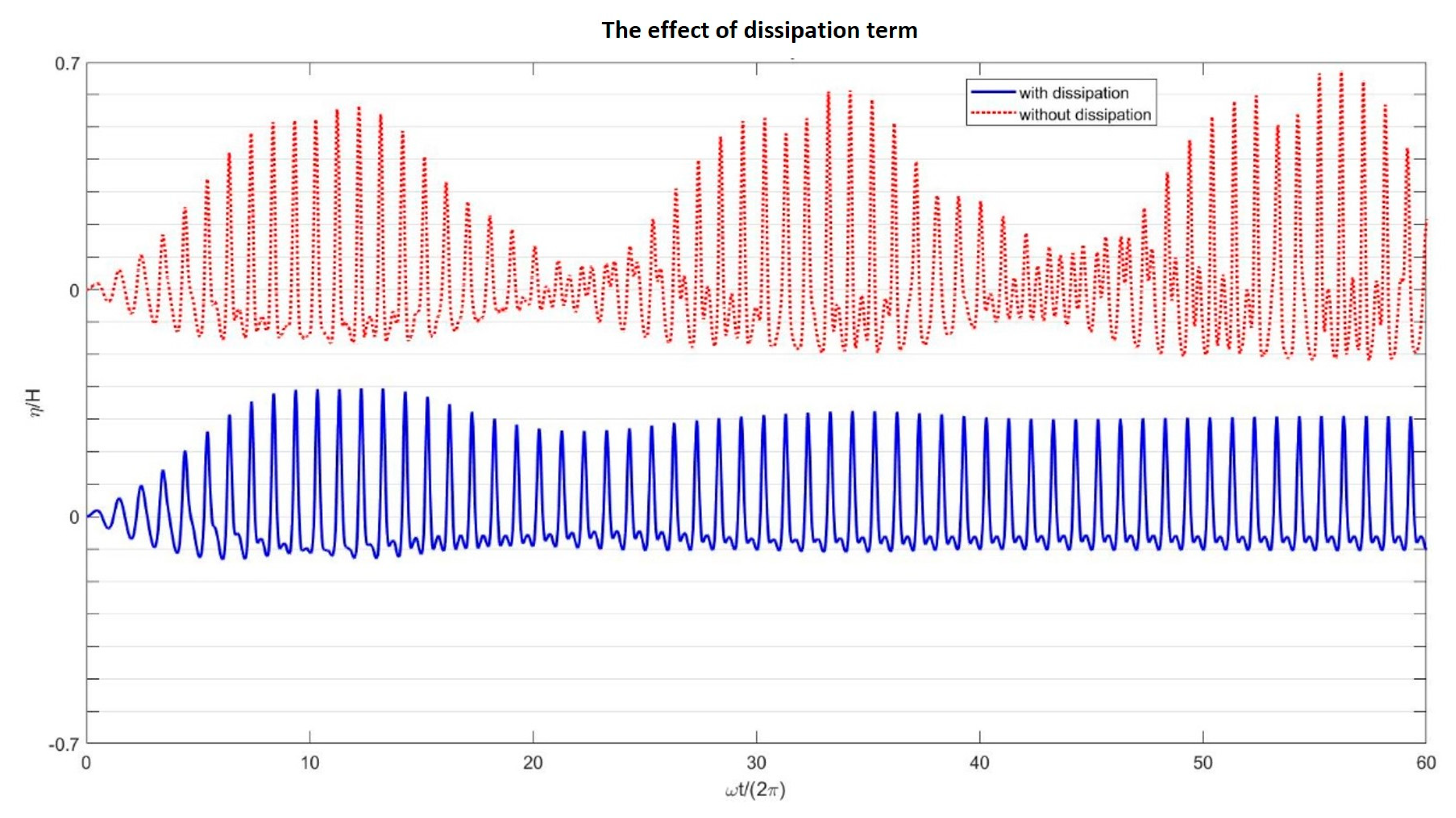
4.4.4. The Role of the Dissipation Term
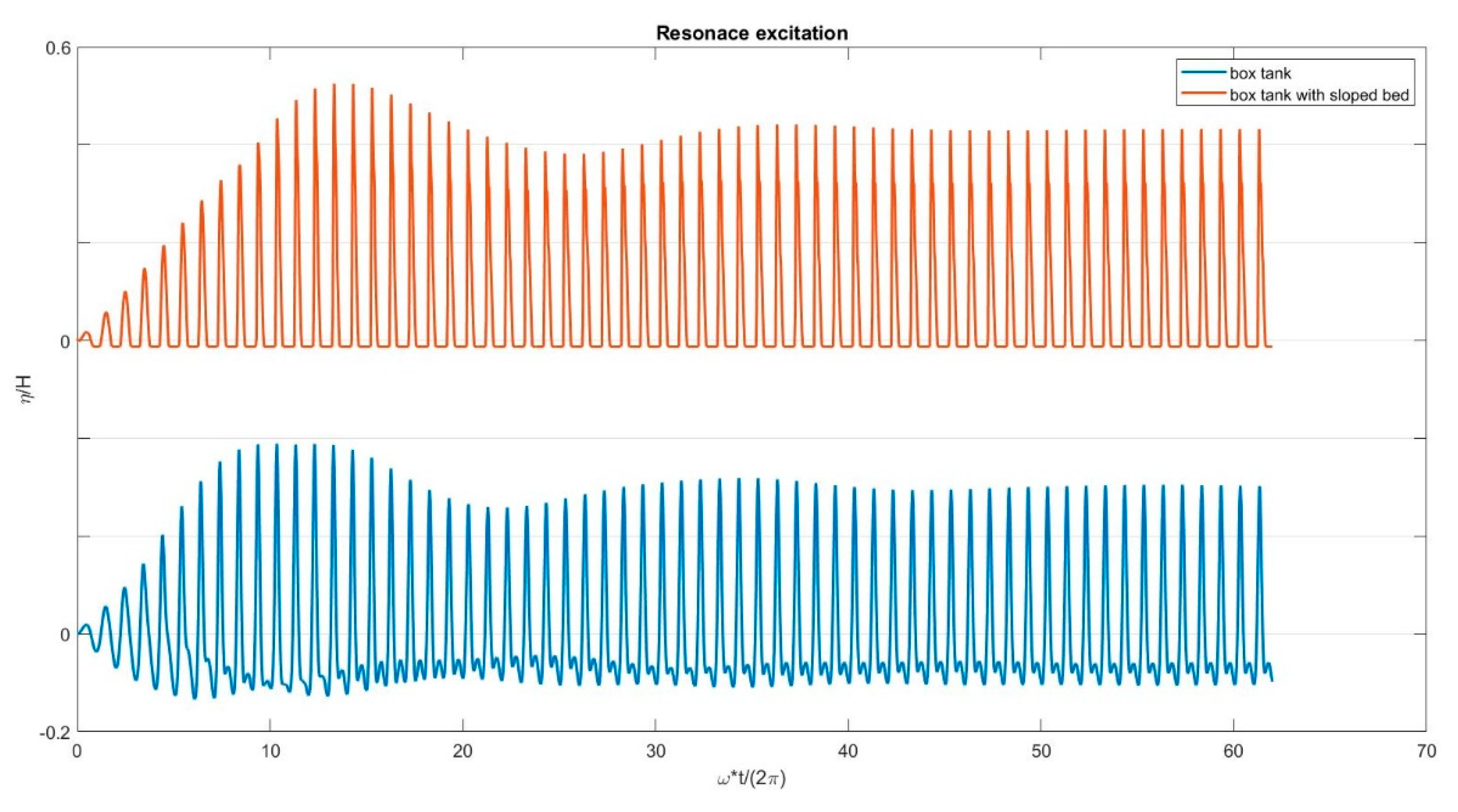
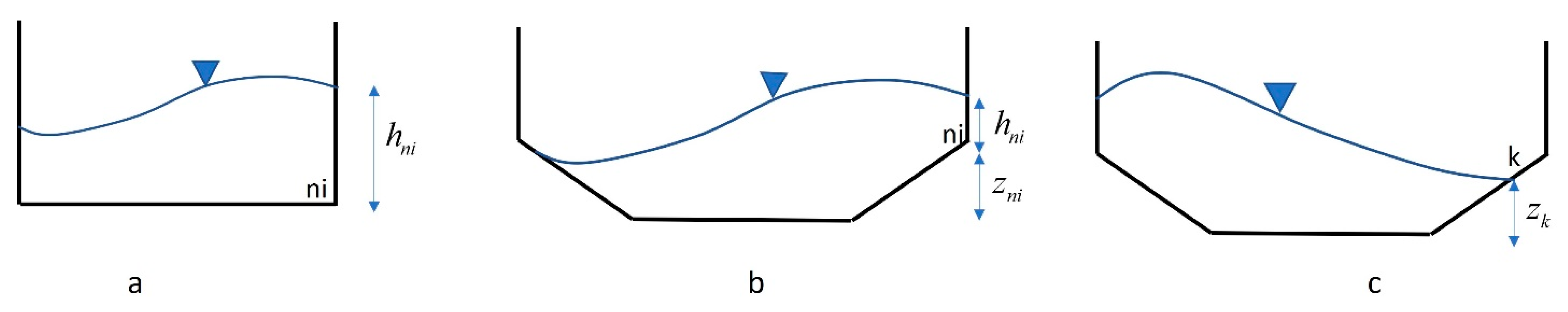
4.5. Test Case 5: Sloshing of Box Tank with Sloped Bed
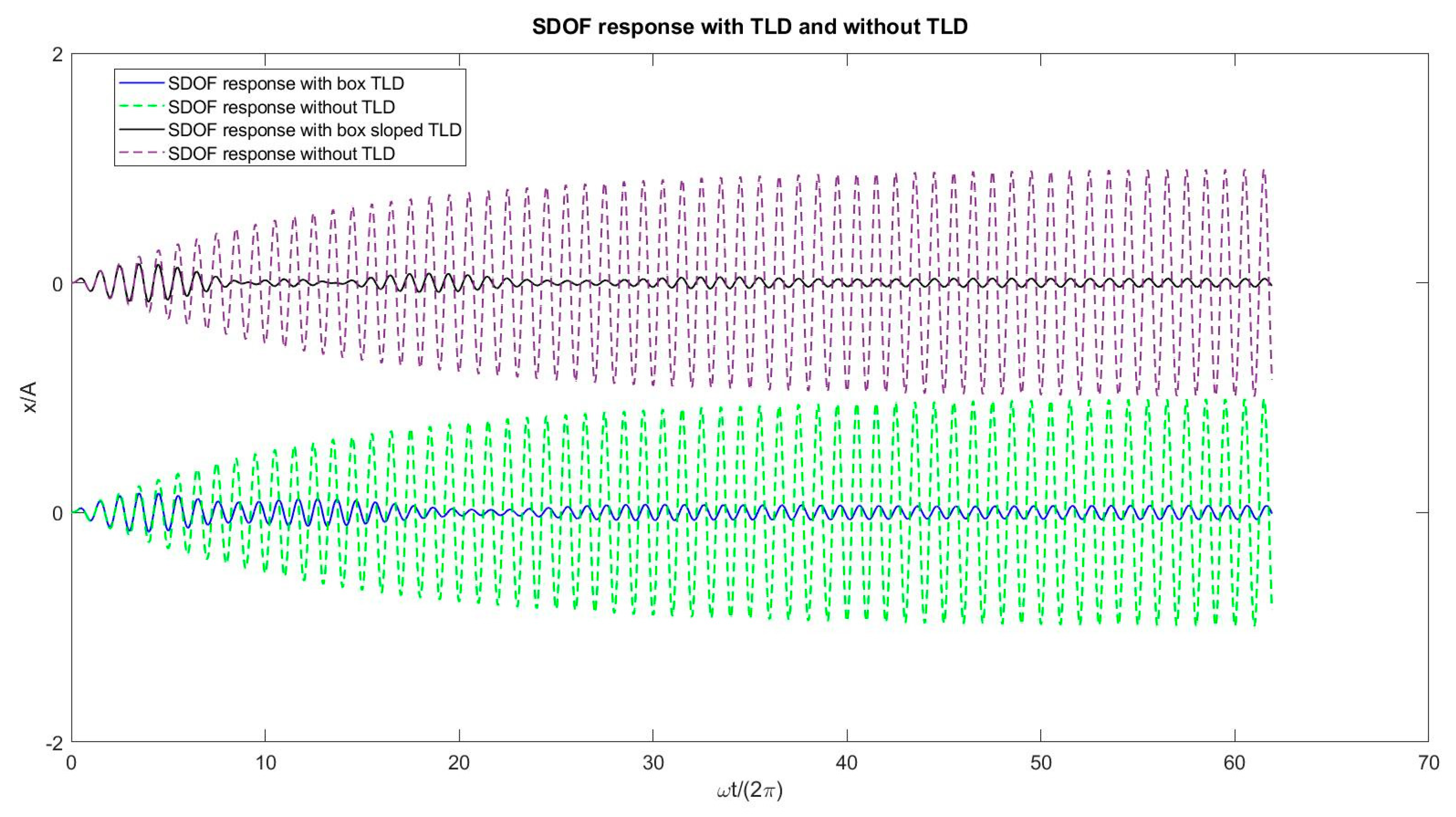
4.6. Test Case 6: TLD Simulation
5. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. MacCormack Scheme
References
- Toussi, I.B.; Kianoush, R.; Mohammadian, A. Numerical and Experimental Investigation of Rectangular Liquid-Containing Structures under Seismic Excitation. Infrastructures 2020, 6, 1. [Google Scholar] [CrossRef]
- Liu, D.; Lin, P. A numerical study of three-dimensional liquid sloshing in tanks. J. Comput. Phys. 2008, 227, 3921–3939. [Google Scholar] [CrossRef]
- Vîlceanu, V.; Kavrakov, I.; Morgenthal, G. Coupled numerical simulation of liquid sloshing dampers and wind–structure simulation model. J. Wind. Eng. Ind. Aerodyn. 2023, 240, 105505. [Google Scholar] [CrossRef]
- Hirt, C.W.; Nichols, B.D. Volume of fluid (VOF) method for the dynamics of free boundaries. J. Comput. Phys. 1981, 39, 201–225. [Google Scholar] [CrossRef]
- Veletsos, A.S.; Tang, Y.; Tang, H.T. Dynamic Response of Flexibly Supported Liquid-Storage Tanks. J. Struct. Eng. 1992, 118, 264–283. [Google Scholar] [CrossRef]
- Ghaemmaghami, A.R.; Kianoush, M.R. Effect of Wall Flexibility on Dynamic Response of Concrete Rectangular Liquid Storage Tanks under Horizontal and Vertical Ground Motions. J. Struct. Eng. 2010, 136, 441–451. [Google Scholar] [CrossRef]
- Panigrahy, P.; Saha, U.; Maity, D. Experimental studies on sloshing behavior due to horizontal movement of liquids in baffled tanks. Ocean Eng. 2009, 36, 213–222. [Google Scholar] [CrossRef]
- Virella, J.C.; Suárez, L.E.; Godoy, L.A. A Static Nonlinear Procedure for the Evaluation of the Elastic Buckling of Anchored Steel Tanks Due to Earthquakes. J. Earthq. Eng. 2008, 12, 999–1022. [Google Scholar] [CrossRef]
- Sobhan, M.; Rofooei, F.; Attari, N.K. Buckling behavior of the anchored steel tanks under horizontal and vertical ground motions using static pushover and incremental dynamic analyses. Thin-Walled Struct. 2017, 112, 173–183. [Google Scholar] [CrossRef]
- Hashemi, S.; Kianoush, R.; Khoubani, M. A mechanical model for soil-rectangular tank interaction effects under seismic loading. Soil Dyn. Earthq. Eng. 2021, 153, 107092. [Google Scholar] [CrossRef]
- Sriram, V.; Sannasiraj, S.A.; Sundar, V. Numerical simulation of 2D sloshing waves due to horizontal and vertical random excitation. Applied Ocean Research 2006, 28, 19–32. [Google Scholar] [CrossRef]
- Ikeda, T.; Ibrahim, R.A.; Harata, Y.; Kuriyama, T. Nonlinear liquid sloshing in a square tank subjected to obliquely horizontal excitation. J. Fluid Mech. 2012, 700, 304–328. [Google Scholar] [CrossRef]
- Antuono, M.; Bouscasse, B.; Colagrossi, A.; Lugni, C. Two-dimensional modal method for shallow-water sloshing in rectangular basins. J. Fluid Mech. 2012, 700, 419–440. [Google Scholar] [CrossRef]
- Saburin, D.S. Tank sloshing simulations in shallow-water approximation. In MARINE VI: Proceedings of the VI International Conference on Computational Methods in Marine Engineering; CIMNE: Barcelona, Spain, 2015; pp. 1039–1050. [Google Scholar]
- Sun, L.M.; Fujino, Y.; Pacheco, B.M.; Chaiseri, P. Modelling of tuned liquid damper (TLD). J. Wind. Eng. Ind. Aerodyn. 1992, 43, 1883–1894. [Google Scholar] [CrossRef]
- Koh, C.G.; Mahatma, S.; Wang, C.M. Theoretical and experimental studies on rectangular liquid dampers under arbitrary excitations. Earthq. Eng. Struct. Dyn. 1994, 23, 17–31. [Google Scholar] [CrossRef]
- Tait, M.; El Damatty, A.; Isyumov, N.; Siddique, M. Numerical flow models to simulate tuned liquid dampers (TLD) with slat screens. J. Fluids Struct. 2005, 20, 1007–1023. [Google Scholar] [CrossRef]
- Murudi, M.; Banerji, P. Effective control of earthquake response using tuned liquid dampers. J. Earthq. Technol. 2012, 49, 53–71. [Google Scholar]
- Banerji, P.; Samanta, A.; Chavan, S.A. Earthquake vibration control of structures using tuned liquid dampers: Experimental studies. Int. J. Adv. Struct. Eng. 2010, 2, 133–152. [Google Scholar]
- Konar, T.; Ghosh, A.D. Flow Damping Devices in Tuned Liquid Damper for Structural Vibration Control: A Review. Arch. Comput. Methods Eng. 2020, 28, 2195–2207. [Google Scholar] [CrossRef]
- Abd-Elhamed, A.; Tolan, M. Tuned liquid damper for vibration mitigation of seismic-excited structures on soft soil. Alex. Eng. J. 2022, 61, 9583–9599. [Google Scholar] [CrossRef]
- Pandit, A.R.; Biswal, K.C. Seismic Control of Structures Using Sloped Bottom Tuned Liquid Damper. Int. J. Struct. Stab. Dyn. 2019, 19, 1950096. [Google Scholar] [CrossRef]
- Chaiviriyawong, P.; Webster, W.; Pinkaew, T.; Lukkunaprasit, P. Simulation of characteristics of tuned liquid column damper using a potential-flow method. Eng. Struct. 2007, 29, 132–144. [Google Scholar] [CrossRef]
- Banerji, P.; Murudi, M.; Shah, A.H.; Popplewell, N. Tuned liquid dampers for controlling earthquake response of structures. Earthq. Eng. Struct. Dyn. 2000, 29, 587–602. [Google Scholar] [CrossRef]
- Fujino, Y.; Sun, L.; Pacheco, B.M.; Chaiseri, P. Tuned Liquid Damper (TLD) for Suppressing Horizontal Motion of Structures. J. Eng. Mech. 1992, 118, 2017–2030. [Google Scholar] [CrossRef]
- Marivani, M.; Hamed, M. Evaluate pressure drop of slat screen in an oscillating fluid in a tuned liquid damper. Comput. Fluids 2017, 156, 384–401. [Google Scholar] [CrossRef]
- Zhao, Z.; Zhang, R.; Jiang, Y.; Pan, C. A tuned liquid inerter system for vibration control. Int. J. Mech. Sci. 2019, 164, 105171. [Google Scholar] [CrossRef]
- Wang, Q.; Tiwari, N.D.; Qiao, H.; Wang, Q. Inerter-based tuned liquid column damper for seismic vibration control of a single-degree-of-freedom structure. Int. J. Mech. Sci. 2020, 184, 105840. [Google Scholar] [CrossRef]
- Ashasi-Sorkhabi, A.; Malekghasemi, H.; Ghaemmaghami, A.; Mercan, O. Experimental investigations of tuned liquid damper-structure interactions in resonance considering multiple parameters. J. Sound Vib. 2017, 388, 141–153. [Google Scholar] [CrossRef]
- Saha, S.; Debbarma, R. An experimental study on response control of structures using multiple tuned liquid dampers under dynamic loading. Int. J. Adv. Struct. Eng. 2016, 9, 27–35. [Google Scholar] [CrossRef]
- Hu, X.; Zhao, Z.; Weng, D.; Hu, D. Design of a pair of isolated tuned liquid dampers (ITLDs) and application in multi-degree-of-freedom structures. Int. J. Mech. Sci. 2022, 217, 107027. [Google Scholar] [CrossRef]
- Bhattacharjee, E.; Halder, L.; Sharma, R.P. An experimental study on tuned liquid damper for mitigation of structural response. Int. J. Adv. Struct. Eng. 2013, 5, 3. [Google Scholar] [CrossRef]
- Samanta, A.; Banerji, P. Structural vibration control using modified tuned liquid dampers. IES J. Part A Civ. Struct. Eng. 2010, 3, 14–27. [Google Scholar] [CrossRef]
- Chang, Y.; Noormohamed, A.; Mercan, O. Analytical and experimental investigations of Modified Tuned Liquid Dampers (MTLDs). J. Sound Vib. 2018, 428, 179–194. [Google Scholar] [CrossRef]
- Ocak, A.; Bekdaş, G.; Nigdeli, S.M.; Kim, S.; Geem, Z.W. Optimization of Tuned Liquid Damper Including Different Liquids for Lateral Displacement Control of Single and Multi-Story Structures. Buildings 2022, 12, 377. [Google Scholar] [CrossRef]
- Pandit, A.; Biswal, K. Seismic control of multi degree of freedom structure outfitted with sloped bottom tuned liquid damper. Structures 2020, 25, 229–240. [Google Scholar] [CrossRef]
- Gardarsson, S.; Yeh, H.; Reed, D. Behavior of Sloped-Bottom Tuned Liquid Dampers. J. Eng. Mech. 2001, 127, 266–271. [Google Scholar] [CrossRef]
- Toro, E.F. Shock-Capturing Methods for Free-Surface Shallow Flows; Wiley and Sons Ltd.: Hoboken, NJ, USA, 2001; pp. 1–309. [Google Scholar]
- Benkhaldoun, F.; Seaïd, M. A simple finite volume method for the shallow water equations. J. Comput. Appl. Math. 2010, 234, 58–72. [Google Scholar] [CrossRef]
- Ardakani, H.A.; Bridges, T. Shallow-water sloshing in vessels undergoing prescribed rigid-body motion in two dimensions. Eur. J. Mech.-B/Fluids 2012, 31, 30–43. [Google Scholar] [CrossRef]
- Mohammadian, A.; Le Roux, D.Y. Simulation of shallow flows over variable topographies using unstructured grids. Int. J. Numer. Methods Fluids 2006, 52, 473–498. [Google Scholar] [CrossRef]
- Kurganov, A.; Levy, D. Central-upwind schemes for the Saint-Venant system. ESAIM Math. Model. Numer. Anal. 2002, 36, 397–425. [Google Scholar] [CrossRef]
- Mohammadian, A.; Le Roux, D.; Tajrishi, M. A conservative extension of the method of characteristics for 1-D shallow flows. Appl. Math. Model. 2007, 31, 332–348. [Google Scholar] [CrossRef]
- Durran, D.R. Numerical Methods for Fluid Dynamics: With Applications to Geophysics; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2010; Volume 32. [Google Scholar]
- Humar, J. Dynamics of Structures; CRC Press: Boca Raton, FL, USA, 2012. [Google Scholar]
- Chopra, A.K. Dynamics of Structures; Pearson Education India: Chennai, India, 2007. [Google Scholar]
- Liao, K.; Hu, C. A coupled FDM–FEM method for free surface flow interaction with thin elastic plate. J. Mar. Sci. Technol. 2012, 18, 1–11. [Google Scholar] [CrossRef]
- MacCormack, R.W. Numerical solution of the interaction of a shock wave with a laminar boundary layer. In Proceedings of the Second International Conference on Numerical Methods in Fluid Dynamics, University of California, Berkeley, CA, USA, 15–19 September 1970; Springer: Berlin/Heidelberg, Germany, 2005; pp. 151–163. [Google Scholar]
- Chaudhry, M.H.; Hussaini, M.Y. Second-order accurate explicit finite-difference schemes for waterhammer analysis. J. Fluids Eng. 1985, 107, 523–529. [Google Scholar] [CrossRef]
- Amara, L.; Berreksi, A.; Achour, B. Adapted MacCormack Finite-Differences Scheme for Water Hammer Simulation. J. Civ. Eng. Sci. 2013, 2, 226–233. [Google Scholar]
- Fennema, R.J.; Chaudhry, M.H. Explicit numerical schemes for unsteady free-surface flows with shocks. Water Resour. Res. 1986, 22, 1923–1930. [Google Scholar] [CrossRef]
- Garcia, R.; Kahawita, R.A. Numerical solution of the St. Venant equations with the MacCormack finite-difference scheme. Int. J. Numer. Methods Fluids 1986, 6, 259–274. [Google Scholar] [CrossRef]
- van Leer, B.; Lee, W.T.; Powell, K.G. Sonic point capturing. In Proceedings of the 9th CFD Conference, Buffalo, NY, USA, 13–15 June 1989. [Google Scholar]
- Bradford, S.F.; Sandres, B.F. Finite-volume model for shallow-water fooding of arbitrary topography. J. Hydraul. Eng. (ASCE) 2002, 128, 289–298. [Google Scholar] [CrossRef]
- Xing, Y.; Zhang, X.; Shu, C.-W. Positivity-preserving high order well-balanced discontinuous Galerkin methods for the shallow water equations. Adv. Water Resour. 2010, 33, 1476–1493. [Google Scholar] [CrossRef]
- Zhu, Z.; Yang, Z.; Bai, F.; An, R. A New Well-Balanced Reconstruction Technique for the Numerical Simulation of Shallow Water Flows with Wet/Dry Fronts and Complex Topography. Water 2018, 10, 1661. [Google Scholar] [CrossRef]
- Sampson, J.; Easton, A.; Singh, M. Moving boundary shallow water flow above parabolic bottom topography. Anziam J. 2006, 47, 373–387. [Google Scholar] [CrossRef]
- Lepelletier, T.G.; Raichlen, F. Nonlinear Oscillations in Rectangular Tanks. J. Eng. Mech. 1988, 114, 1–23. [Google Scholar] [CrossRef]
- Wu, G.; Ma, Q.; Taylor, R.E. Numerical simulation of sloshing waves in a 3D tank based on a finite element method. Appl. Ocean Res. 1998, 20, 337–355. [Google Scholar] [CrossRef]
- Cao, X.; Ming, F.; Zhang, A. Sloshing in a rectangular tank based on SPH simulation. Appl. Ocean Res. 2014, 47, 241–254. [Google Scholar] [CrossRef]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khanpour, M.; Mohammadian, A.; Shirkhani, H.; Kianoush, R. Simulation of Sloped-Bed Tuned Liquid Dampers Using a Nonlinear Shallow Water Model. Water 2024, 16, 1394. https://doi.org/10.3390/w16101394
Khanpour M, Mohammadian A, Shirkhani H, Kianoush R. Simulation of Sloped-Bed Tuned Liquid Dampers Using a Nonlinear Shallow Water Model. Water. 2024; 16(10):1394. https://doi.org/10.3390/w16101394
Chicago/Turabian StyleKhanpour, Mahdiyar, Abdolmajid Mohammadian, Hamidreza Shirkhani, and Reza Kianoush. 2024. "Simulation of Sloped-Bed Tuned Liquid Dampers Using a Nonlinear Shallow Water Model" Water 16, no. 10: 1394. https://doi.org/10.3390/w16101394




