Pseudo-Steady-State Parameters for a Well Penetrated by a Fracture with an Azimuth Angle in an Anisotropic Reservoir
Abstract
:1. Introduction
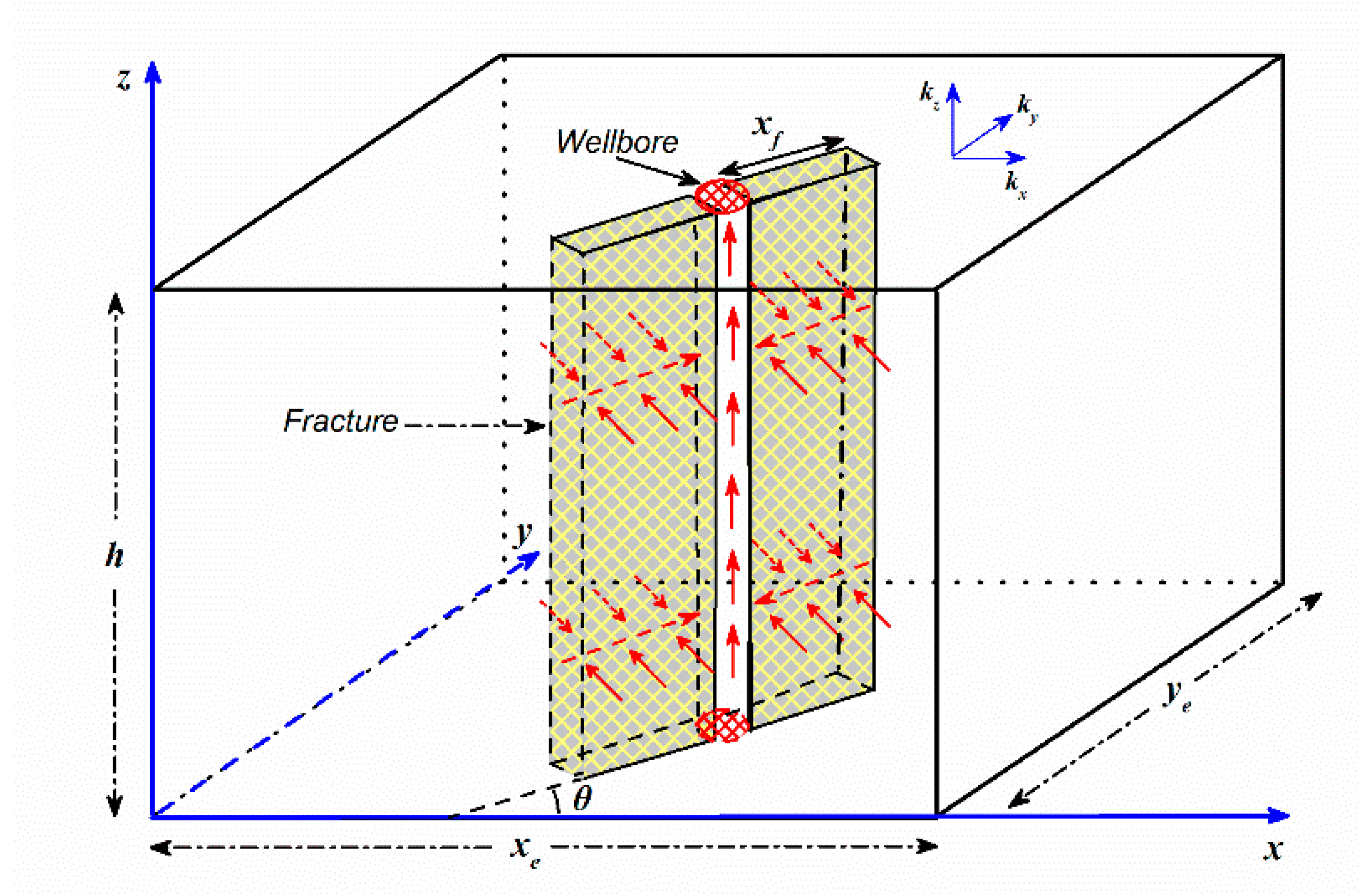
2. Physical Model
- (1)
- This rectangular reservoir is closed at every boundary, and it is anisotropic in the horizontal direction and its porous medium is homogeneous. It has a uniform thickness with constant porosity and its permeability in x and y direction are and , respectively.
- (2)
- Flow in this reservoir is considered to be a slightly compressible single-phase fluid with constant viscosity and total compressibility , and obeys Darcy’s law.
- (3)
- The reservoir is fully penetrated by a finite-conductivity vertical fracture, which lies at an arbitrary azimuth angle in the x-y plane ().
- (4)
- No fluid is assumed to flow at the fracture tip. The total flow contribution of the fracture to the wellbore is .
- (5)
- The half length of the fracture is assumed to have constant length , constant width and constant permeability .
- (6)
- Gravity effects are neglected and basic laminar flow occurs in the system.
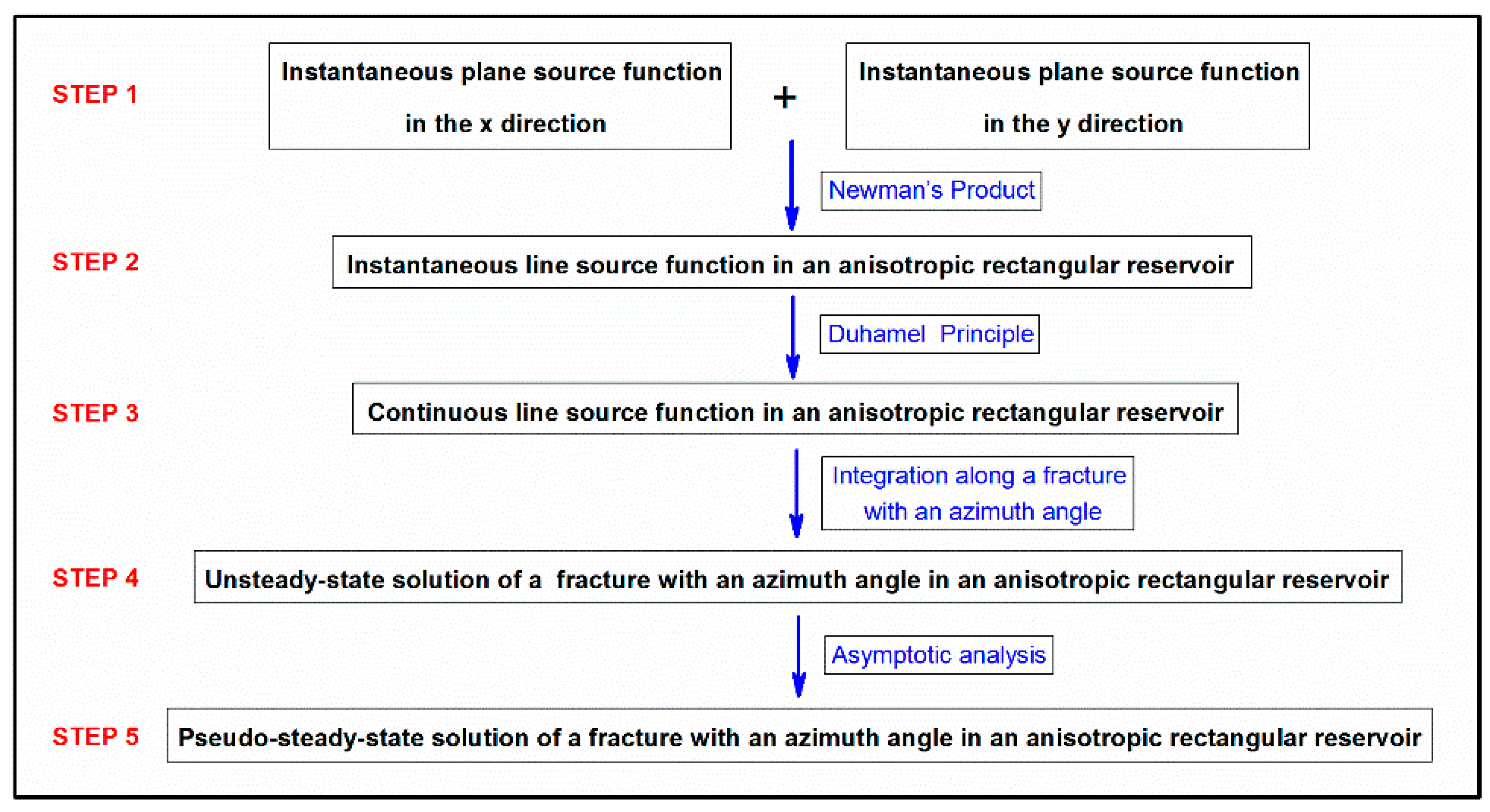
3. Mathematical Model
4. Model Validation
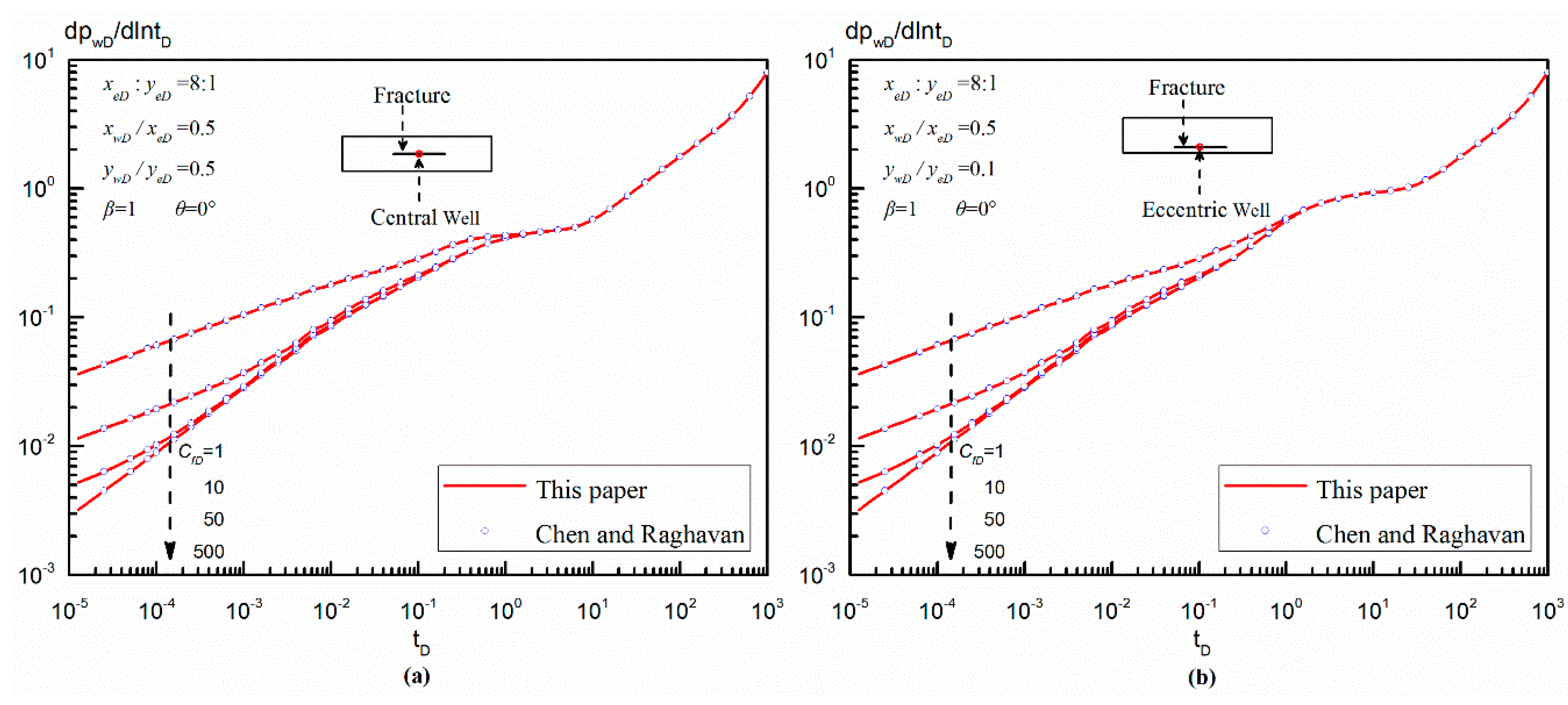
4.1. Validation of a Fractured Well in an Isotropic Rectangular Reservoir
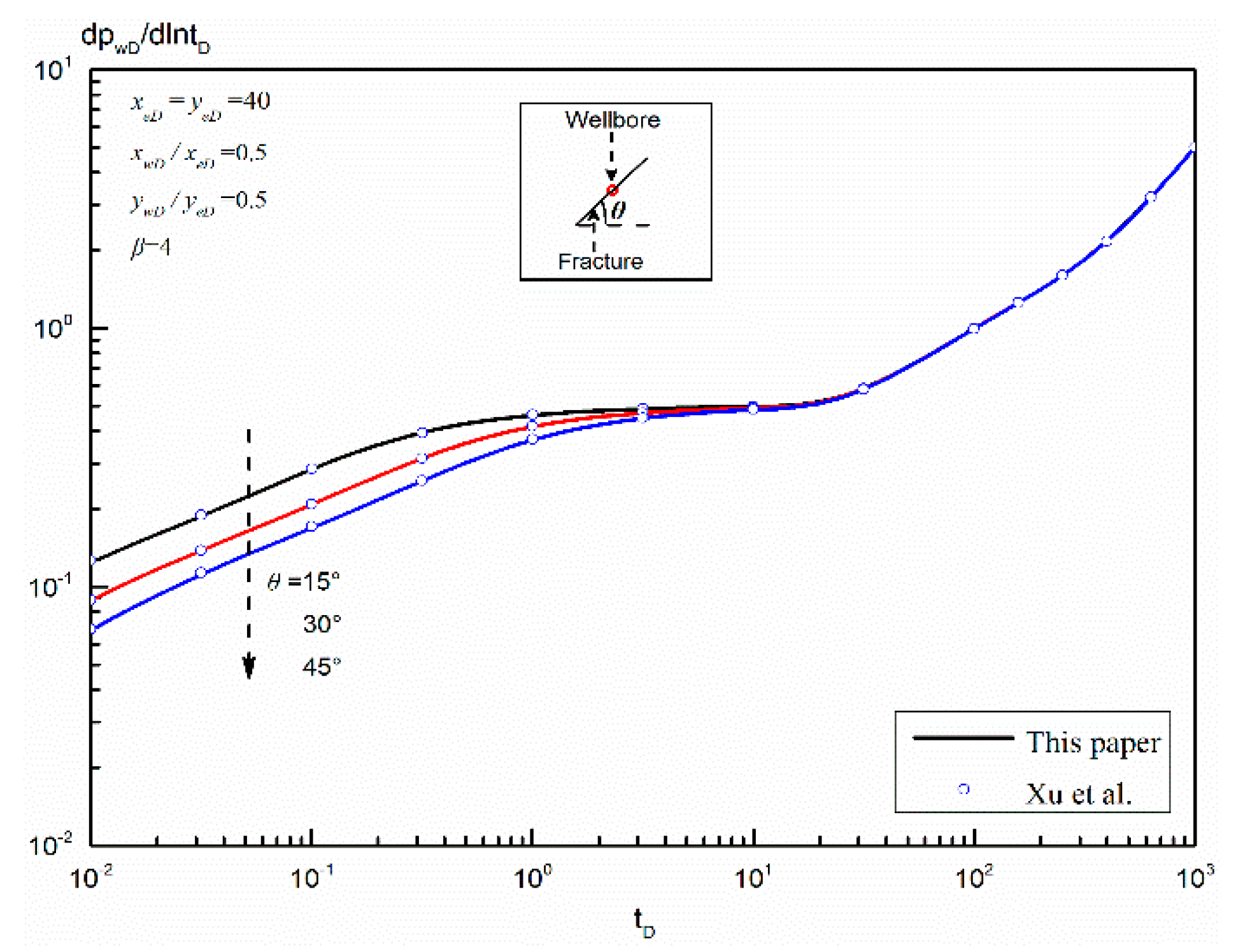
4.2. Validation of a Finite-Conductivity Fracture with an Azimuth Angle in an Anisotropic Reservoir
5. Pseudo-Steady-State Flow Parameters
5.1. Pseudo-Steady-State Constant
- (1)
- Rate integral function:
- (2)
- Rate integral-derivative function:
5.2. Pseudo-Skin Factor
6. Results and Discussion
6.1. Analysis of Parameters’ Influence on Pseudo-Steady-State Flow
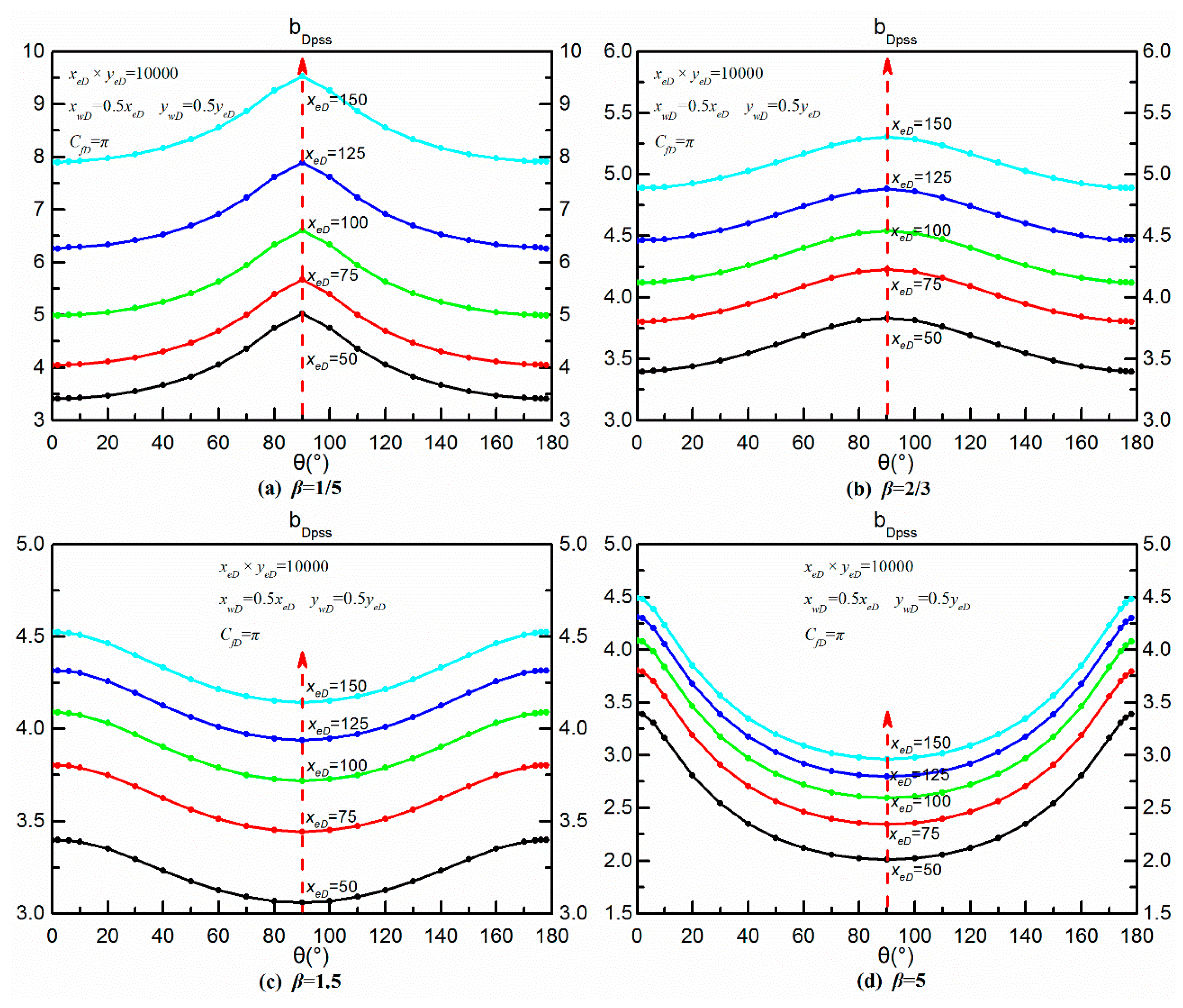
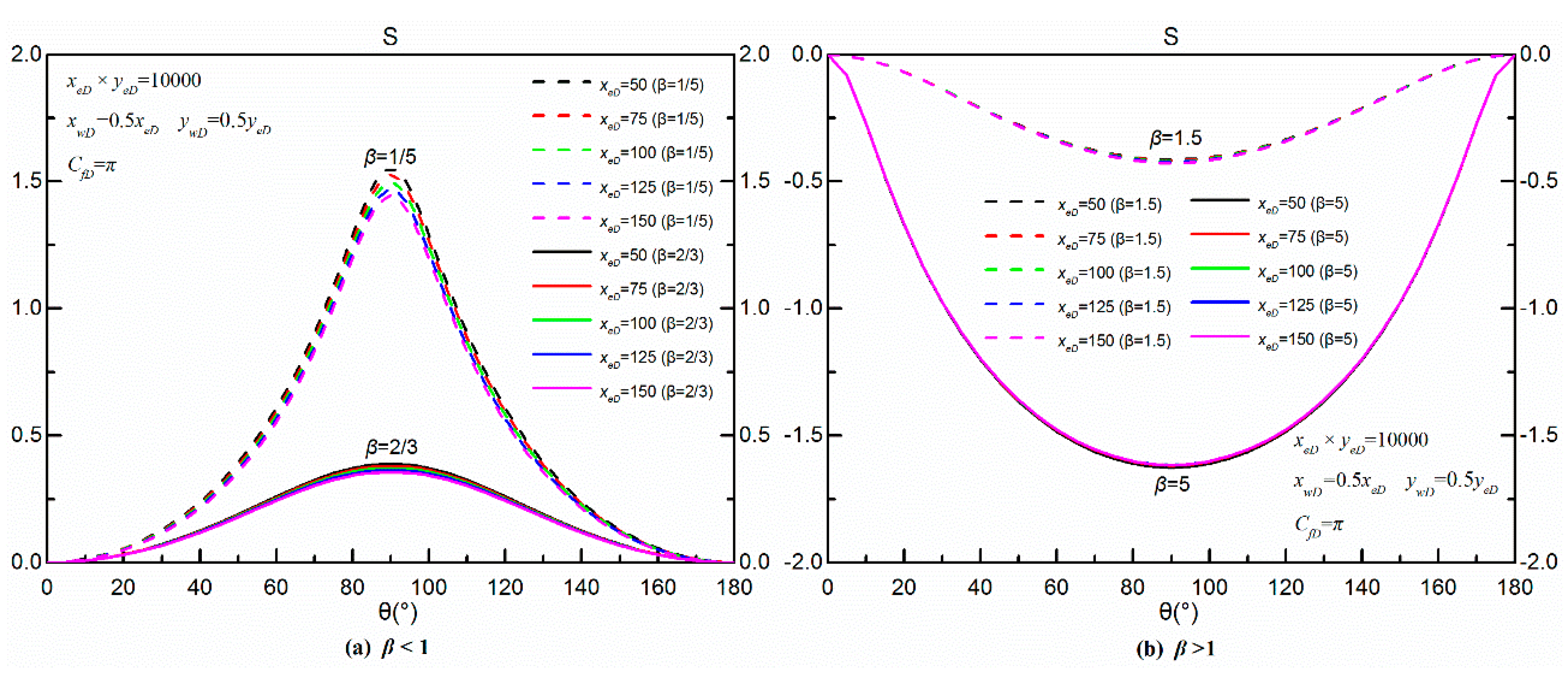
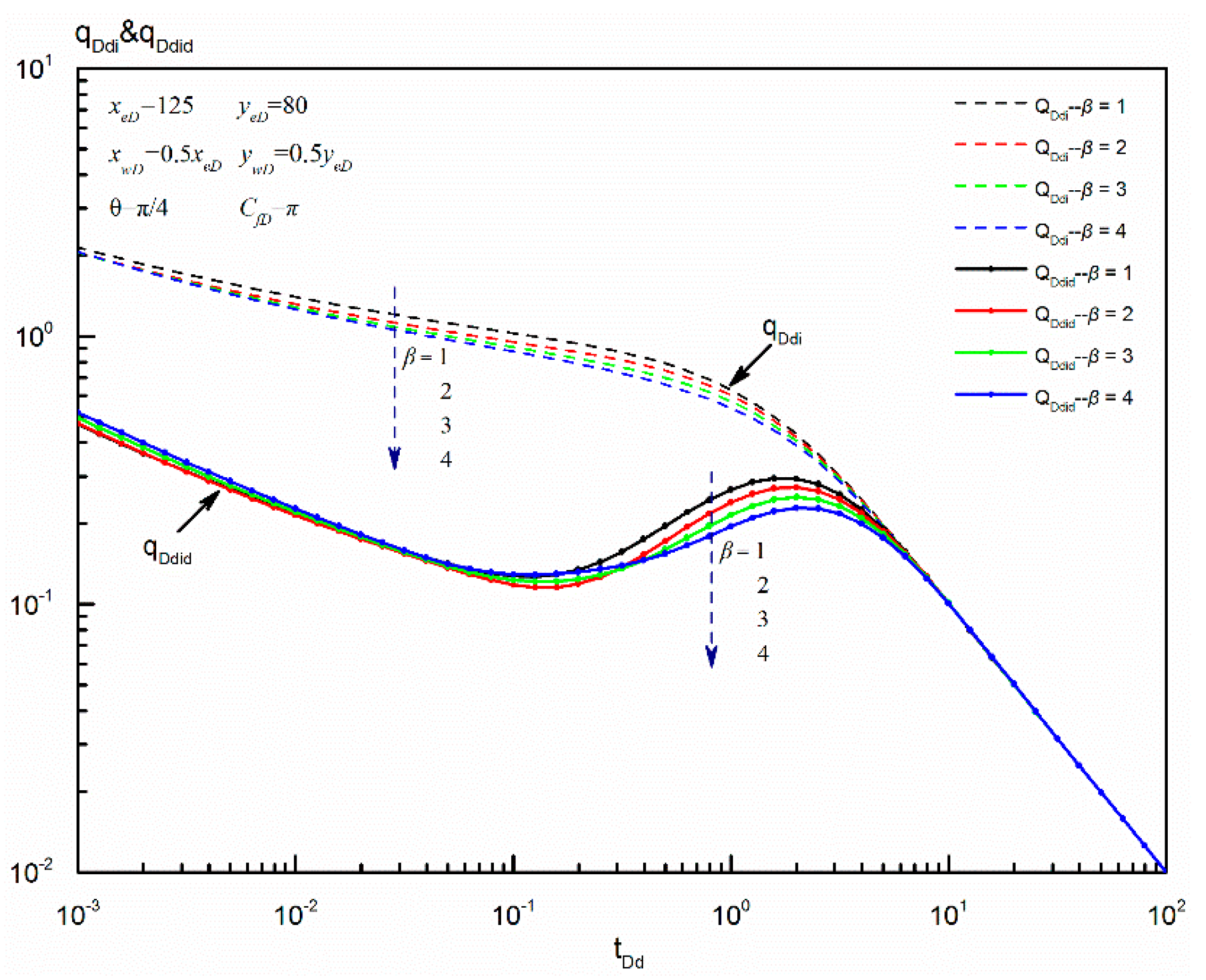
6.1.1. Permeability Anisotropy
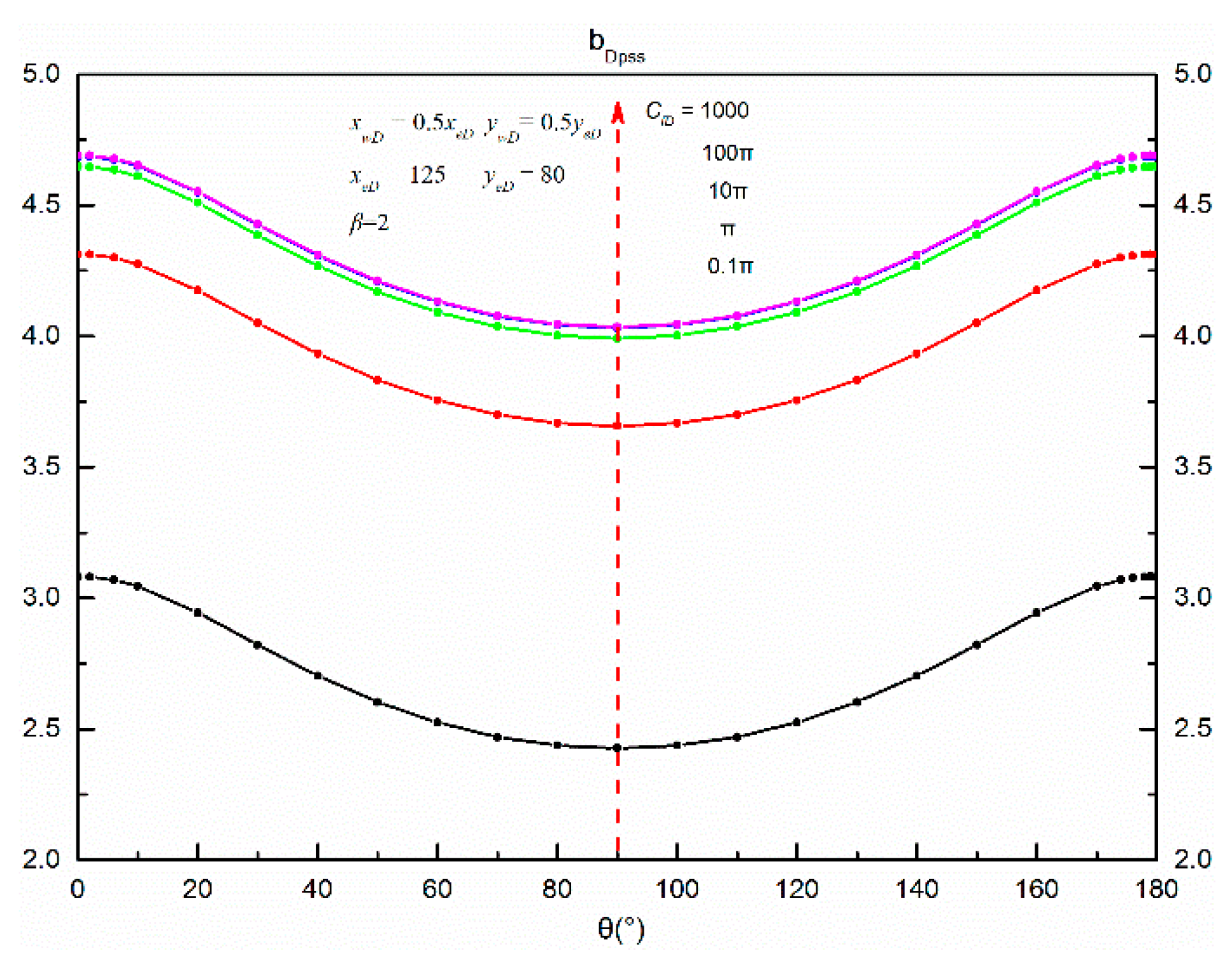
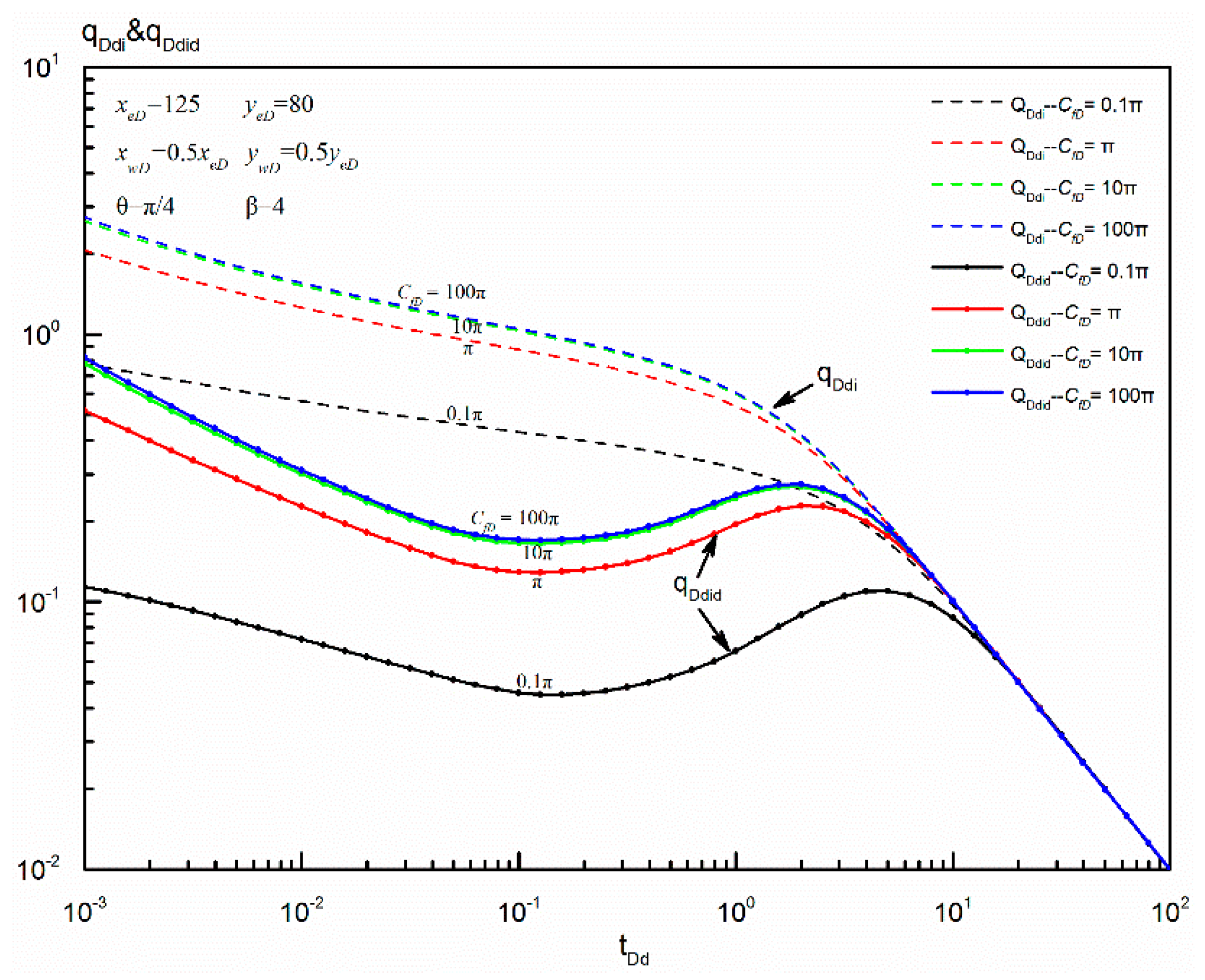
6.1.2. Fracture Conductivity
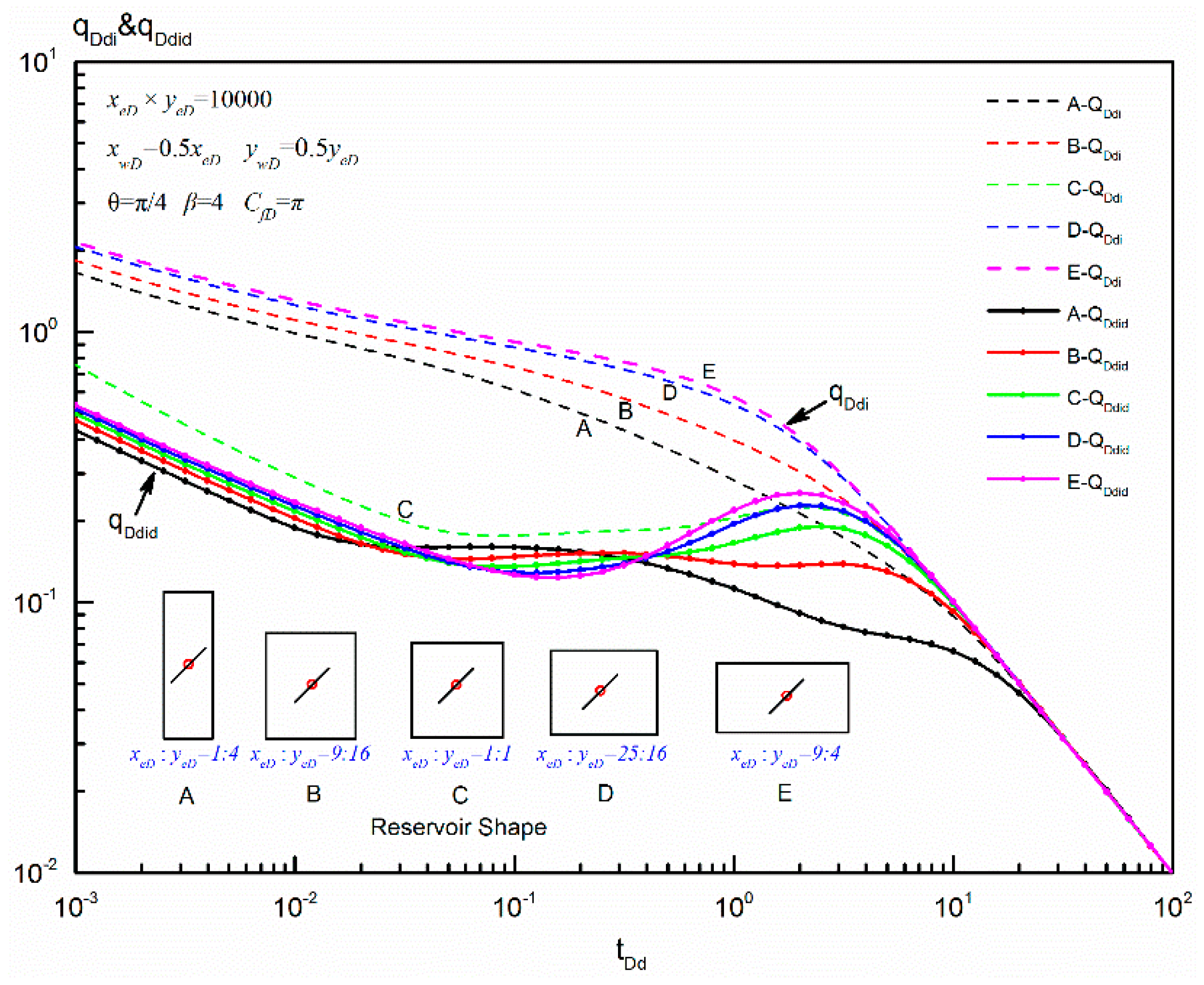
6.1.3. Reservoir Shape
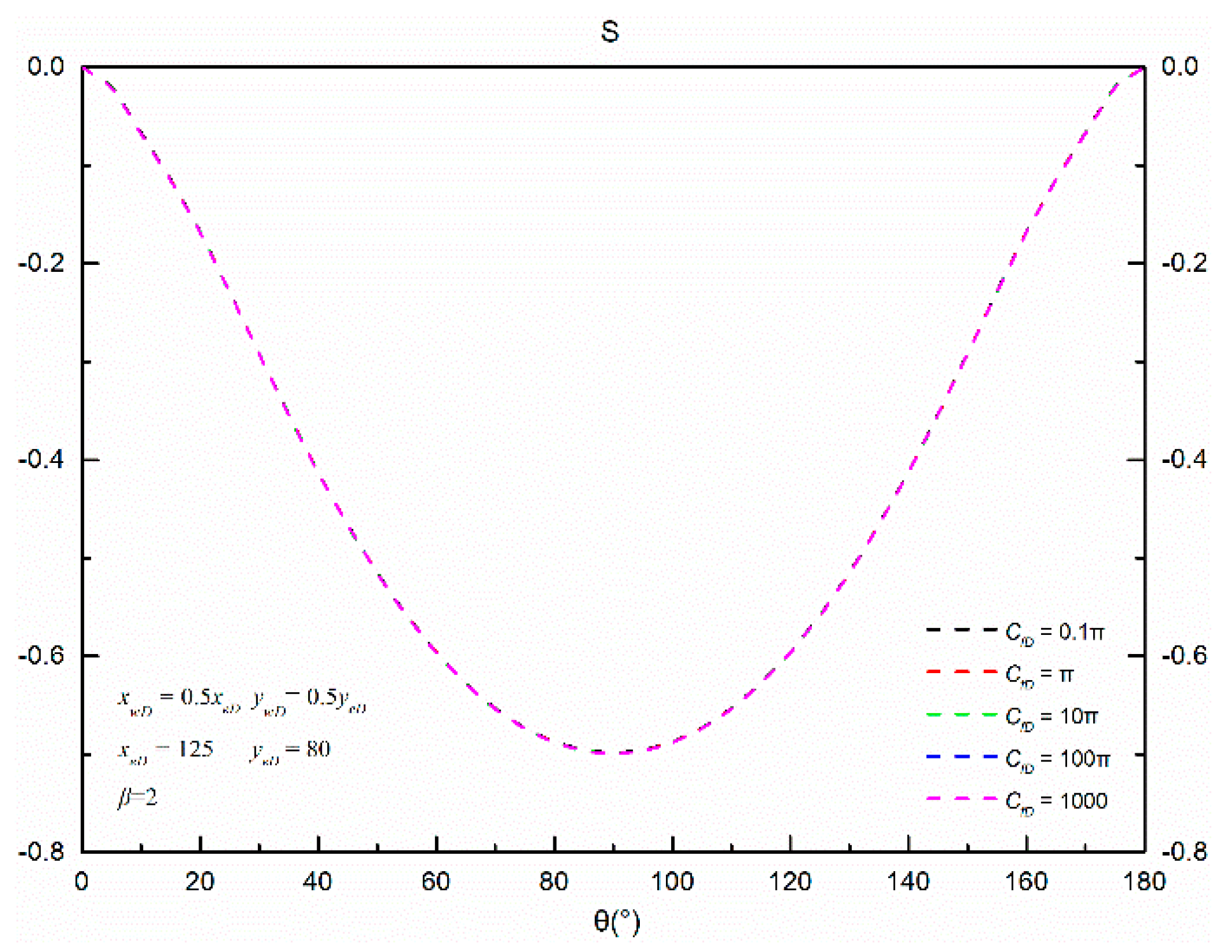
6.2. Analysis of the Relationship between Pseudo-Steady-State Constant and Pseudo-Skin Factor
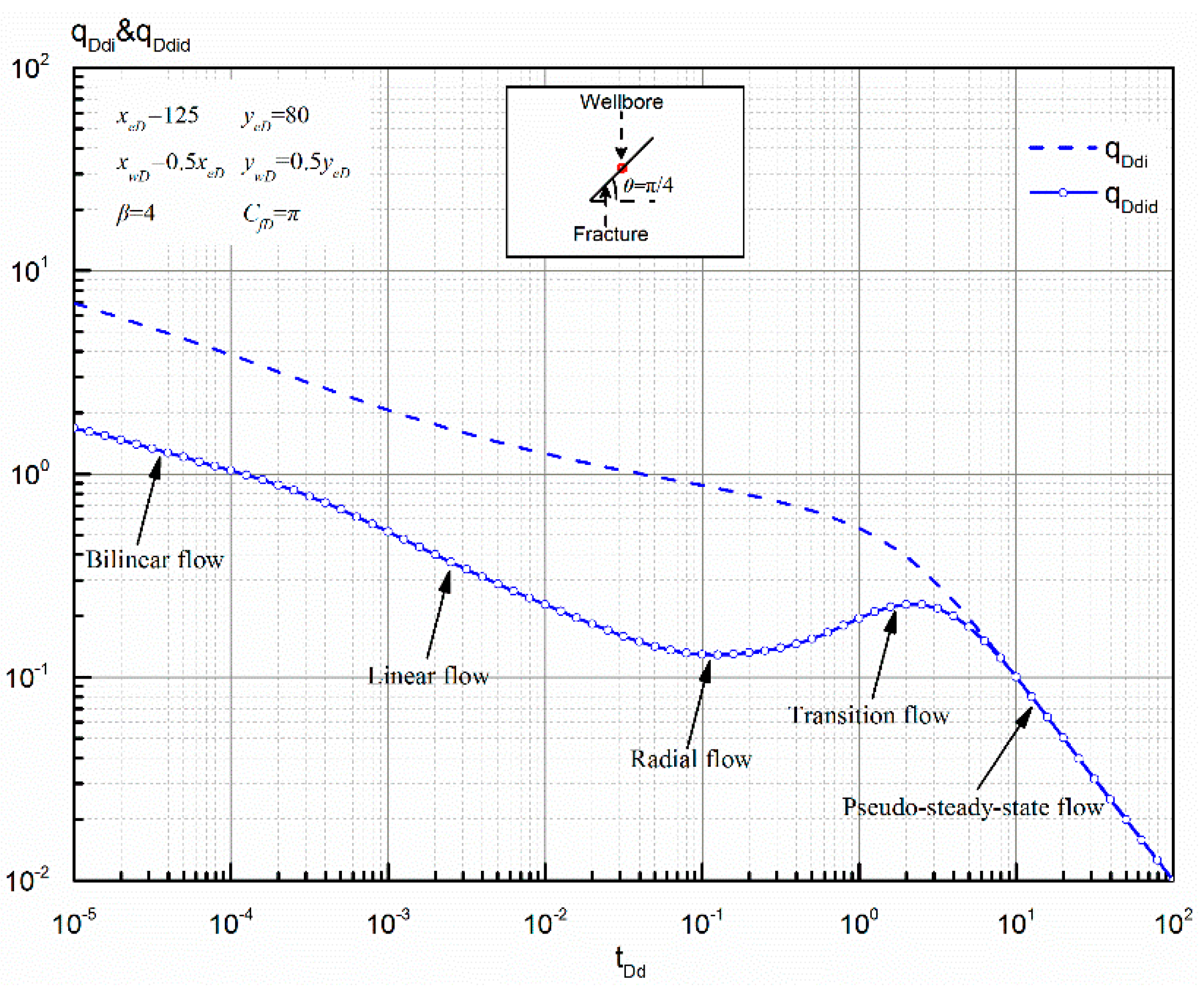
6.3. Establishment of Blasingame Format Rate Decline Curves by Using Pseud-Steady-State Constant
7. Conclusions
- (1)
- The equivalent wellbore pressure solution for a single finite-conductivity fracture with or without an azimuth angle in an anisotropic rectangular reservoir was developed by using the point-source function and spatial integral method. It showed excellent agreement with the work of Chen and Raghavan, and Xu et al., which demonstrates that the proposed pressure solution is reliable.
- (2)
- An approximate pressure solution for this model in the pseudo-steady-state flow regime was derived with the asymptotic analysis method. The expressions of pseudo-steady-state constant and pseudo-skin factor were further obtained on the basis of their definitions.
- (3)
- Except for the isotropic reservoirs, all the and curves are symmetric around the vertical line, and form a hump or groove shape. Fracture conductivity and reservoir shape almost have no effect on the pseudo-skin factor for a given anisotropic reservoir. The optimized fracture direction mainly depends on permeability anisotropy and is perpendicular to the principal permeability axis.
- (4)
- Based on the definitions of pseudo-steady-state constant and pseudo-skin factor, a new formula to calculate the pseudo-skin factor was successfully proposed with their relationship. The comparison of the pseudo-skin factors calculated from its definition and the new formula at different azimuth angle verified the reliability of this new formula.
- (5)
- As an application of pseudo-steady-state constant, a set of Blasingame format rate decline curves for the proposed model were established. Five flow regimes can be observed on the typical Blasingame format curves. Fracture azimuth angle mainly affects the early-time flow, and permeability anisotropy mainly affects the intermediate-time flow. But fracture conductivity and reservoir shape have a strong effect on both the early- and intermediate-time flow. All the curves normalize in the pseudo-steady-state flow regime. Additionally, there is an optimized fracture conductivity for a given anisotropic rectangular reservoir.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| Field Variables | |
| Distance in the x axis, m | |
| Distance in the y axis, m | |
| Distance in the x or y axis, m | |
| Reservoir length in the x axis, m | |
| Reservoir length in the y axis, m | |
| Reservoir length in the x or y axis, m | |
| Reservoir thickness, m | |
| Fracture half length, m | |
| Fracture width, m | |
| Permeability in the x-axis, 10−3μm2 | |
| Permeability in the y-axis, 10−3μm2 | |
| Equivalent system permeability, 10−3μm2 | |
| Fracture permeability, 10−3μm2 | |
| Angle between x axis and the extension direction of fracture, deg | |
| Reservoir initial pressure, MPa | |
| Porosity, fraction | |
| Fluid viscosity, mPa·s | |
| Total compressibility, 1/MPa | |
| Permeability anisotropic factor | |
| Wellbore flow rate, m3/d | |
| Principal diffusivities | |
| Correlation coefficient, n = 0,1,2…… | |
| Dimensionless Variables | |
| Dimensionless pressure in real time domain | |
| Dimensionless pressure in Laplace domain | |
| Dimensionless point-source solution in an anisotropic rectangular reservoir in Laplace domain | |
| Dimensionless pressure for an infinite-conductivity fracture in real time domain | |
| Dimensionless pressure for an infinite-conductivity fracture in Laplace domain | |
| Dimensionless pressure for an infinite-conductivity fracture paralleled to the x axis in real time domain | |
| Dimensionless pressure for an infinite-conductivity fracture paralleled to the x axis in Laplace domain | |
| Dimensionless pseudo-steady-state pressure in real time domain | |
| Dimensionless time | |
| Dimensionless time based on drainage area | |
| Dimensionless decline time | |
| Dimensionless pseudo-steady-state constant | |
| Dimensionless decline rate | |
| Dimensionless decline rate integral | |
| Dimensionless decline rate integral derivative | |
| Dimensionless distance in the x axis | |
| Dimensionless distance in the y axis | |
| Dimensionless reservoir length in the x axis | |
| Dimensionless reservoir width in the y axis | |
| Dimensionless wellbore location in the x axis | |
| Dimensionless wellbore location in the y axis | |
| Dimensionless coordinate in the x axis | |
| Dimensionless coordinate in the y axis | |
| Dimensionless fracture conductivity | |
| Impact function of dimensionless fracture conductivity in real time domain | |
| Impact function of dimensionless fracture conductivity in Laplace domain | |
| Dimensionless coefficient, i = 1, 2, 3…… | |
| Dimensionless coefficient related to and | |
| Dimensionless pseudo-skin factor | |
| Dimensionless time variable in Laplace domain | |
| Dimensionless integral variable | |
| Dimensionless coefficient, n = 0, 1, 2…… | |
| Dimensionless pseudo-steady-state coefficient, i = 1, 2 | |
| Dimensionless coefficient, i = 1, 2 | |
| Dimensionless productivity index | |
Appendix A. Derivation of Our Model’s Pseudo-Steady-State Constant
References
- Czarnota, R.; Stopa, J.; Janiga, D.; Kosowski, P.; Wojnarowski, P. Semianalytical horizontal well length optimization under pseudosteady-state conditions. In Proceedings of the IEEE 2nd International Conference on Smart Grid and Smart Cities (ICSGSC 2018), Kuala Lumpur, Malaysia, 12–14 August 2018; pp. 68–72. [Google Scholar]
- Ghahri, P.; Jamiolahmadi, M.; Alatefi, E.; Wilkinson, D.; Dehkordi, F.S.; Hamidi, H. A new and simple model for the prediction of horizontal well productivity in gas condensate reservoirs. Fuel 2018, 223, 431–450. [Google Scholar] [CrossRef] [Green Version]
- Fetkovich, M.J. Decline curve analysis using type curves. J. Pet. Technol. 1980, 32, 1065–1077. [Google Scholar] [CrossRef]
- McCray, T.L. Reservoir Analysis Using Production Decline Data and Adjusted Time. Ph.D. Thesis, A&M University, College Station, TX, USA, 1990. [Google Scholar]
- Blasingame, T.A.; McCray, T.L.; Lee, W.J. Decline Curve Analysis for Variable Pressure Drop/Variable Flowrate Systems. In Proceedings of the SPE Gas Technology Symposium, Houston, TX, USA, 22–24 January 1991. [Google Scholar]
- Pratikno, H.; Rushing, J.A.; Blasingame, T.A. Decline curve analysis using type curves-fractured wells. In Proceedings of the SPE Annual Technical Conference and Exhibition, Denver, CO, USA, 5–8 October 2003. [Google Scholar]
- Hagoort, J. The productivity of a well with a vertical infinite-conductivity fracture in a rectangular closed reservoir. SPE J. 2009, 14, 715–720. [Google Scholar] [CrossRef]
- Cinco-Ley, H.; Ramey, H.J., Jr.; Miller, F.G. Unsteady-state pressure distribution created by a well with an inclined fracture. In Proceedings of the Fall Meeting of the Society of Petroleum Engineers of AIME, Dallas, TX, USA, 28 September–1 October 1975. [Google Scholar]
- Reynolds, A.C.; Chen, J.C.; Raghavan, R. Pseudoskin factor caused by partial penetration. J. Pet. Technol. 1984, 36, 2197–2210. [Google Scholar] [CrossRef]
- Ozkan, E. Performance of horizontal wells. Ph.D. Thesis, Tulsa University, Tulsa, OH, USA, 1988. [Google Scholar]
- Tang, Y.; Ozkan, E.; Kelkar, M.; Yildiz, T. Fast and accurate correlations to compute the perforation pseudoskin and productivity of horizontal wells. In Proceedings of the SPE Annual Technical Conference and Exhibition, New Orleans, LA, USA, 30 September–3 October 2001. [Google Scholar]
- Jia, P.; Cheng, L.; Huang, S.; Liu, H. Pressure-transient analysis of a finite-conductivity inclined fracture connected to a slanted wellbore. SPE J. 2016, 21, 522–537. [Google Scholar] [CrossRef]
- Stopa, J.; Wiśniowski, R.; Wojnarowski, P.; Janiga, D.; Skrzypaszek, K. Integrated approach to drilling project in unconventional reservoir using reservoir simulation; E3S Web of Conferences. EDP Sci. 2018, 35, 01002. [Google Scholar]
- Jia, P.; Cheng, L.; Huang, S.; Xue, Y.; Clarkson, C.R.; Williams-Kovacs, J.D.; Wang, D. Dynamic coupling of analytical linear flow solution and numerical fracture model for simulating early-time flowback of fractured tight oil wells (planar fracture and complex fracture network). J. Pet. Sci. Eng. 2019, 177, 1–23. [Google Scholar] [CrossRef]
- Kong, B.; Chen, S. Numerical simulation of fluid flow and sensitivity analysis in rough-wall fractures. J. Pet. Sci. Eng. 2018, 168, 546–561. [Google Scholar] [CrossRef]
- Dejam, M. Advective-diffusive-reactive solute transport due to non-Newtonian fluid flows in a fracture surrounded by a tight porous medium. Int. J. Heat Mass Transf. 2019, 128, 1307–1321. [Google Scholar] [CrossRef]
- Wu, Z.W.; Cui, C.Z.; Lv, G.Z.; Bing, S.X.; Cao, G. A multi-linear transient pressure model for multistage fractured horizontal well in tight oil reservoirs with considering threshold pressure gradient and stress sensitivity. J. Pet. Sci. Eng. 2019, 172, 839–854. [Google Scholar] [CrossRef]
- Wu, S.H.; Xing, G.Q.; Cui, Y.D.; Wang, B.H.; Shi, M.Y.; Wang, M.X. A semi-analytical model for pressure transient analysis of hydraulic reorientation fracture in an anisotropic reservoir. J. Pet. Sci. Eng. 2019, 179, 228–243. [Google Scholar] [CrossRef]
- Luo, W.J.; Tang, C.F. Pressure-transient analysis of multiwing fractures connected to a vertical wellbore. SPE J. 2015, 20, 360–367. [Google Scholar] [CrossRef]
- Kucuk, F.; Brigham, W.E. Transient flow in elliptical systems. SPE J. 1979, 19, 401–410. [Google Scholar] [CrossRef]
- Kuchuk, F.J.; Goode, P.A.; Wilkinson, D.J.; Thambynayagam, R.K.M. Pressure-transient behavior of horizontal wells with and without gas cap or aquifer. SPE Form. Eval. 1991, 6, 86–94. [Google Scholar] [CrossRef]
- Ozkan, E.; Raghavan, R. New solutions for well-test-analysis problems: Part 1-analytical considerations. SPE Form. Eval. 1991, 6, 359–368. [Google Scholar] [CrossRef]
- Yildiz, T.; Ozkan, E. Influence of Areal Anisotropy on Horizontal Well Performance. In Proceedings of the SPE Annual Technical Conference and Exhibition, San Antonio, TX, USA, 5–8 October 1997. [Google Scholar]
- Spivey, J.P.; Lee, W.J. Estimating the pressure-transient response for a horizontal or a hydraulically fractured well at an arbitrary orientation in an anisotropic reservoir. SPE Reserv. Eval. Eng. 1999, 2, 462–469. [Google Scholar] [CrossRef]
- Xu, W.; Wang, X.; Xing, G.; Wang, J. Pressure-transient analysis for a vertically fractured well at an arbitrary azimuth in a rectangular anisotropic reservoir. J. Pet. Sci. Eng. 2017, 159, 279–294. [Google Scholar] [CrossRef]
- Gringarten, A.C.; Ramey, H.J., Jr. The use of source and Green’s functions in solving unsteady-flow problems in reservoirs. SPE J. 1973, 13, 285–296. [Google Scholar]
- Gringarten, A.C.; Ramey, H.J., Jr.; Raghavan, R. Unsteady-state pressure distributions created by a well with a single infinite-conductivity vertical fracture. SPE J. 1974, 14, 347–360. [Google Scholar] [CrossRef]
- Gradshteyn, I.S.; Ryzhik, I.M. Table of Integrals, Series, and Products; Academic Press: London, UK, 2007. [Google Scholar]
- Wang, X.; Luo, W.; Hou, X.; Wang, J. Pressure transient analysis of multi-stage fractured horizontal wells in boxed reservoirs. Petrol. Explor. Dev. 2014, 41, 82–87. [Google Scholar] [CrossRef]
- Stehfest, H. Algorithm 368: Numerical inversion of Laplace transforms. Commun. ACM 1970, 13, 47–49. [Google Scholar] [CrossRef]
- Chen, C.C.; Raghavan, R.A. Multiply-fractured horizontal well in a rectangular drainage region. SPE J. 1997, 2, 455–465. [Google Scholar] [CrossRef]
- Blasingame, T.A.; Johnston, J.L.; Lee, W.J. Type-curve analysis using the pressure integral method. In Proceedings of the SPE California Regional Meeting, Bakersfield, CA, USA, 5–7 April 1989. [Google Scholar]
- Dinh, A.V.; Tiab, D. Pressure-transient analysis of a well with an inclined hydraulic fracture. SPE Reserv. Eval. Eng. 2010, 13, 845–860. [Google Scholar] [CrossRef]
- Guo, J.C.; Nie, R.S.; Jia, Y.L. Dual permeability flow behavior for modeling horizontal well production in fractured-vuggy carbonate reservoirs. J. Hydrol. 2012, 464–465, 281–293. [Google Scholar] [CrossRef]
- Nie, R.S.; Meng, Y.F.; Guo, J.C.; Jia, Y.L. Modeling transient flow behavior of a horizontal well in a coal seam. Int. J. Coal Geol. 2012, 92, 54–68. [Google Scholar] [CrossRef]
- Wang, M.; Fan, Z.; Xing, G.; Zhao, W.; Song, H.; Su, P. Rate decline analysis for modeling volume fractured well production in naturally fractured reservoirs. Energies 2018, 11, 43. [Google Scholar]
- Riley, M.F.; Brigham, W.E.; Horne, R.N. Analytical solutions for elliptical finite-conductivity fractures. In Proceedings of the SPE Annual Technical Conference and Exhibition, Dallas, TX, USA, 6–9 October 1991. [Google Scholar]
- Wang, L.; Wang, X.; Ding, X.; Zhang, L.; Li, C. Rate decline curves analysis of a vertical fractured well with fracture face damage. J. Energy Resour. Technol. 2012, 134, 032803. [Google Scholar]




| Parameters | Definitions |
|---|---|
| Dimensionless time | |
| Dimensionless pressure | |
| Dimensionless coordinate in the x- and y-axis | , |
| Dimensionless wellbore coordinate in the x- and y-axis | , |
| Dimensionless length of the reservoir in the x axis | |
| Dimensionless width of the reservoir in the y axis | |
| Permeability anisotropic factor | |
| Dimensionless fracture conductivity | |
| Dimensionless time based on drainage area |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xing, G.; Wang, M.; Wu, S.; Li, H.; Dong, J.; Zhao, W. Pseudo-Steady-State Parameters for a Well Penetrated by a Fracture with an Azimuth Angle in an Anisotropic Reservoir. Energies 2019, 12, 2449. https://doi.org/10.3390/en12122449
Xing G, Wang M, Wu S, Li H, Dong J, Zhao W. Pseudo-Steady-State Parameters for a Well Penetrated by a Fracture with an Azimuth Angle in an Anisotropic Reservoir. Energies. 2019; 12(12):2449. https://doi.org/10.3390/en12122449
Chicago/Turabian StyleXing, Guoqiang, Mingxian Wang, Shuhong Wu, Hua Li, Jiangyan Dong, and Wenqi Zhao. 2019. "Pseudo-Steady-State Parameters for a Well Penetrated by a Fracture with an Azimuth Angle in an Anisotropic Reservoir" Energies 12, no. 12: 2449. https://doi.org/10.3390/en12122449





