The Simulation of an Automotive Air Spring Suspension Using a Pseudo-Dynamic Procedure
Abstract
:1. Introduction
2. The Air Spring Stiffness
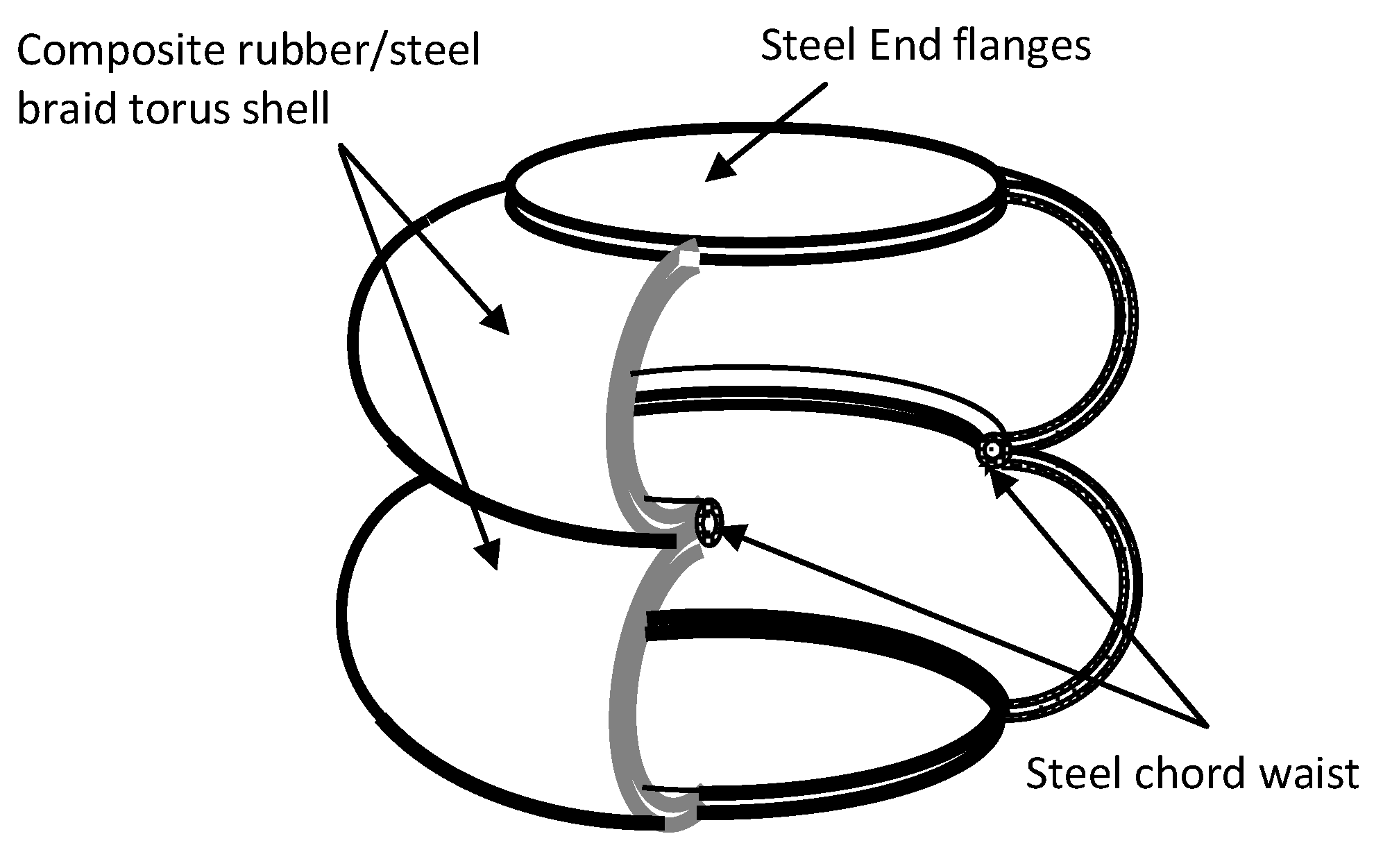
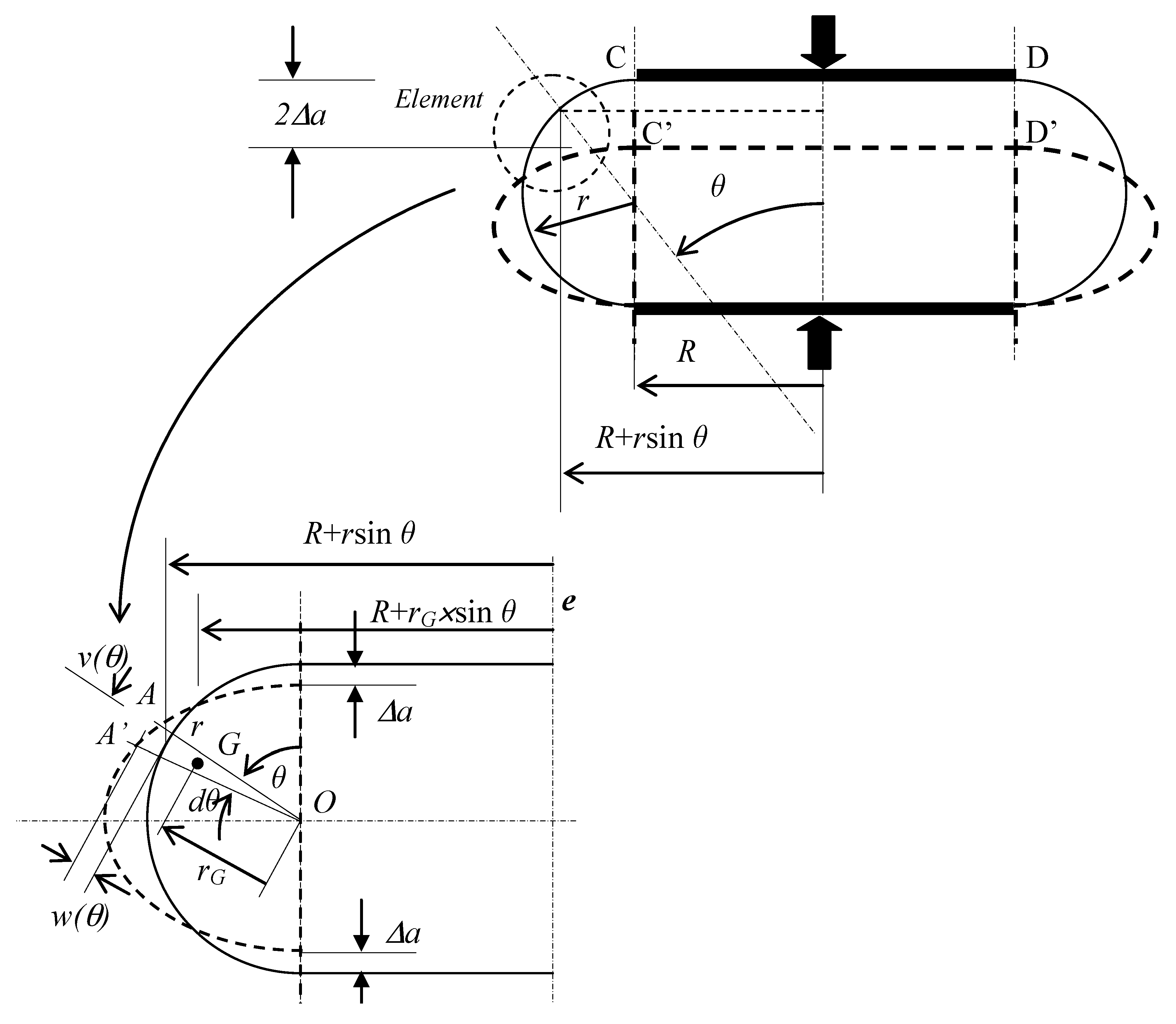
2.1. The Displacement Field of the Toroidal Shell
- r is the meridian radius
- θ is the meridian angle
- R is the longitudinal (or circumferential) radius
- w is the transverse (or normal) displacement
- v is the meridian (membrane) displacement. This displacement does not participate in the simplified model to calculate the work done by the internal pressure in the shell deformation.
2.2. The Pneumatic Stiffness
- (a)
- The first model assumes that only the compressed air participates as an elastic body in the evaluation of the spring stiffness. The shell torus enclosing the air does not contribute to the suspension stiffness;
- (b)
- The second model consists in superimposing the structural stiffness of the shell torus to the one of the air under compression, as two springs working in parallel.
2.3. The Equilibrium Equation: The Iterative Newton–Raphson Method
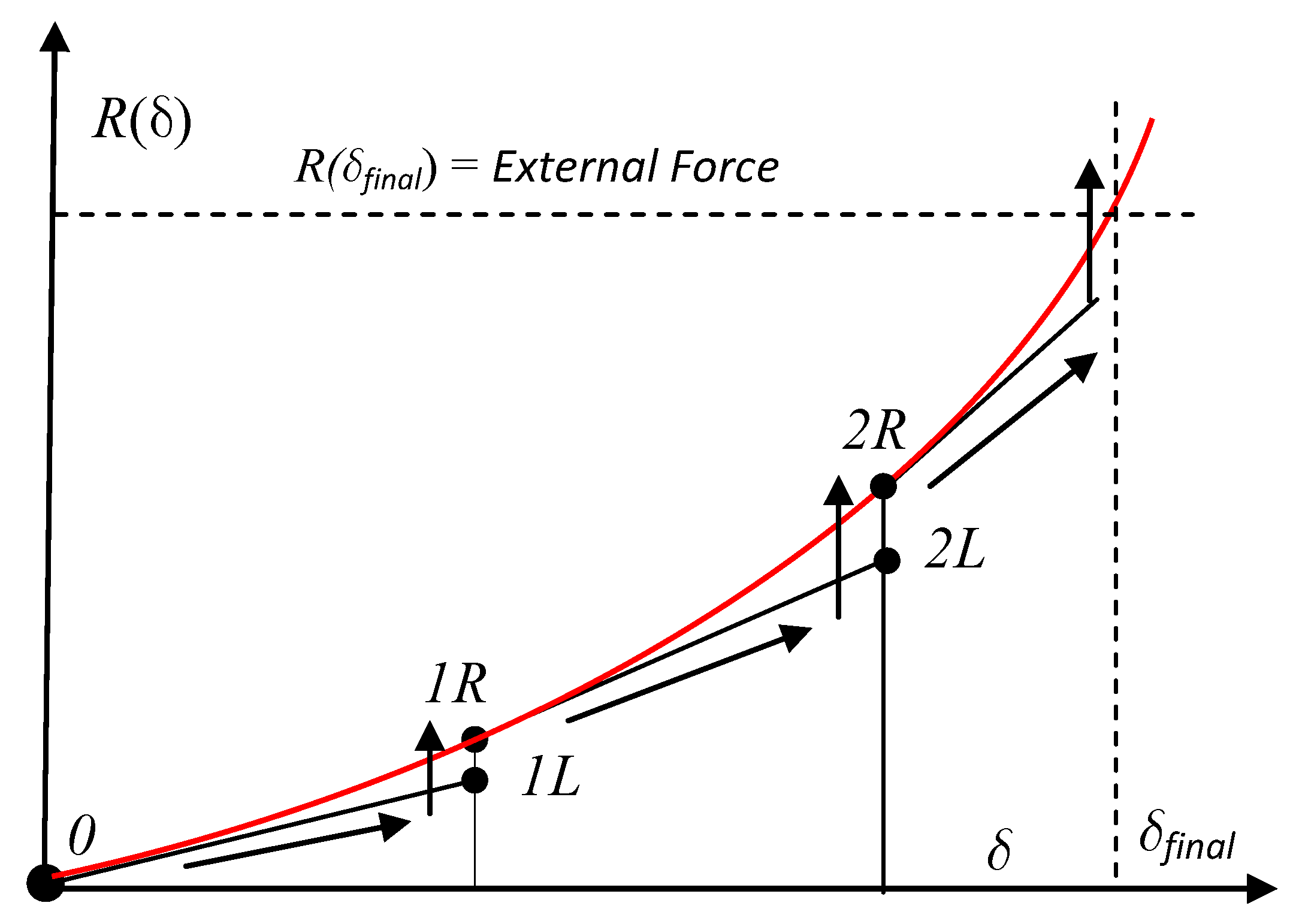
- A continuous function F(a) can be written for an increment of its independent variable a, as F(a + Δa) ≅ F(a)+Δt × F’(a) from the Taylor’s formula up to a first-order expansion. Naming F(a + Δa) = Fn+1 and F(a) = Fn; a + Δa = an+1 and a = an, then, the previous approach is equivalent to: Fn+1 − Fn ≅ (an+1 − an) × F’(a). This formula models a linearized evolution of the structure constitutive relation for displacements inside the interval [a, a + Δa]. Graphically, this equation corresponds to a tangent line at a point of the curve that effectively represents the constitutive behavior of a structure, eventually with elastic, yet also represents the non-linear behavior of the internal reaction force vector from a prescribed displacements vector, as sketched in Figure 4. As point F(a + Δa) here obtained does not correspond to the effective reaction of the structure (this one given by Equation (7)), there is a residual difference to the real value, which is a parameter suitable to control the iteration pitch, as depicted in Figure 4.
- The points labeled with “L” result from the linear approach, while points from initial internal reactions are labeled with “R” (Figure 4). Past the iteration, the linearly obtained next value is compared with the effective one, where the difference of effective and linear points is the residual value for the precision control of the iteration. This process is schematically represented by arrows in Figure 4, and is concluded when the final linear value of the internal reaction vector is close to the real one (the external force) by an error smaller than the prescribed tolerance.
2.4. Example of Loading a Single Cell Bellows of a Pneumatic Suspension
- Radius of the meridian line r = 50 mm
- Radius of the circumference line (passing through the center of the transverse meridian circumference) R = 200 mm
- Initial pressure P = 2 bars (≅0.2 MPa)
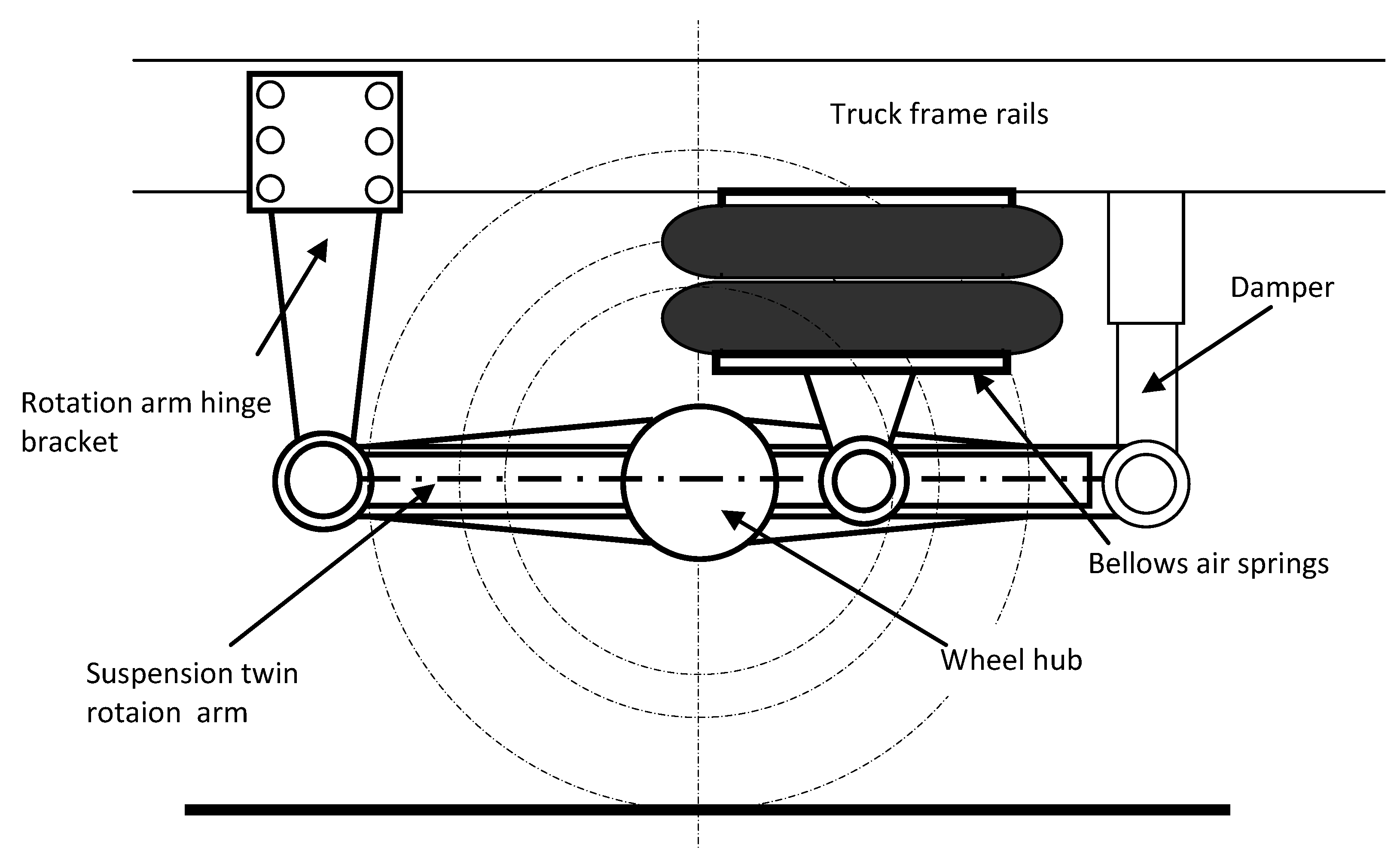
3. Pseudo-Dynamic Simulation of the Automotive Suspension
3.1. Applications to the Dynamic Analysis of Automotive Suspensions
3.2. The Direct Time Integration Algorithm: The Newark Constant Acceleration Method. Application to Pseudo-Dynamic Methods
3.3. Description of the Method and Application to the Dynamic Analysis
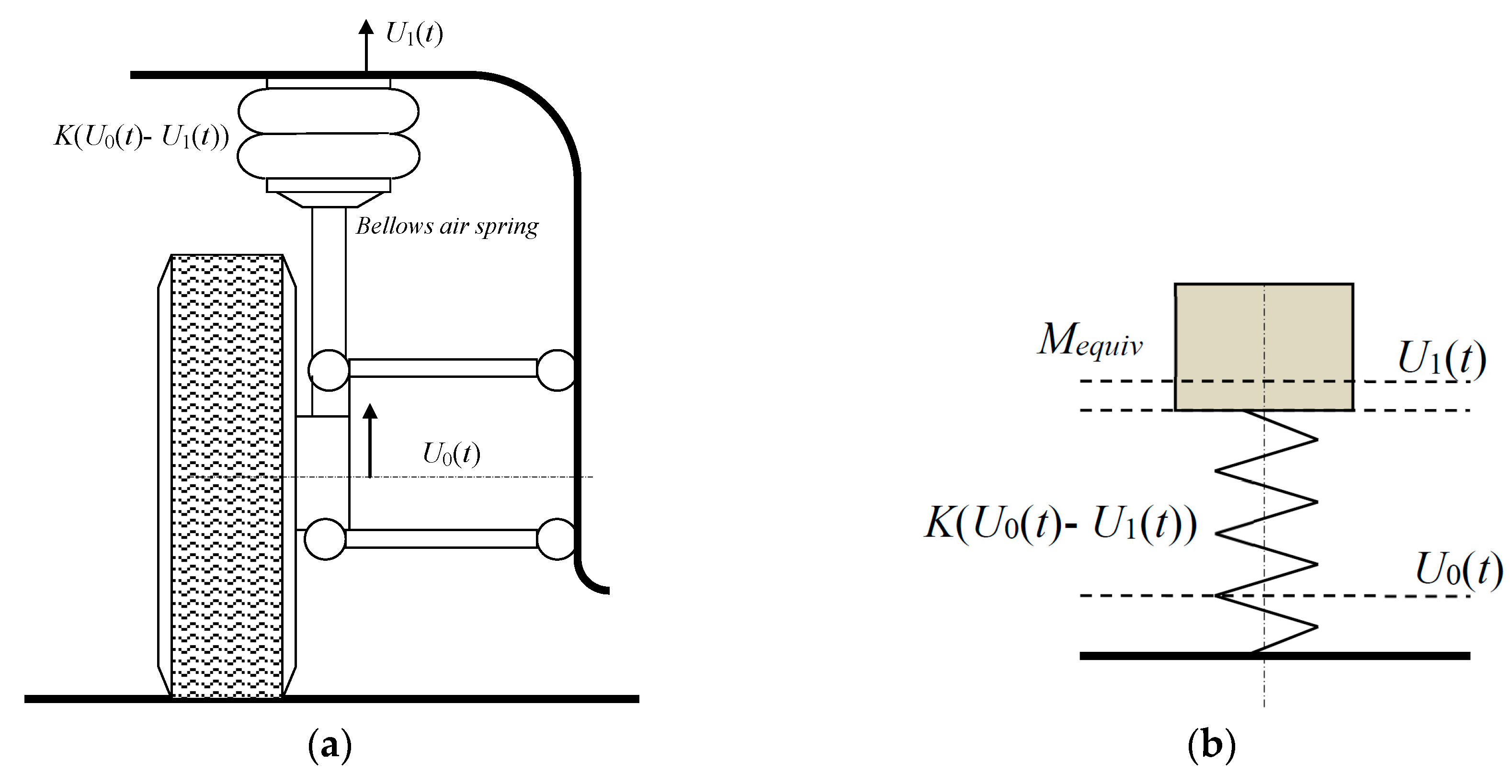
3.3.1. Operation Principles
3.3.2. Engineering Design of the Rig and Applications
- The number of degrees of freedom to obtain accurate results may be predictably high, a less favorable attribute in the design of pseudo-dynamic test rigs;
- The size and engineering complexity of the equipment for pseudo-dynamic testing are not remarkable advantages considering that a real-time dynamic analysis test rig records internal reactions and damping forces. In turn, with pseudo-dynamic methods, viscous damping forces have to be numerically inserted in the time integration algorithm, as referred above.
- The dynamic analysis of some components in the automotive industry cannot be approached only with a set of time-dependent displacements, since the inclusion of nodal rotations in discretized models is necessary. This requirement makes the adequate design of the test rig more expensive, with an additional need of implementing angular transducers, more elaborate test-specimen fixtures, and specific load cells, which is an option that may not guarantee accurate results.
- Sprung mass Ms = 340 kg;
- Damping Constant Cs = 2.25 kNs/m
- Spring Stiffness Ks = 31.2 kN/m.
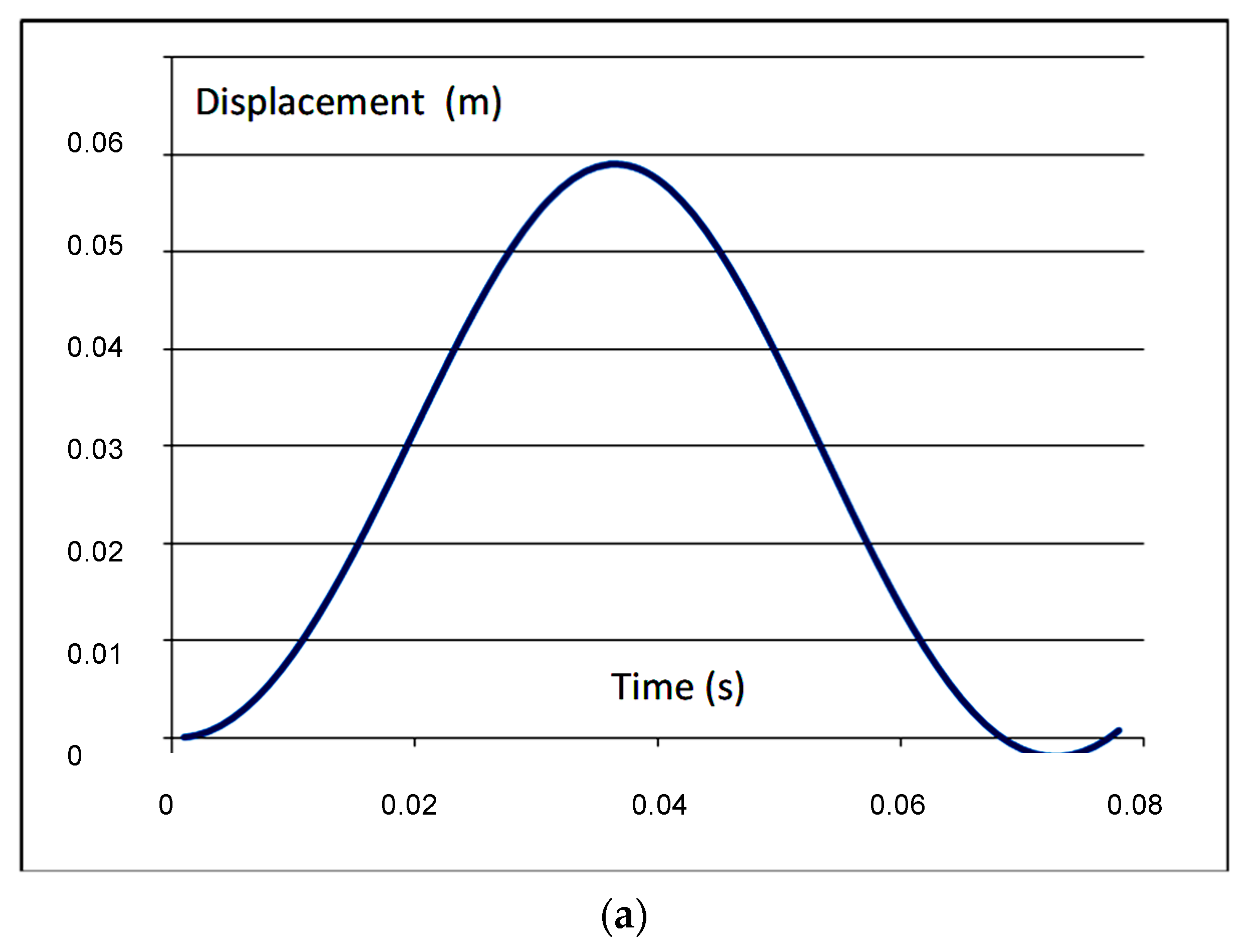
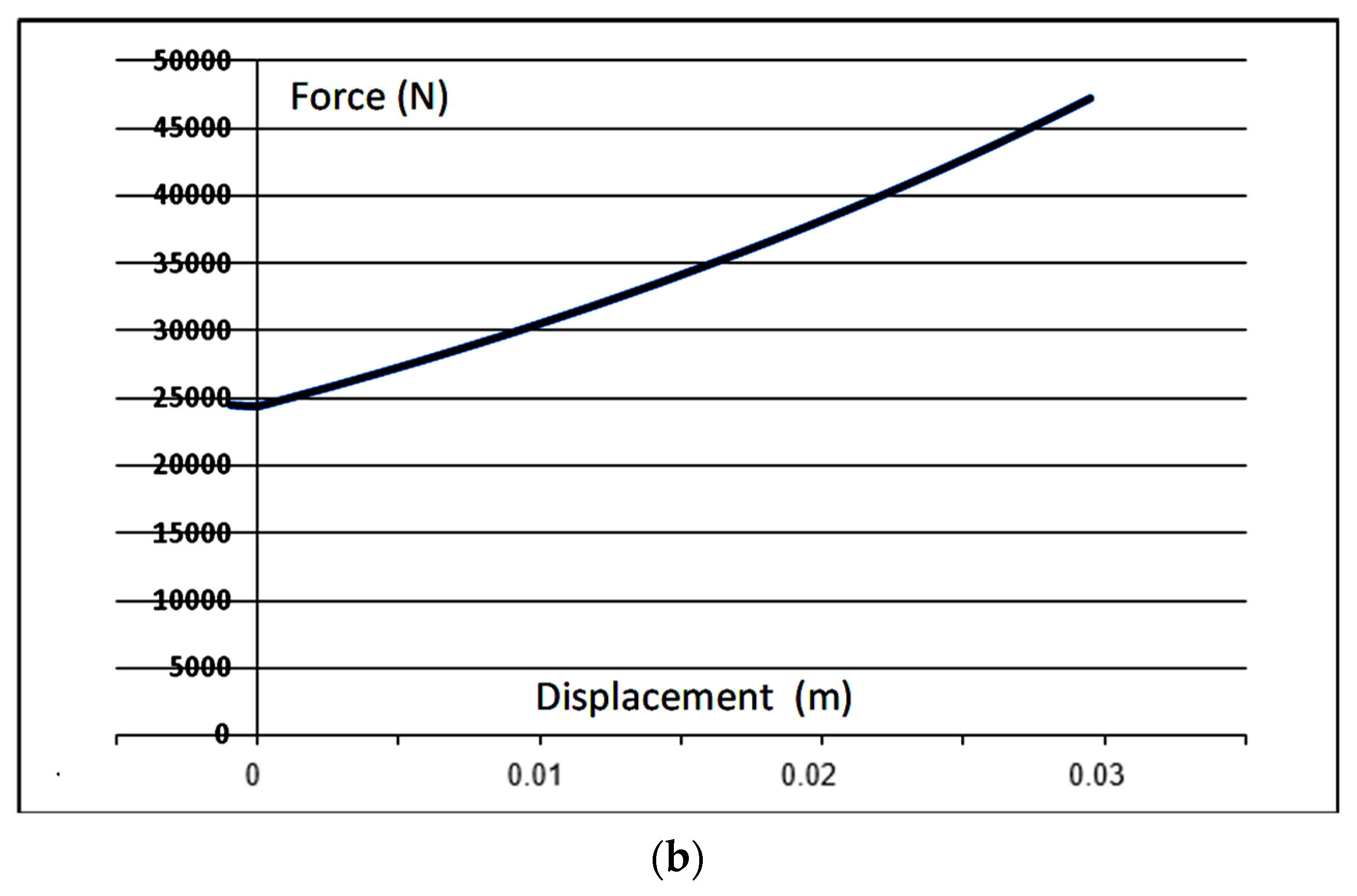
3.3.3. Testing a Bellows Air Spring under Dynamic Load Using a Pseudo-Dynamic Procedure
- Torus radius r = 50 mm
- Radius of circle passing through the transverse sections radius: R = 200 mm
- Initial pressure (preload pressure) P0 = 2 bar (≅0.2 MPa)
- External force F(t) = 10 KN (suddenly applied as a step load)
- Virtual mass of the SDOF system M = 50 Kg
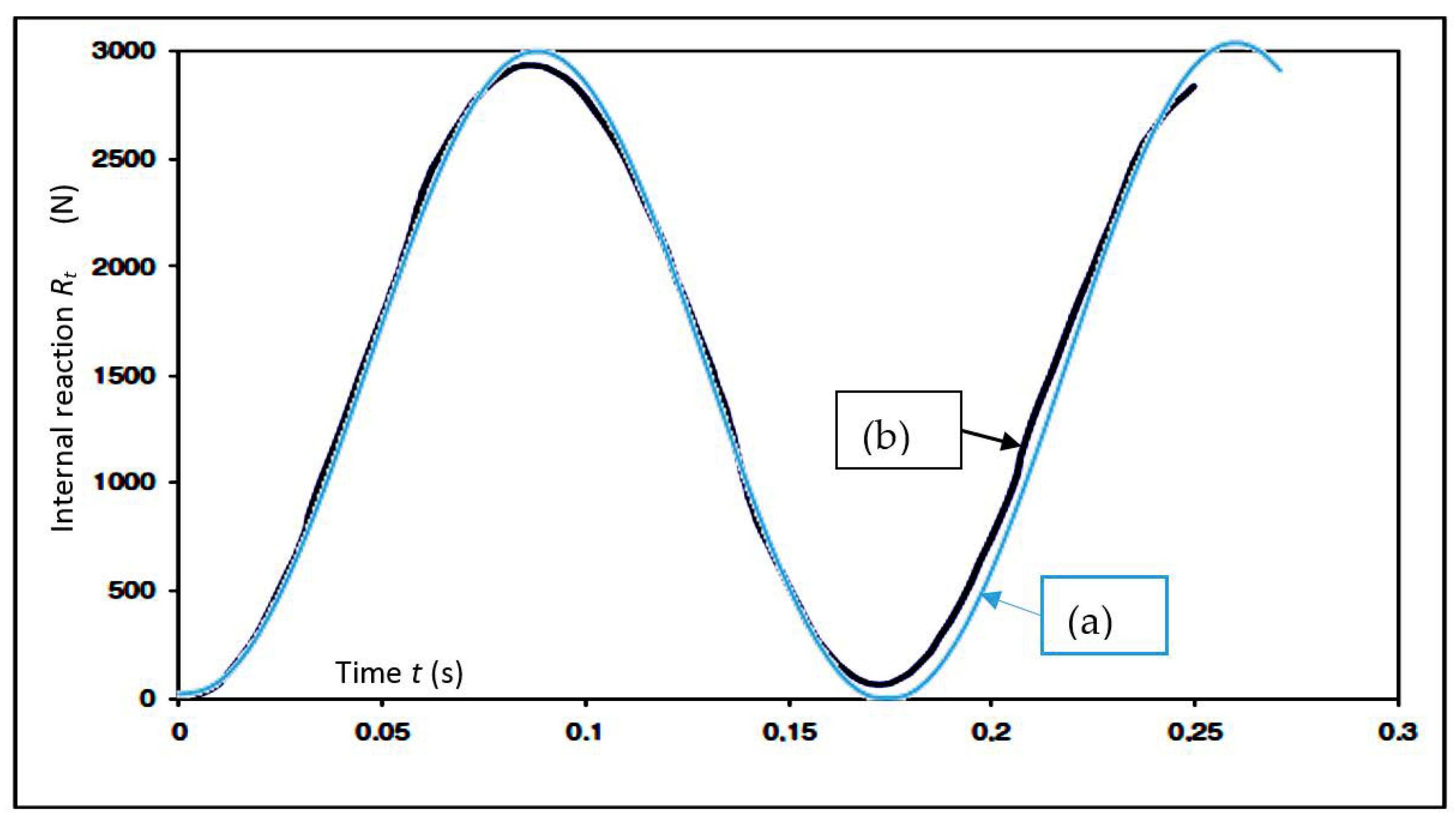
3.4. Pseudo-Dynamic Test of a Real Pneumatic Spring: Procedures and Results
- A pneumatic double-bellows type spring (Numatics-Emerson®) having a rigid end flanges diameter of 160 mm. Internal pressure is p0 = 4 bar;
- A virtual mass M = 100 Kg (no damping considered);
- An impulsive step force F(t) = 1500 N sustained after instant t = 0+ s;
- No damping was considered in the dynamic model.
4. Conclusions
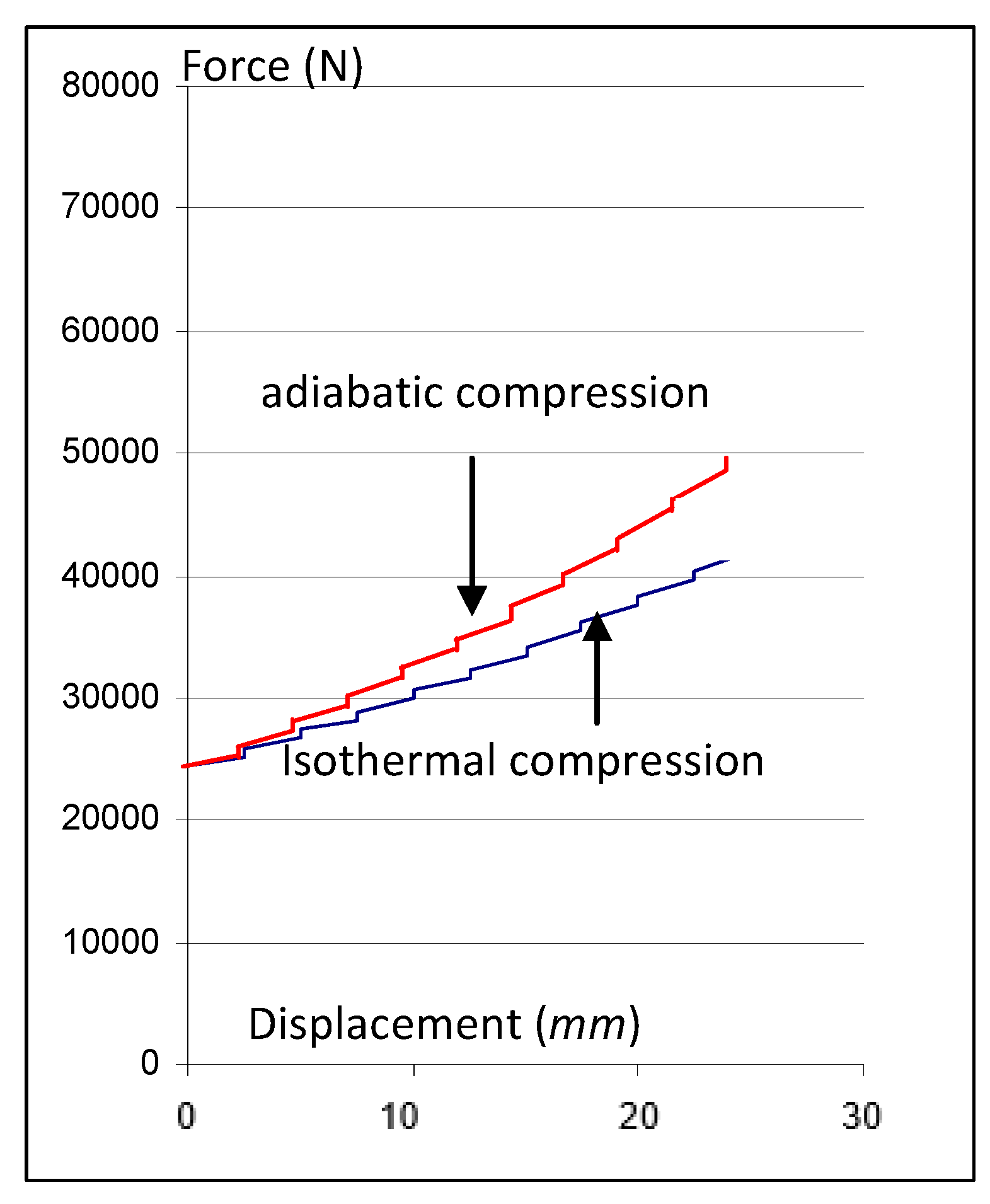
- A simple yet accurate shell model was developed by variational techniques based on the minimum deformation energy concept and essential thermodynamics of gases. For enhanced accuracy, the influence of the velocity of the deformation was included by selecting isothermal or adiabatic compression regimes for the air suspension.
- The present model predictions agreed very well with a finite element model constructed in a commercial code with axisymmetric high order shell finite elements.
Author Contributions
Funding
Conflicts of Interest
References
- Humphreys, W. Pneumatic Spring for Vehicles. U.S. Patent 673,682, 22 January 1901. [Google Scholar]
- Sainsbury, H. Air Suspension for Road Vehicles. Proc. Inst. Mech. Eng. Automob. Div. 1957, 11, 75–101. [Google Scholar] [CrossRef]
- Quaglia, J.; Sorli, M. Air Suspension Dimensionless Analysis and Design Procedure. Veh. Syst. Dyn. 2010, 35, 443–475. [Google Scholar] [CrossRef]
- Löcken, F.; Welsch, M. The Dynamic Characteristic and Hysteresis Effect of an Air Spring. Int. J. Appl. Mech. Eng. 2015, 20, 127–145. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Y.; Huang, H.; Chen, R.; He, M. Studies on Modeling a Single-Bellows Air Spring and Simulating its Inherent Characteristics. In Proceedings of the 5th International Conference on Information Engineering for Mechanics and Materials, Huhhot, China, 25–26 July 2015. [Google Scholar] [CrossRef]
- Apostol, M. Calculus Volume II, 2nd ed.; John Wiley & Sons: New York, NY, USA, 1969; pp. 312–323. ISBN 0-471-00007-8. [Google Scholar]
- Ugural, A. Stresses in Plates and Shells, 2nd ed.; McGraw-Hill Co.: New York, NY, USA, 1999; pp. 211–236. ISBN 0070657696. [Google Scholar]
- Reddy, J. An Introduction to Non-Linear Finite Element Analysis, 2nd ed.; Oxford University Press: New York, NY, USA, 2004; pp. 430–447. ISBN 9780199641758. [Google Scholar]
- Newmark, M. A method of computation for structural dynamics. ASCE J. Eng. Mech. Div. 1959, 85, 67–94. [Google Scholar]
- Pinto, A.; Verzeletti, G.; Molina, J.; Varum, H.; Pinho, R.; Coelho, E. Pseudo-Dynamic Tests of Non-Seismic Resisting RC Frames (Bare and Selective Retrofit Frames); JRC-EUR 20244 EN Report; Joint Research Centre: Ispra, Italy, 2002. [Google Scholar]
- Pinto, A.; Pegon, P.; Magonette, G.; Tsionis, G. Pseudo-dynamic testing of bridges using non-linear substructuring, Earthquake Engineering Structural Dynamics. J. Int. Assoc. Earthq. Eng. 2004, 33, 1125–1186. [Google Scholar] [CrossRef]
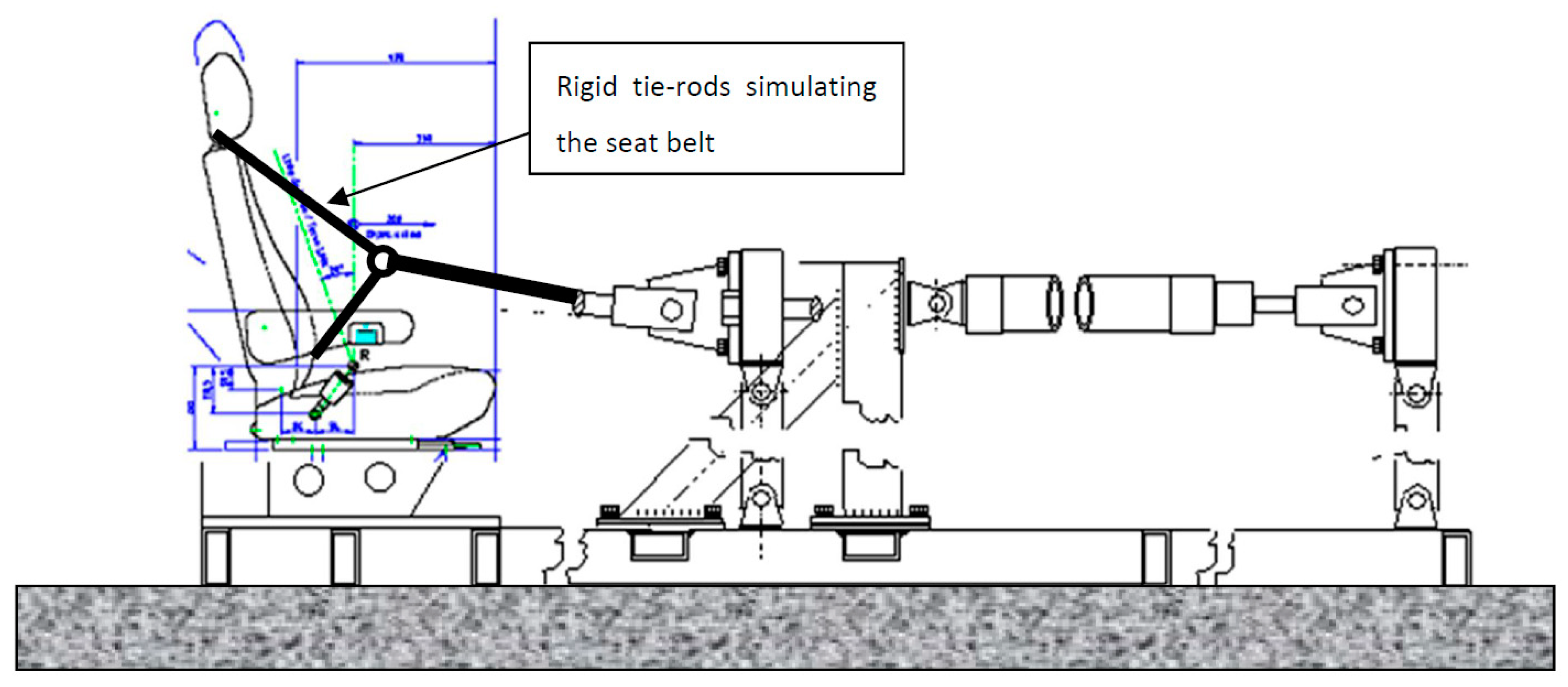
- Carneiro, J.; Melo, F.; Pereira, J.; Teixeira, V. Pseudo-Dynamic Method for Structural Analysis of Automobile Seats. Proc. Inst. Mech. Eng. Part K J. Multi-Body Dyn. 2005, 219, 337–344. [Google Scholar] [CrossRef] [Green Version]
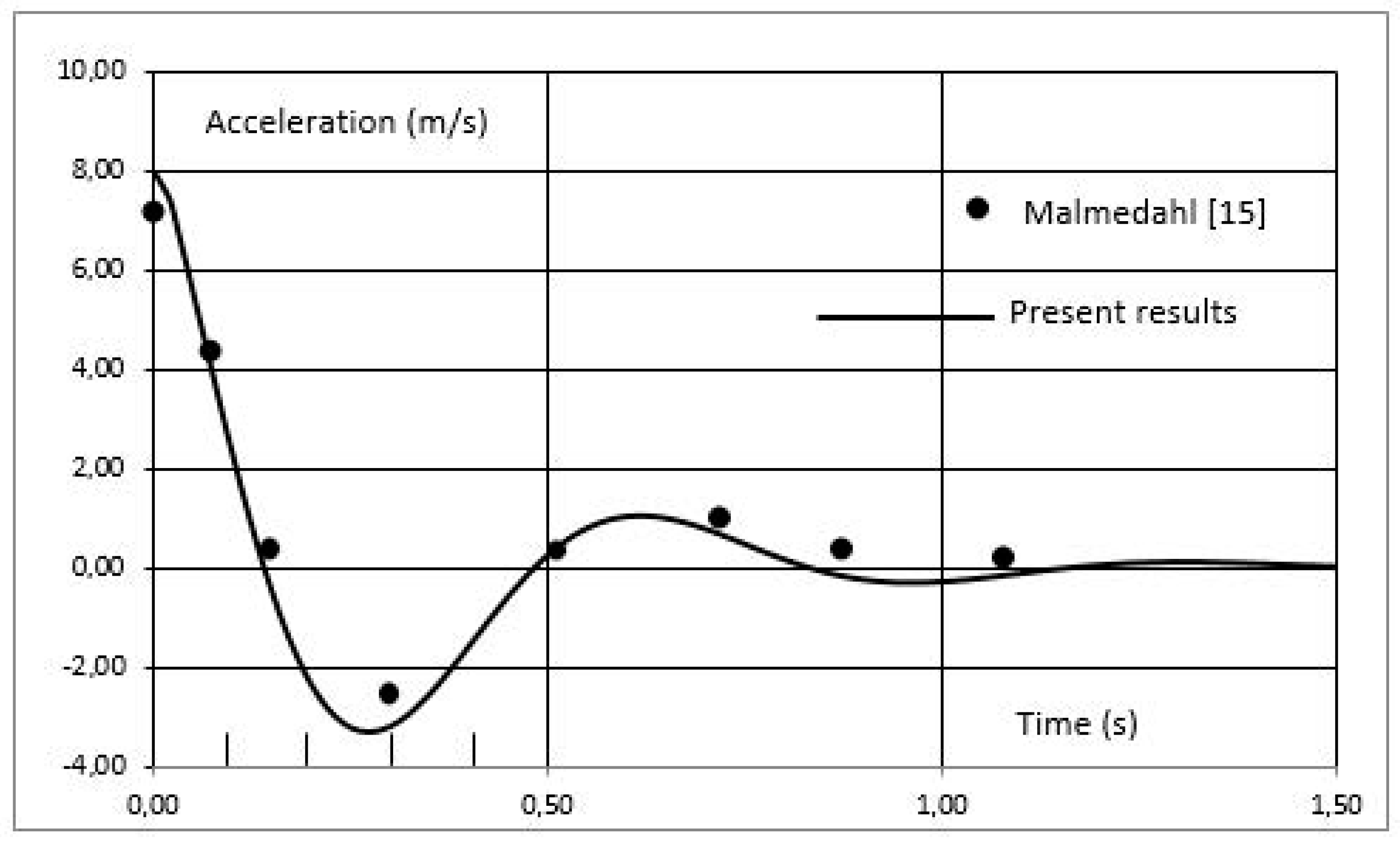
- Malmedahl, G. Analysis of Automotive Damper Data and Design of a Portable Measurement System. Bachelor’s Thesis, Department of Mechanical Engineering, Center for Automotive Research, The Ohio State University, Columbus, OH, USA, December 2005. [Google Scholar] [Green Version]



© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
De Melo, F.J.M.Q.; Pereira, A.B.; Morais, A.B. The Simulation of an Automotive Air Spring Suspension Using a Pseudo-Dynamic Procedure. Appl. Sci. 2018, 8, 1049. https://doi.org/10.3390/app8071049
De Melo FJMQ, Pereira AB, Morais AB. The Simulation of an Automotive Air Spring Suspension Using a Pseudo-Dynamic Procedure. Applied Sciences. 2018; 8(7):1049. https://doi.org/10.3390/app8071049
Chicago/Turabian StyleDe Melo, Francisco J. M. Q., António B. Pereira, and Alfredo B. Morais. 2018. "The Simulation of an Automotive Air Spring Suspension Using a Pseudo-Dynamic Procedure" Applied Sciences 8, no. 7: 1049. https://doi.org/10.3390/app8071049




