A Self-Consistent Physical Model of the Bubbles in a Gas Solid Two-Phase Flow
Abstract
:1. Introduction
2. Theoretical Model
2.1. PR Equation and the Heat Capacity Ratio of Bubbles
2.2. Gas Velocity Equation in the Gas-Solid Two-Phase Flow System
2.3. Bubble Size and Velocity Equation
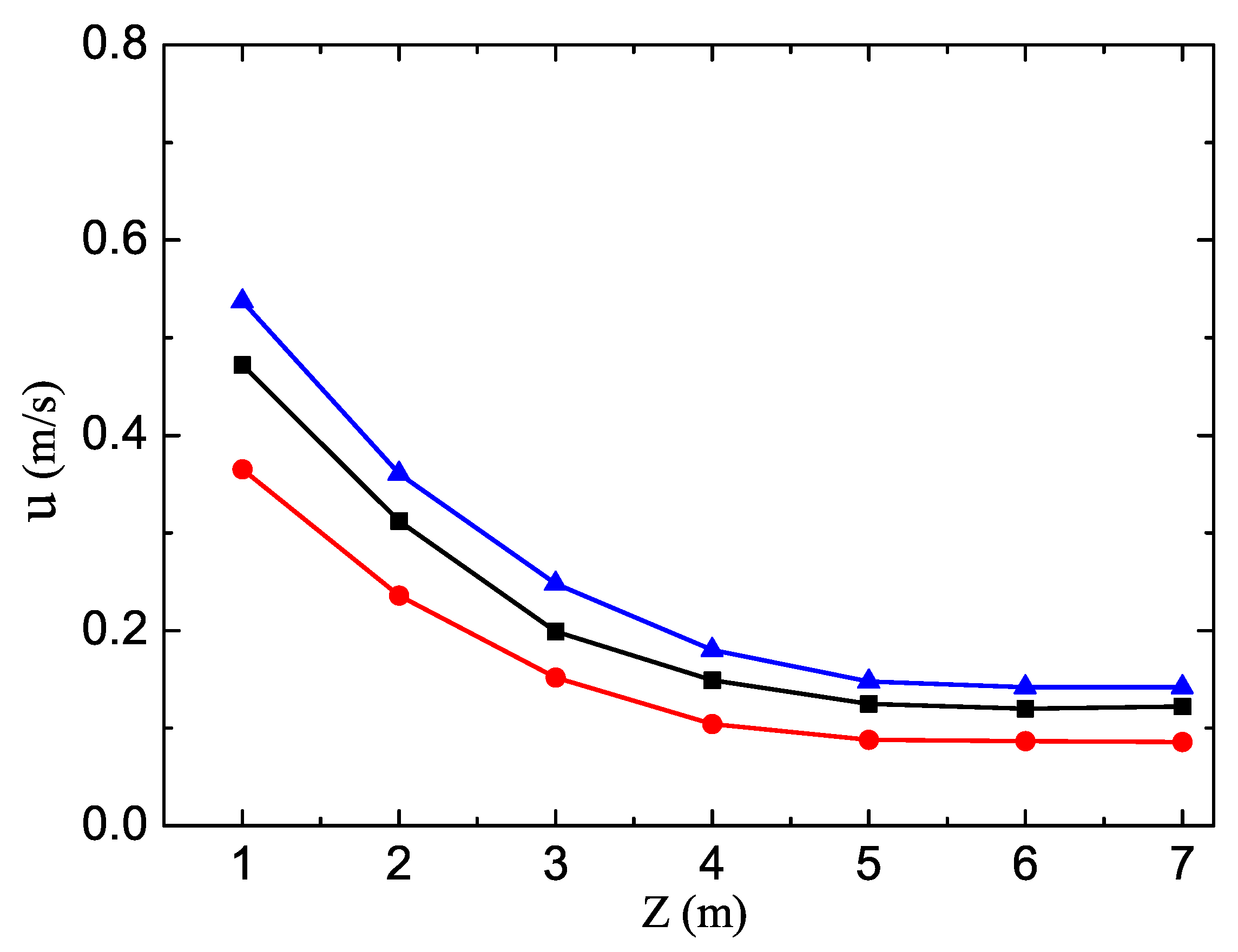
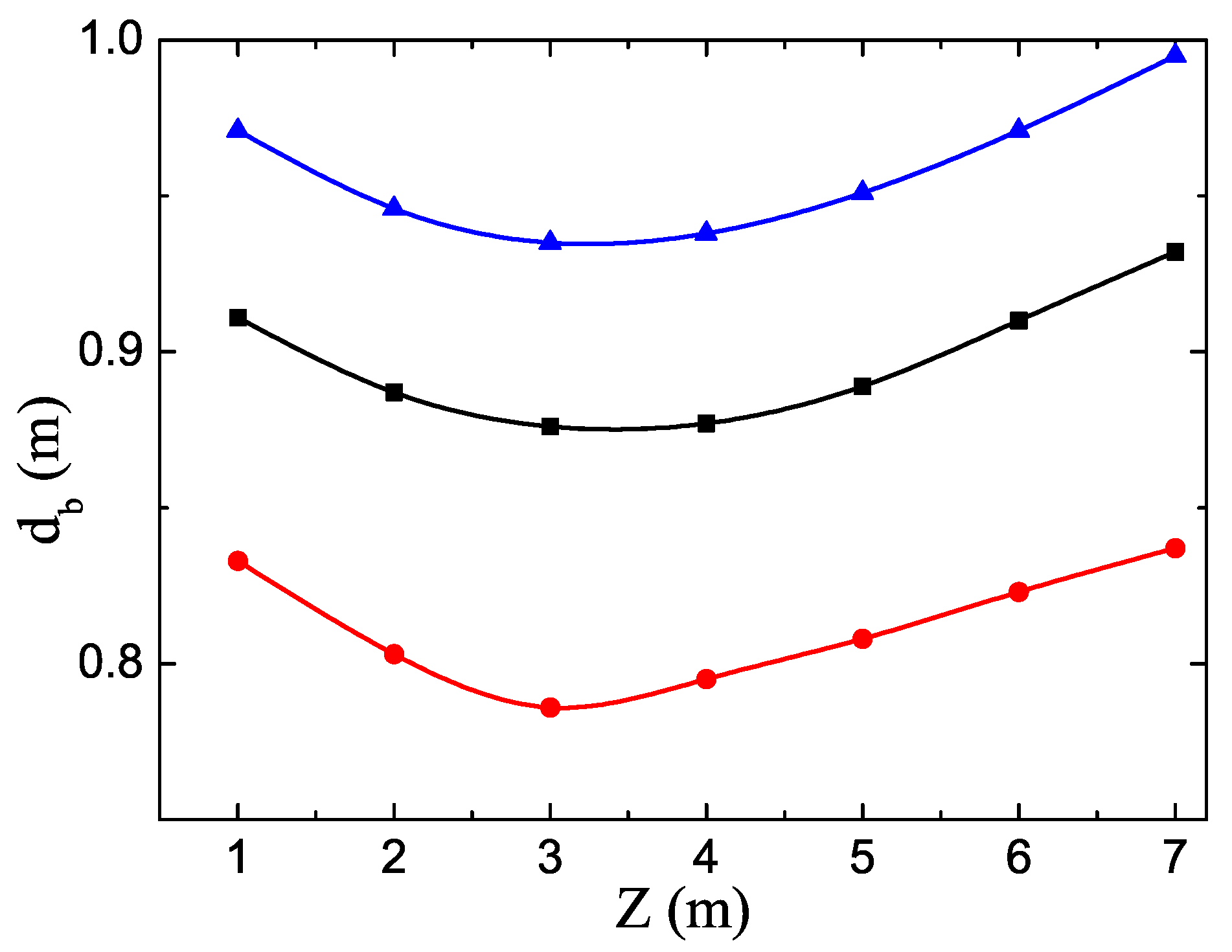
3. Results and Discussion
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Kadanoff Leo, P. Built upon sand: Theoretical ideas inspired by granular flows. Rev. Mod. Phys. 1999, 71, 435. [Google Scholar] [CrossRef]
- Zhao, Y.M.; Duan, C.L.; Wu, L.L.; Zhang, H.J.; He, J.F.; He, Y.Q. The Separation mechanism and application of a tapered diameter Separation Bed. Int. J. Environ. Sci. Technol. 2012, 9, 719–728. [Google Scholar] [CrossRef]
- Yuan, Z.L.; Zhu, L.P.; Geng, F.; Peng, Z.B. Gas-Solid Two Phase Flow and Numerical Simulation; Southeast University Press: Nanjing, China, 2012. [Google Scholar]
- Glasser, B.J.; Sundaresan, S.; Kevrekidis, I.G. From Bubbles to Clusters in Fluidized Beds. Phys. Rev. Lett. 1998, 81, 1849. [Google Scholar] [CrossRef]
- Martyushev, L.M.; Celezneff, V. Nonequilibrium Thermodynamics and Scale Invariance. Entropy 2017, 19, 126. [Google Scholar] [CrossRef]
- Harrision, D.; Leung, L.S. Bubble Formation at an Orifice in a Fluidized Bed. Nature 1961, 190, 433–434. [Google Scholar] [CrossRef]
- Pak, H.K.; Behringer, R.P. Bubbling in vertically vibrated granular materials. Nature 1994, 371, 231–233. [Google Scholar] [CrossRef]
- Xiong, Q.; Lia, B.; Zhou, G.; Fang, X.; Xu, J.; Wang, J.; He, X.; Wang, X.; Wang, L.; Ge, W.; et al. Large-scale DNS of gas–solid flows on Mole-8.5. Chem. Eng. Sci. 2012, 71, 422–430. [Google Scholar] [CrossRef]
- Van Lare, C.E.J.; Piepers, H.W.; Sehoonderbeek, J.N.; Schoonderbeek, J.N.; Thoenes, D. Investigation on bubble characteristics in a gas fluidized bed. Chem. Eng. Sci. 1997, 52, 829–841. [Google Scholar] [CrossRef]
- Ichiki, K.; Hayakawa, H. Dynamical simulation of fluidized beds: Hydrodynamically interacting granular particles. Phys. Rev. E 1995, 52, 658. [Google Scholar] [CrossRef]
- Farshi, A.; Javaherizaden, H.; Hamzavi Abedi, M.A. An investigation of the effect of bubble diameter on the performance of gas-solid fluidized bed reactor and two-phase modeling of bubbling fluidized bed reactor in melamine production. Pet. Coal 2008, 50, 11–22. [Google Scholar]
- Valverde, J.M.; Castellanos, A.; Mills, P.; Quintanilla, M.A.S. Effect of particle size and interparticle force on the fluidization behavior of gas-fluidized beds. Phys. Rev. E 2003, 67, 051305. [Google Scholar] [CrossRef] [PubMed]
- Müller, C.R.; Davidson, J.F.; Dennis, J.S.; Fennell, P.S.; Gladden, L.F.; Hayhurst, A.N.; Mantle, M.D.; Rees, A.C.; Sederman, A.J. Real-Time Measurement of Bubbling Phenomena in a Three-Dimensional Gas-Fluidized Bed Using Ultrafast Magnetic Resonance Imaging. Phys. Rev. Lett. 2006, 96, 154504. [Google Scholar]
- Homsy, G.M. Nonlinear Waves and the Origin of Bubbles in Fluidized Beds. Appl. Sci. Res. 1998, 58, 251–274. [Google Scholar] [CrossRef]
- Tafreshi, Z.M.; Kingsley, O.G.; Adesoji, A.A. A two-phase model for variable-density fluidized bed reactors with generalized nonlinear kinetics. Can. J. Chem. Eng. 2000, 78, 815–826. [Google Scholar] [CrossRef]
- Komatsu, T.S.; Hayakawa, H. Nonlinear waves in fluidized beds. Phys. Lett. A 1993, 183, 56–62. [Google Scholar] [CrossRef]
- Choi, J.H.; Son, J.E.; Kim, S.D. Bubble size and frequency in gas fluidized beds. J. Chem. Eng. Jpn. 1988, 21, 171–178. [Google Scholar] [CrossRef]
- Al-Zahrani, A.A.; Daous, M.A. Bed expansion and average bubble rise velocity in a gas-solid fluidized bed. Powder Technol. 1996, 87, 255–257. [Google Scholar] [CrossRef]
- Best, J.P. The formation of toroidal bubbles upon the collapse of transient cavities. J. Fluid Mech. 1993, 251, 79–107. [Google Scholar] [CrossRef]
- Tian, J.X.; Jiang, H.; Guic, Y.X.; Mulerod, A. Equation of state for hard-sphere fluids offering accurate virial coefficients. Phys. Chem. Chem. Phys. 2009, 11, 11213–11218. [Google Scholar] [CrossRef] [PubMed]
- Martín, C.; Jørgen, M. Development and application of a three-parameter RK–PR equation of state. Fluid Phase Equilibria 2005, 232, 74–89. [Google Scholar]
- Gasem, K.A.M.; Gao, W.; Pan, Z.; Robinson, R.L., Jr. A modified temperature dependence for the Peng–Robinson equation of state. Fluid Phase Equilibria 2001, 181, 113–125. [Google Scholar] [CrossRef]
- Vandermeulen, J.; Leliaert, J.; Dupre, L.; Van Waeyenberge, B. Field-driven chiral bubble dynamics analysed by a semi-analytical approach. J. Phys. D 2017, 50. [Google Scholar] [CrossRef]
- Wei, L.P.; Lu, Y.J. Bubble dynamic wave velocity in fluidized bed. Chem. Eng. Sci. 2016, 147, 21–29. [Google Scholar] [CrossRef]
- Syamlal, M.; O’Brien, T.J. Fluid dynamic simulation of O3 decomposition in a bubbling fluidized bed. AICHE J. 2003, 49, 2793–2801. [Google Scholar] [CrossRef]
- Sau, D.C.; Mohanty, S.; Biswal, K.C. Prediction of critical fluidization velocity and maximum bed pressure drop for binary mixture of regular particles in gas–solid tapered fluidized beds. Chem. Eng. Process. 2008, 47, 2114–2120. [Google Scholar] [CrossRef]
- Zhang, C.X.; Qian, W.Z.; Wei, F. Instability of uniform fluidization. Chem. Eng. Sci. 2017, 173, 187–195. [Google Scholar] [CrossRef]
- Darton, R.C.; Lanauze, R.D.; Davidson, J.F.; Harrison, D. Bubble Growth due to Coalescence in Fluidized Beds. Trans. Inst. Chem. Eng. 1977, 55, 274–280. [Google Scholar]
- Zhang, C.X.; Li, P.L.; Lei, C.; Qian, W.Z.; Wei, F. Experimental study of non-uniform bubble growth in deep fluidized beds. Chem. Eng. Sci. 2018, 176, 515–523. [Google Scholar] [CrossRef]
- Chen, L.M.; Yang, X.G.; Li, G.; Yang, J.; Wen, C.H.; Li, X.; Snape, C. Dynamic modelling of fluidisation in gas-solid bubbling fluidised beds. Powder Technol. 2017, 322, 461–470. [Google Scholar] [CrossRef]



© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dong, H.; He, J.; Duan, C.; Zhao, Y. A Self-Consistent Physical Model of the Bubbles in a Gas Solid Two-Phase Flow. Appl. Sci. 2018, 8, 360. https://doi.org/10.3390/app8030360
Dong H, He J, Duan C, Zhao Y. A Self-Consistent Physical Model of the Bubbles in a Gas Solid Two-Phase Flow. Applied Sciences. 2018; 8(3):360. https://doi.org/10.3390/app8030360
Chicago/Turabian StyleDong, Haiming, Jingfeng He, Chenlong Duan, and Yuemin Zhao. 2018. "A Self-Consistent Physical Model of the Bubbles in a Gas Solid Two-Phase Flow" Applied Sciences 8, no. 3: 360. https://doi.org/10.3390/app8030360





