1. Introduction
The emergence and persistence of cooperation is a classical issue in natural and social sciences. This question is often analyzed in game theoretical situations like the Prisoner’s Dilemma or the Public Good provision game, reflecting the conflict between individual interest, collective rationality and altruism. The biological, economic and game theoretical literatures highlight various routes to sustain cooperation in communities. A first perspective takes an evolutionary viewpoint and considers the evolution of altruistic traits or cooperative preferences subject to some genetic or cultural selection forces ([
1,
2]). A second approach assumes the existence of specific institutions or social mechanisms, such as reputation building in repeated relationships or segregation in social interactions (assortative matching), that incentivize or protect cooperation in a community ([
3,
4,
5]). Another strand of the literature highlights the importance of space and the diffusion process of cultural dimensions that help sustain modes of cooperation in various social contexts (see for instance [
6], and more recently [
7,
8]). Interesting historical examples related to this perspective are the spatial neolithic transition from hunting-gathering into farming and stockbreeding in Europe with its emphasis both on demic diffusion and cultural diffusion
1 (see [
11,
12]), the rise and decline of merchant guilds as organizations promoting exchanges and cooperation among traders in different parts of medieval Europe (see [
13,
14,
15,
16,
17]), or recently, the diffusion of a “sharing culture” through which local communities come up with creative initiatives, based on sharing and collaboration, to serve their daily needs with economic alternatives to traditional service provision (private or public) ([
18]).
The purpose of this paper is to contribute to this line of research by analyzing how preferences evolution and institution building can jointly evolve and interact to sustain cooperation in a spatial context. Specifically, we consider the spatial transmission and diffusion of a cultural trait for cooperation in a context where a local institution of social segregation (assortative matching) can be endogenously decided by some political collective decision making mechanism. To do this, we develop a continuous space framework in which preferences are locally transmitted across generations through a cultural transmission process à la Bisin-Verdier (Bisin and Verdier 2001, [
19]), and also diffuse randomly in space due to some spatial noise in socialization. Additionally, following [
3,
5], we introduce the presence of an assortative matching institution by which cooperators can partially segregate themselves for their social interactions. In contrast to the previous literature however, we assume that the degree of assortativity and extent by which matching between cooperators is biased, is endogenous, costly to implement, and decided collectively at the local community level. We describe this in the sequel as the degree of institutional assortativity that prevails at the community level.
In such a context, we analyse the spatial co-evolution of the cultural trait of cooperation and the local institution of assortative matching that facilitates the expression of this culture. Specifically, the questions we address are the followings: how do preferences for cooperation evolve in time and space? When is it that cooperative preferences initially located in a limited region give rise to a cultural diffusion wave that successfully invades the whole population in space? Under which conditions will such phenomenon happen? What is the equilibrium spatial and time co-evolution of a culture of cooperation and the (endogenous) local institutions that promote such culture?
The paper provides the following contributions. First on the technical side, and in contrast to agent-based simulations models analyzing cooperative behaviors in discrete spatial environments such as lattice or networks frameworks ([
8] and others), we approach the problem of spatial diffusion of cooperation in a continuum space, applying for this purpose mathematical techniques and results from partial differential reaction-diffusion equations theory. This perspective, relatively new to economists and social scientists, is useful as it allows the derivation of specific analytical results on the spatial evolution of cooperation and the assortative matching institutions that interact with these cultural patterns.
Starting with the benchmark case of exogenous assortative matching institutions as described in [
5], or [
3] in a non spatial context, we show that for a degree of institutional assortativity above a certain threshold, the existence of a spatially localized cultural cluster of cooperators may allow the diffusion of a culture of cooperation to the whole spatial population on an infinite domain. More precisely, we are able to provide analytical conditions for the existence of a positive
traveling wave of a culture of cooperation that invades the whole population. In this case, we characterize the asymptotic speed of diffusion, and show that it is increasing in some crucial fundamentals of the problem: the degree of institutional assortativity and the intensity of the taste for cooperation in the preference structure.
We then extend the framework to the case of endogenous and costly assortative institutions implemented at the local community level, and we analyze the interaction between cultural transmission and the emergence of these matching institutions along the spatial process of diffusion of a culture of cooperation. We show how relatively low cost assortative matching institutions can appear in new places thanks to the spatial random diffusion of cooperation, and in turn facilitate a localized cluster of cooperative preferences to invade the whole population. Again, we provide conditions for the existence of a traveling wave of a culture of cooperation. We also study the resulting spatial pattern of institutional evolution. We show that the equilibrium degree of assortativity follows a non monotonic path along the diffusion process in space. Places with initially no cooperators and no local institution of social segmentation, thanks to the diffusion process, will be the locus of emergence of an assortative matching mechanism, first growing and then disappearing once cooperators have reached a sufficient proportion of the population inside the local community. This highlights an interesting example in which culture and institutions act initially as dynamic complements and then as dynamic substitutes along the diffusion process.
Finally, while our analytical results are provided for diffusion processes on infinite domains, we provide in the appendix numerical simulations to show that the main qualitative insights derived analytically persist on finite domains.
Our paper connects directly to the large literature about cooperation evolving on a discrete space structure, i.e., lattices or networks (see Eshel, Samuelson and Shaked (1998), [
8,
20,
21])
2. The main result in that literature is that a structured population with interactions limited to neighbors often sustains the long run existence of local clusters of cooperation (and even invasion over the full structure) under conditions where, without space, one would not expect cooperation to survive from an evolutionary perspective. Our approach differs from the previous ones along two dimensions. First, while in the previous models cooperators’ movement was directed and purposeful, we assume here that cultural traits diffuse locally with some random direction in space due to a spatial random component in the socialization mechanism associated to cultural transmission. Second, this literature mainly investigates the properties of the discrete spatial dimension through simulations. Here we consider a continuum spatial structure that allows us to obtain some general analytical results.
The mathematical biology literature has also applied some spatial models with a continuum space to economic games of cooperation (see for instance [
7,
23]). However, our paper differs from these in two ways. First, we consider cultural evolution based on intergenerational socialization instead of biological evolution based on fitness. Second and more importantly, we study the interaction between cultural diffusion and social institutional building, by allowing for the possibility of the emergence of an endogenous assortative matching institution set at the local level, according to the proportion of cooperators and defectors weighted by their “political power ” in the community. The analysis then exhibits the existence of dynamic complementarities and substituabilities between preferences and institutions spatially, and it generates an example of the endogenous “rise-and-fall” of “cooperation supporting” institutions following a diffusion “wave”pattern over time and space.
Our work connects as well to recent economic papers which discuss the links between the emergence of cooperation and the existence of segregation in interactions. Reference [
24] consider for instance the interplay between assortativity in actions and homophily as induced by cultural intolerance. They show that it induces states where there is perfect correlation between culture and behavior with all agents from one cultural group cooperating, and all agents from the other cultural group defecting. In contrast to this work, in our framework, cultural types are not fixed and evolve along a cultural transmission process both across generations and across space. In a similar vein, Reference [
25] propose a model of local interactions with endogenous network formation, and show how cooperators and defectors endogenously cluster themselves in separated components, supporting cooperation and segregation altogether. Our framework differs in two ways. First, as already said, we consider a continuum space structure as well as an endogenous intergenerational cultural transmission process. Second, segregation comes in our work from a centralized collective action problem at the community level, rather than as a random opportunity to break or create a link for some local interaction.
Closer to our work is the recent paper [
26] which investigates how assortativity, jointly with cooperation, can be driven by evolution. The authors consider an evolutionary game theoretical framework in which assortativity evolves by `democratic consensus’, and show that full-or-null assortativities turn out to be long-run stable in most cases. In our framework, assortativity is also determined by some collective decision making process. In contrast we introduce an explicit resource cost for the design of the endogenous degree of assortativity, and investigate how this assortativity evolves over time and space. In particular, we show that along with the diffusion process of a spatial cooperative wave, assortativity follows a non monotonic life cycle evolution at the community level.
Finally, by emphasizing the interaction between the diffusion of a culture of cooperation and the emergence of assortative institutions, we relate to the recent literature on culture and institutions, and in particular the work by [
27]
3 that formally analyzes the joint evolution of culture and institutions in more general institutional and cultural contexts. We complement this piece of work by presenting a specific example that explicitly focuses on the
spatial dimension of the coevolution of culture and institutions. Interestingly, our analysis exhibits as well the existence of dynamic complementarities and substituabilities between preferences and institutions spatially.
The paper is organized as follows.
Section 2 presents the main structure of the model, the interaction game between cooperators and defectors and the cultural transmission mechanism.
Section 3 adds the spatial structure and shows analytically when there exists a traveling wave of a culture of cooperation on an infinite domain.
Section 4 studies the case of endogenous assortative matching institutions. Finally,
Section 5 provides a short conclusion. All the proofs are relegated to the appendix, as well as the numerical simulations.
3. Adding the Spatial Structure
Under the previous simple evolutionary model, the cooperative culture has to exceed a given initial threshold to invade the whole population. How is such conclusion affected when we add a spatial structure? Should the cooperative trait be present over the full space to survive cultural selection? In this section, we provide conditions such that the culture for cooperation needs to exist only on a relatively small domain in order to invade spatially the full population.
Specifically, assume that after the classic Bisin-Verdier socialization mechanism, there is a spatial noisy component of socialization. Children can be socialized to their neighbors’ cultural trait through a random local diffusion of cooperative and defective traits. More precisely, a child at location
x on the real line
could be randomly re-socialized with a cultural trait coming from location
or
, meaning that cultural traits can move locally with random direction in space. While the most relevant papers describing spatial cooperators dynamics usually consider a discrete lattice where agents interact with their neighbors and then replace them with a purposeful movement ([
8]), we differ in the following ways. First, we assume that interactions are strictly local and happen in a community located at
Second, we assume that individuals cannot physically move and, given that each individual has only one offspring, the size of a community located at a point
x is fixed at a constant level
N. Third, after social interaction and reproduction and intergenerational cultural transmission, the new generation (the socialized children) can be randomly resocialized to a neighbors’ trait. Specifically, while individuals cannot move physically, we assume that cultural information moves with random local direction in space causing therefore a spatial noise in the final stage of the socialization mechanism of children. Because of intergenerational cultural transmission and the spatial noise component in the socialization mechanism, the types of individuals in each community will change overtime and space. More precisely, the frequency of cooperators in community
x can be denoted as
, where
is the number of cooperator types at time
t in community
x. Interactions happening at the community level located at
x are then characterized by frequencies
of cooperators and
of non cooperators.
Finally, we consider a continuum spatial structure and an infinite domain () to have analytical and general results. We will then show with some numerical simulations how these results remain generally true in a finite domain.
Using a classical mathematical argument (see [
30], p. 395), one can show that when cultural traits move randomly in a small time interval
on a localized small domain of size
and that one passes to the continuous limit
,
with a constant diffusive assumption that
8 then the local random diffusion process of cultural information can be described through a laplacian operator. The cultural dynamics describing the evolution of the local frequency of cooperators
rewrite therefore as a partial differential equation of the following form
with initial time condition describing the initial spatial frequency of cooperators on the full domain:
with
a real function such that
for all
. Substitution of
and
provides the partial differential equation:
with
and
.
A reaction-diffusion equation similar to (
5) was firstly introduced by R. Fisher (1937) [
31] to describe the wave of advance of an
advantageous gene. The Fisher’s equation could be considered as a spatial extension of the logistic equation and rapidly spread in mathematical biology to describe a wide range of different phenomena. A less known application of Fisher’s equation is the one made by [
32]) who applied this particular reaction-diffusion equation in evolutionary anthropology to study the spread of early farming in Europe. It is clear that this mathematical representation simplifies the diffusion process making it continuous in time and space. However, as stated by [
32], even when real diffusion is discontinuous, it often operates over short intervals of space and time. A continuous temporal and spatial diffusion theory may then still provide useful insights on the nature of the spatial cultural dynamics.
To describe the diffusion process of the cooperative culture in a spatial context, it is of specific interest to analyze solutions of (
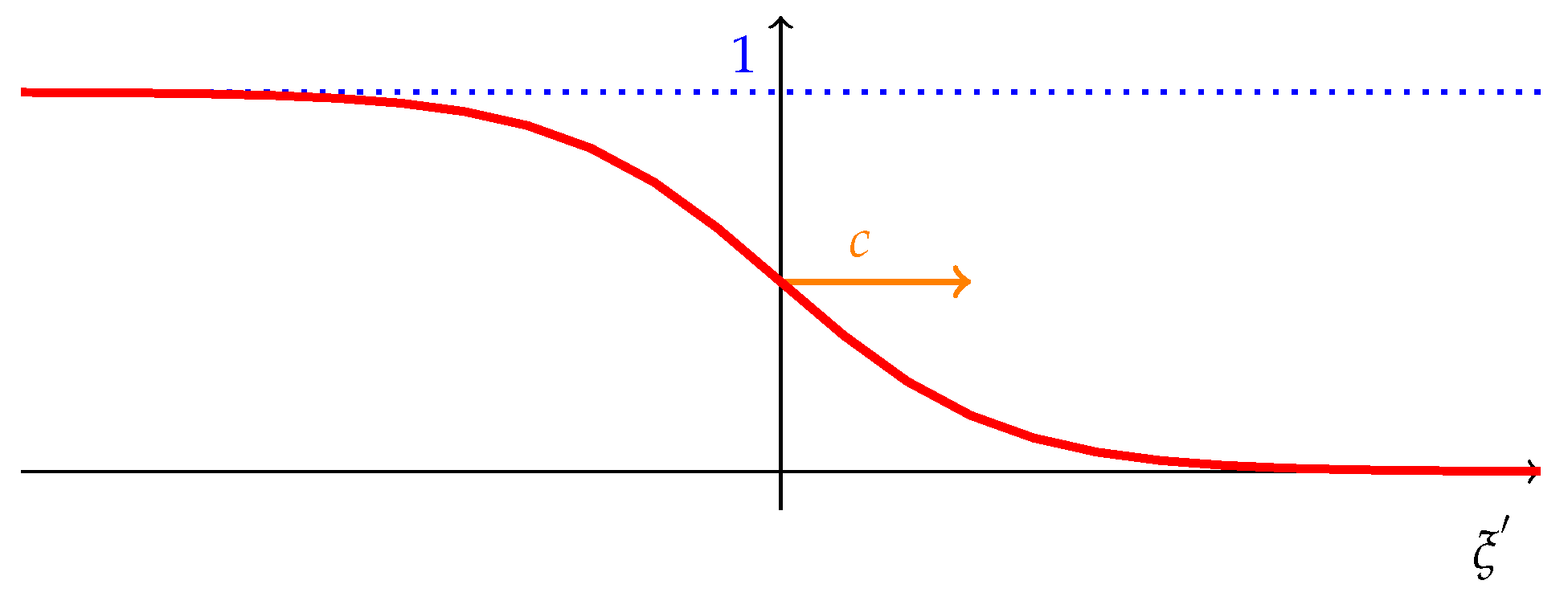
5) in the form of a traveling wave. A traveling wave is a particular type of solution on an infinite domain obtained by a change of variables such that it connects the two homogeneous steady states (here 0 and 1) and travels without change of shape. Specifically, if
represents a traveling wave, the shape of the solution will be the same for all time and the speed of propagation of this shape is a constant usually denoted by
c. If we look at this wave in a traveling frame moving at speed
c it will appear stationary. The traveling wave is therefore a tractable theoretical tool to describe how a diffusive phenomenon happens in time and space (see the Mathematical Appendix for the exact definition).
In the case of system (
5), we can apply a classical partial differential equations’ theorem (see the theorem in the Appendix) that ensures the existence of a unique traveling wave that moves with speed
c, whose sign is given by the sign of the integral
with
Furthermore, using a classical result (see [
33], p. 234) for reaction-diffusion equations of the following form
we can compute the exact speed and the profile function
of the traveling wave
. In particular, the speed is given by
and the function
has the following shape:
where
. Because we use
as the traveling wave coordinate, a solution with
corresponds to a wave moving from right to left on the spatial domain
. We could equally well have used
as the traveling wave coordinate, to obtain waves moving from left to right for
positive c (this is actually the choice we made in
Figure 1).
In the context of our Equation (
5), we obtain the speed of propagation
We can now state the following result.
Proposition 2. Under the hypothesis (4) there exists such that for a localized set of cooperators can invade the whole space, i.e., there exists a traveling wave that moves with positive speed The value of the cooperation diffusion speed c is an increasing function of ρ and δ. Conversely for , a localized set of non cooperators can invade the whole space, i.e., there exists a traveling wave that moves with negative speed The absolute value of the non cooperation diffusion speed is a decreasing function of ρ and δ. Proposition 2 provides a simple characterization of the sign of the diffusion speed
c of the traveling wave. When this speed is positive, it implies that starting from an initial configuration where there are just noncooperative preferences, the appearance of a small cluster of a culture of cooperation with enough assortative matching and whose proportion is larger than
is enough for cooperation to invade the whole space.
9 Conversely, when the diffusion speed
c is negative, a small cluster of non cooperative preferences (in a proportion larger than
, can fully invade an initial spatial configuration of cooperative preferences, provided that there is not too much assortative matching.
In the simple non spatial model of
Section 2, cooperative preferences could only diffuse in the full population if their frequency initially exceeded the threshold
. With a local random spatial component of socialization, the requirement for the spatial diffusion of the culture of cooperation is weaker. Cooperative preferences still need to exceed
at some point of space. However, to invade the full population, it is now sufficient to have this condition satisfied on a small localized domain. In other words, even with an initial “average”lower than
in space, cooperative preferences can still take advantage of their local success to take over the whole population (see the numerical simulations on finite domains for an intuitive idea).
Inspection of (
8) also shows that the speed of propagation of cooperation is an increasing function of the degree of segregation
and of the intensity of preference for cooperation
. Furthermore, looking at the Expression (
7) and the value for
D, we can easily observe that the higher is
G the steeper is the profile of the traveling wave (the “jump” between 0 and 1) while
has the opposite effect, the higher the segregation the smoother becomes the profile of the wave.
10The previous results have been proved analytically for infinite spatial domains since on finite domains the notion of traveling wave has no meaning. However, if the domain is finite but large enough, the solution of the partial differential reaction-diffusion Equation (
12) qualitatively follows for some time the profile of a traveling wave. In the appendix we present and discuss a Matlab simulation to analyze how the spatial diffusion process will happen on a finite one dimensional domain and how the invasion fronts resemble to the profile of a traveling wave. We can easily observe (see the
Appendix D) that, given an initial datum, the smaller is
the faster is the invasion.Furthermore, the profile of the solution resembles to the profile of the traveling wave we characterized for an infinite domain.
4. Diffusion of Cooperation and Matching Institutions
In the previous section we have shown that a high enough degree of social segmentation and a random spatial process of socialization induce a diffusion mechanism that allows the invasion of cooperative preferences in the whole space, even when such preferences are initially localized only in a small domain. In this section, we analyze the interaction of the process of spatial diffusion of preferences with the emergence of assortative matching institutions promoting cooperation at the local level.
4.1. Endogenous Assortative Matching Institutions
To do this, we consider the case where the degree of assortativity
is endogenous and chosen by a collective decision mechanism at the local level. The parameter
allows cooperators to protect themselves from exploitation by defectors. Clearly since it shapes social interactions inside the community, the choice of
can be a source of conflict between cooperators and defectors as it favors the former and penalizes the latter. Presumably, the outcome of such collective decision problem will depend on the relative political strength of the two groups. We highlight these features in the simplest way by assuming that at each point of time,
is determined at the community level by the maximization of a social welfare function reflecting the sum of the utilities of the two groups of individuals, weighted by their political power, that we simply assume to be proportional to their population size in the community.
11Specifically, we consider first the non spatial case with a given community characterized by a frequency
q of cooperators. The community selects the institutional assortativity parameter
by the following social choice maximization program:
where
represents the cost of the endogenous institution that is borne by the whole community
12. We assume that the cost function is increasing and convex, (i.e.,
), with the Inada conditions
and
. Moreover we assume that
for technical reasons.
Substituting the expressions of
and
in (
9) and using the hypothesis
we find that the optimal degree of assortativity
is given by:
Inspection of (
10) shows immediately that the optimal degree of assortative matching is a non monotonic function of the frequency
q of cooperators in the community. The intuition for this is the following. Cooperators are in favor of social segmentation while defectors are against it. A first effect is associated to the negative intensive margin effect of
q on the marginal benefit of segmentation for cooperators. The marginal benefit of segmentation for each cooperator writes indeed as
and it declines with the frequency of cooperators
As the population becomes more homogeneous in terms of cooperative individuals, random matching provides naturally more encounters between cooperators and the payoff benefit for a cooperator to separate himself from defectors gets smaller.
At the same time, there is also a positive extensive effect of due to the fact that when the cooperative group increases in size, it has more political influence in the collective choice problem determining the optimal value of segregation. The aggregate effect of q on is therefore non monotonic, increasing for low values of q and decreasing for high values of q.
In a symmetric way, the aggregate marginal gain of segmentation for defectors
is negative and also a non monotonic function of
q. Because of this, it is clear that defectors do not have any incentive to invest in
. Combining both, the aggregate weighted social welfare benefit of segmentation is then simply
and it is also non monotonic in
reflecting the conflicting extensive and intensive margins effects for the two groups.
In a non spatial context, the dynamics of cooperators writes as:
Observing that
we assume that
, or
, so that we can study the case in which there exists just one interior equilibrium. Note also that
We can prove that indeed
(see the
Appendix B.3).
Substituting the optimal value of community segregation
, the dynamics can thus finally be rewritten as
The following lemma, whose proof is relegated to the appendix, characterizes the cultural steady states of the non spatial dynamics with endogenous segmentation:
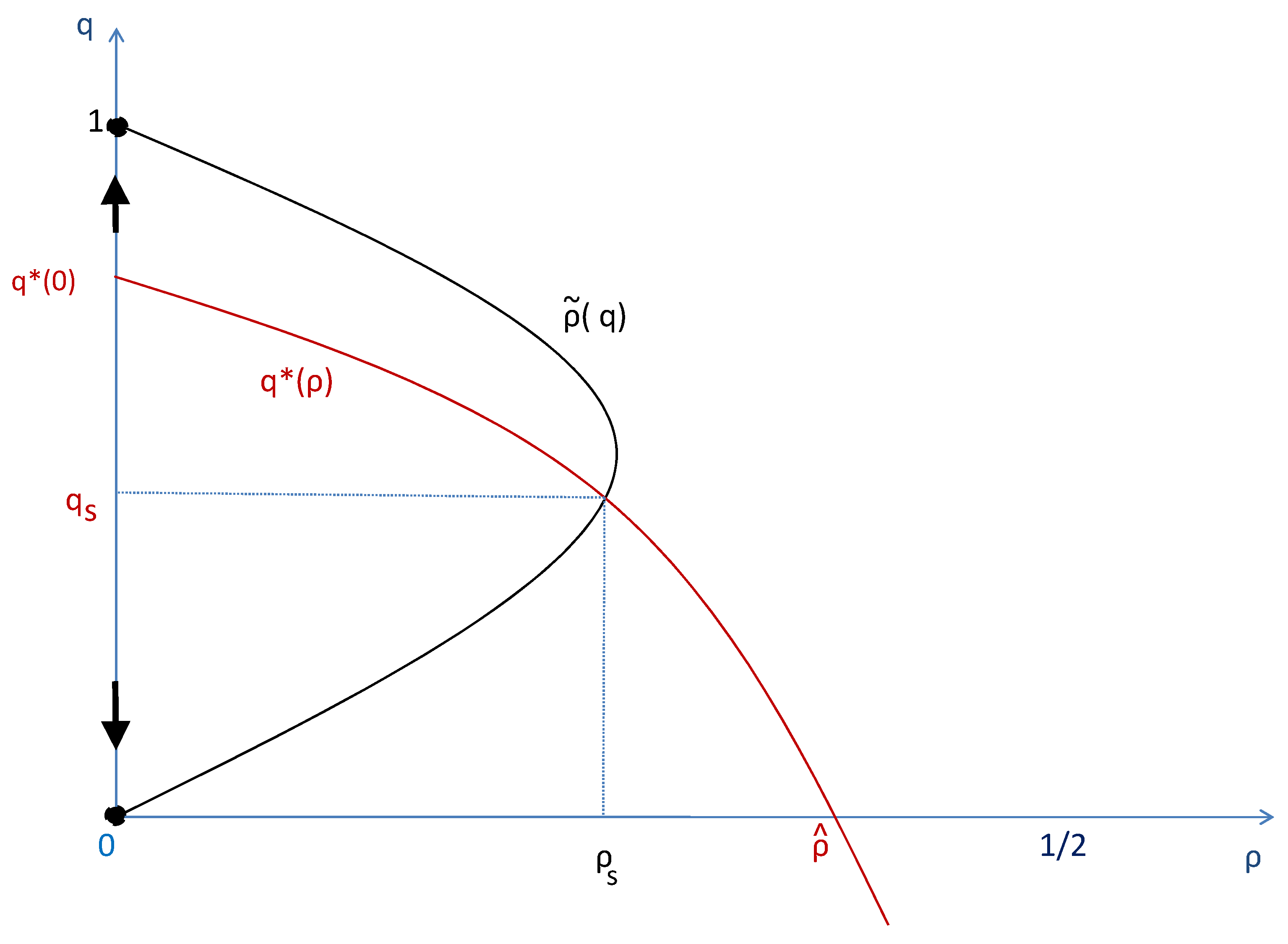
Lemma 1. If and we have that and are two stable equilibria and there exists a unique interior unstable equilibrium .
The dynamics are illustrated in
Figure 2. On
Figure 2, we plot in the space
the two curves
and
; under our assumption
, they only cross at one interior point
. It is easy to see that for all
, we have
and therefore
. On the opposite for all
, we have
and consequently
.
4.2. Adding the Spatial Structure
We now add the spatial structure as we did in the basic model with exogenous assortative matching. The partial differential equation driving the spatial evolution of the culture of cooperation in the domain
writes now as:
As we saw before, the sign of the speed of the traveling wave is given by the sign of the integral of the reaction term, i.e., the right-hand side of (
12).
where we have used the fact that
.
In order to have more precise analytic results, we parametrize the cost function as
with
From this, it follows that the optimal institutional degree of segregation
is determined by:
and we have
and
.
Since
is monotonic in
for all
we obtain that
when
13. In the sequel, we will therefore limit our attention to
ensuring therefore that for all
the interior steady state
is strictly positive.
We obtain the following characterization of a traveling wave with endogenous assortative matching institutions:
Proposition 3. There exists such that:
if there exists a traveling wave for all , such that an initially localized cluster of cooperators may invade the full spatial population;
if there exists such that for there is a traveling wave with positive speed such that a localized cluster of cooperators may invade the full population; conversely, for there exists a traveling wave such that a localized cluster of defectors may invade the full population;
if there exists a traveling wave for all such that a localized cluster of defectors may invade the full population.
The proof of the proposition is relegated to the appendix but is mainly based, as in the case of the exogenous institution, on the computation of the sign of the integral
that characterizes the sign of the speed of diffusion of a traveling wave solution of (
12).
The first part of the previous proposition states that when the gains from cooperation (material payoff, moral payoff or both) are high enough, a localized cluster of cooperators above the threshold may invade the whole population by slowly diffusing spatially independently of the cost of building an assortative institution that protects locally the cooperators. Importantly this cultural diffusion process is accompanied by a spatial institutional life cycle process. When cooperative preferences diffuse to a new location , cooperators at this location start to use their “political influence” (as reflected by their local frequency) to promote the building up of an assortative matching institution that protects them from interacting with defectors. Thanks to that, in that location x, the intergenerational incentives to transmit cooperative preferences are further enhanced locally, and the proportion of individuals endowed with a culture of cooperation keeps on growing in the community. This in turn supports a steadily increasing equilibrium institutional level of assortativity in the community. This positive interaction between the cultural dynamics of cooperative preferences and the institutional dynamics of assortativity continues until the frequency of cooperators crosses a certain threshold. At this stage, there are enough cooperators in the local community that it becomes less worth paying the cost of a highly selective social matching institution. Consequently, the equilibrium institutional degree of assortativity starts to decline, while the cultural diffusion of cooperative preferences steadily increases. This process goes on up to the point where the community is fully composed of cooperators and the assortative matching institution is abandoned because of its positive cost.
Interestingly, during the diffusion of the culture of cooperation in a local community, the life cycle of the local institution of assortative matching provides an example where the institutional and cultural dynamics exhibit a first phase of dynamic complementarities (with positive feedbacks between the evolution of assortative matching institution and the diffusion of the culture of cooperation), and then a second phase of dynamic substituabilities (where on the contrary the assortative matching institution coevolves negatively with the culture of cooperation).
The second part of the proposition highlights the case where the gains of cooperation take intermediate values, not high enough to always ensure the diffusion of a culture of cooperation and not too low to always induce defectors invasion. In this case, when institutional building is not too costly (associated with low values of , i.e., ), it can help a localized cluster of cooperators to diffuse in the whole space and invade the whole population. Conversely, the proposition also says that, if the cost to maintain an assortative matching institution is too high (associated with values of such that ), there exists a traveling wave in which now a localized cluster of defectors is able to invade a population of cooperators by diffusing in the whole space.
Lastly, the third part of the proposition states that when the gains of cooperating are too low, a localized cluster of defectors above the threshold may invade a population of cooperators by slowly diffusing spatially independently of the cost of building an assortative institution.
4.3. Diffusion of the Endogenous Institution
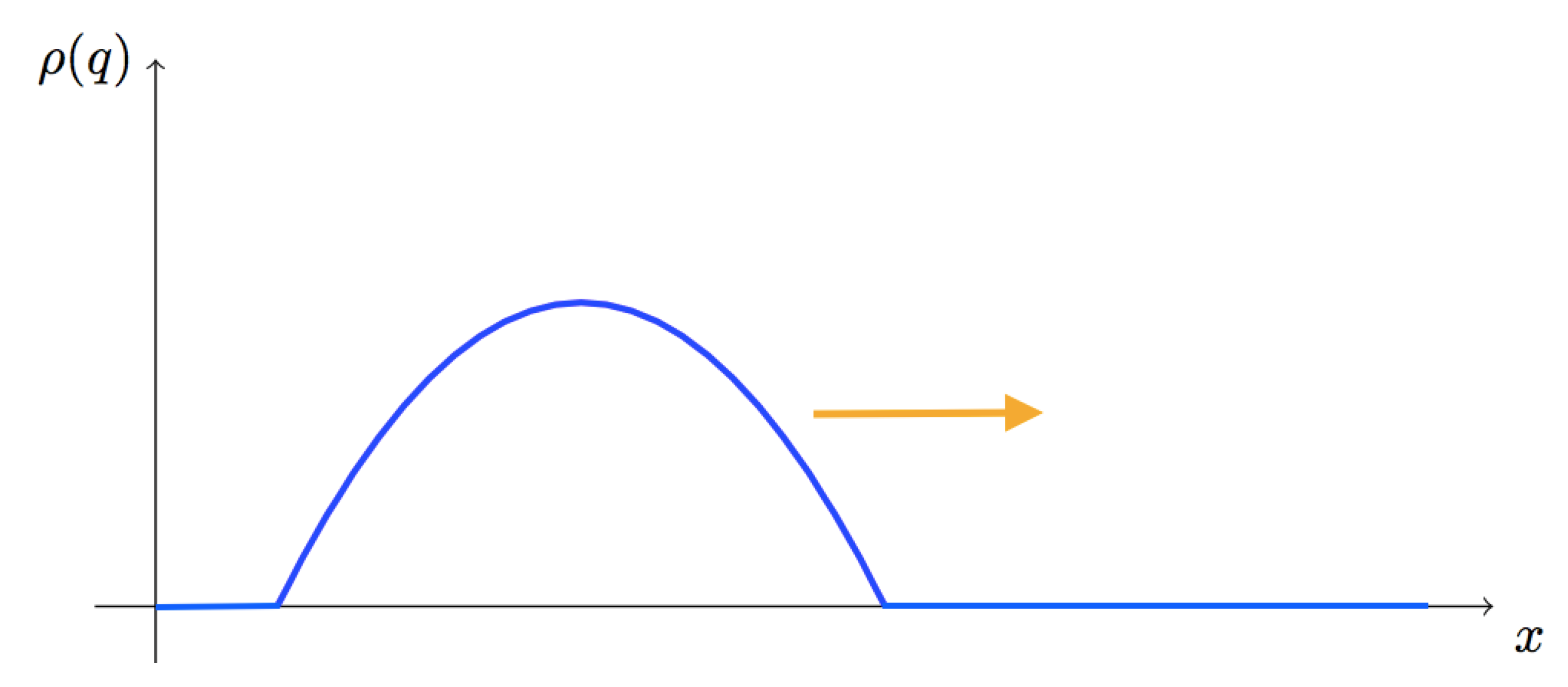
As already noted, when cooperators are able to invade the whole population, the endogenous assortative matching institution that supports such diffusion of a culture of cooperation follows a life cycle process as described in
Figure 3. As cooperative preferences start to appear in sufficient frequency in a given place
x, the institution of assortative matching emerges as well at
and increasingly helps cooperator individuals to segregate. This promotes further a local cultural change biased towards preferences for cooperation. When the local frequency of cooperators passes a threshold, the local institution of assortative matching enters in a declining phase and slowly disappears from location
x.
It is useful to compare the case where the level of the institution is exogenously settled (see
Section 4) to the case where the population can decide endogenously the degree of social segmentation associated to the institution.
For this, recall from Proposition 2 that for the case of an exogenous institution of assortative matching there is a threshold of assortativeness such that with any given matching institution with assortativeness there exists the possibility of the spatial diffusion of a preference for cooperation (i.e., the existence of a traveling wave of a culture of cooperation).
Recall as well from (
10) that the highest degree of social segmentation
that can be obtained with an endogenous institution associated with an implementation cost
is given by the condition
Then we have the following proposition:
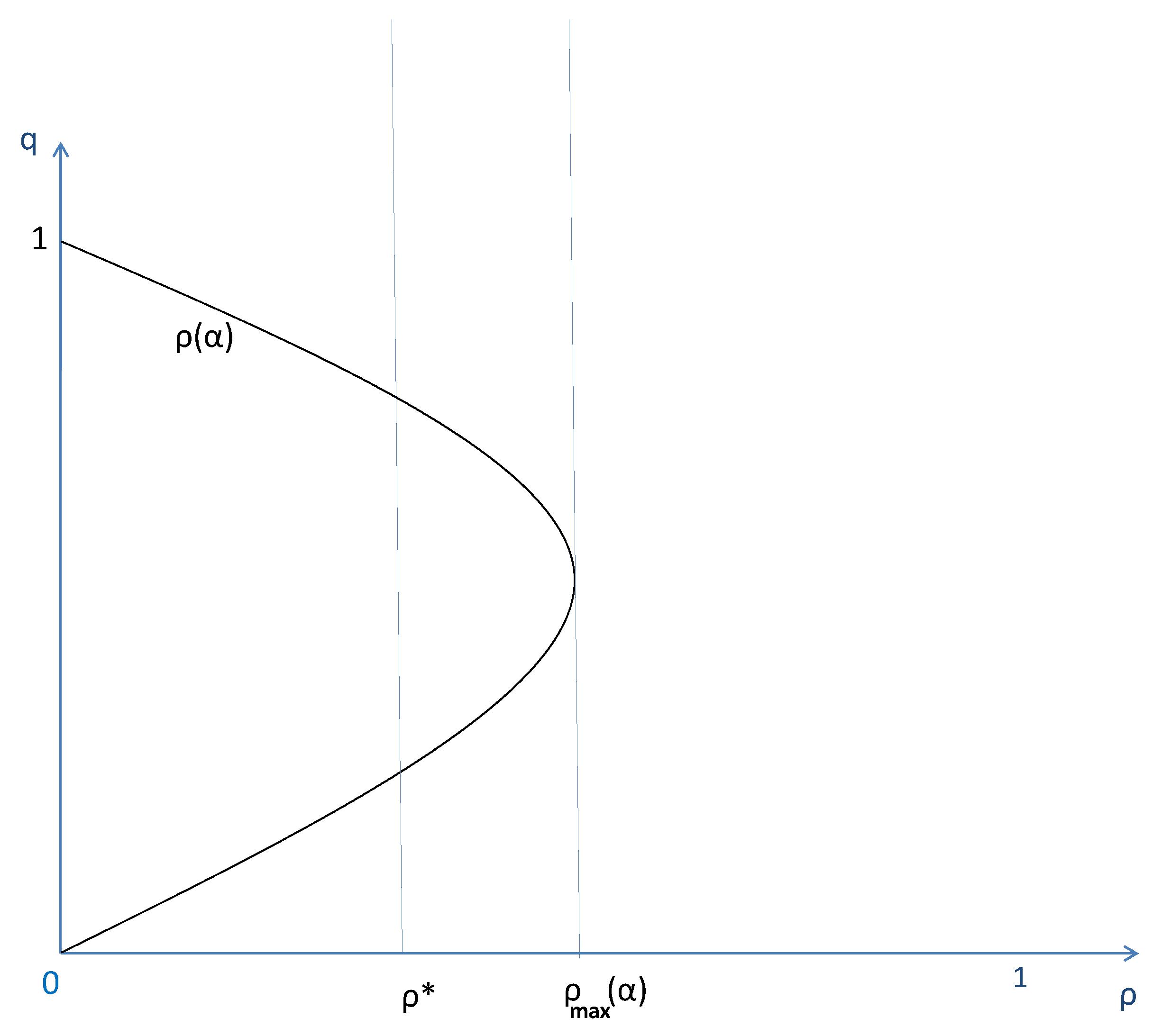
Proposition 4. When for any such that there is a traveling wave allowing cooperators to invade the full population, the maximum degree of segmentation generated along that wave by the assortative matching institutions is such that .
This proposition explores the intermediate parameters case
where both traveling waves for full diffusion of cooperative preferences and diffusion of defector preferences can exist depending on the cost of the institution. It says that along a traveling wave that allows the spatial diffusion of the culture of cooperation with an endogenous assortative matching institution (i.e., when the cost of institutional building is not too high with
), this institution, at its peak, always segments more than the minimum degree of segmentation
necessary to get the same diffusion of cooperative preferences with exogenous assortative matching. This highlights the fact that along its life cycle associated to the diffusion of the preferences for cooperation (see
Figure 4), the local endogenous matching institutions both start with a low degree of assortativity and steadily become more separative than what would be necessary to allow the diffusion of cooperation with exogenous assortative matching. These two elements reflect the importance of the joint interaction between institution building and diffusion of preferences that is not taken into account in
Section 4 with exogenous matching institutions.