Zero-Axis Virtual Synchronous Coordinate Based Current Control Strategy for Grid-Connected Inverter
Abstract
:1. Introduction
2. Principle Analysis of SPC
2.1. Unbalance Current Analysis
2.2. Compensation Principle of SPC
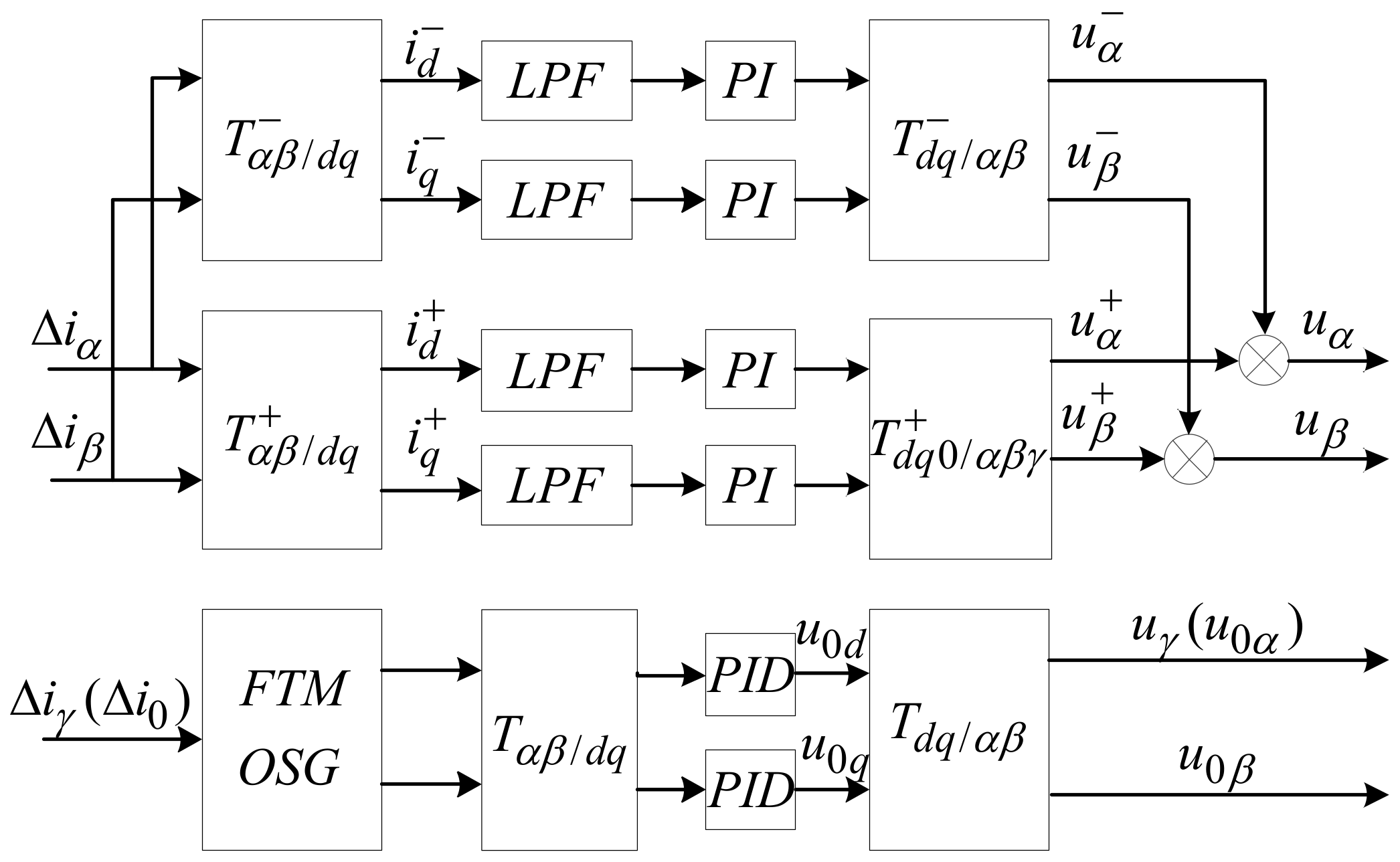
3. Current Control Strategy Based on Zero-Axis Signal Reconstruction
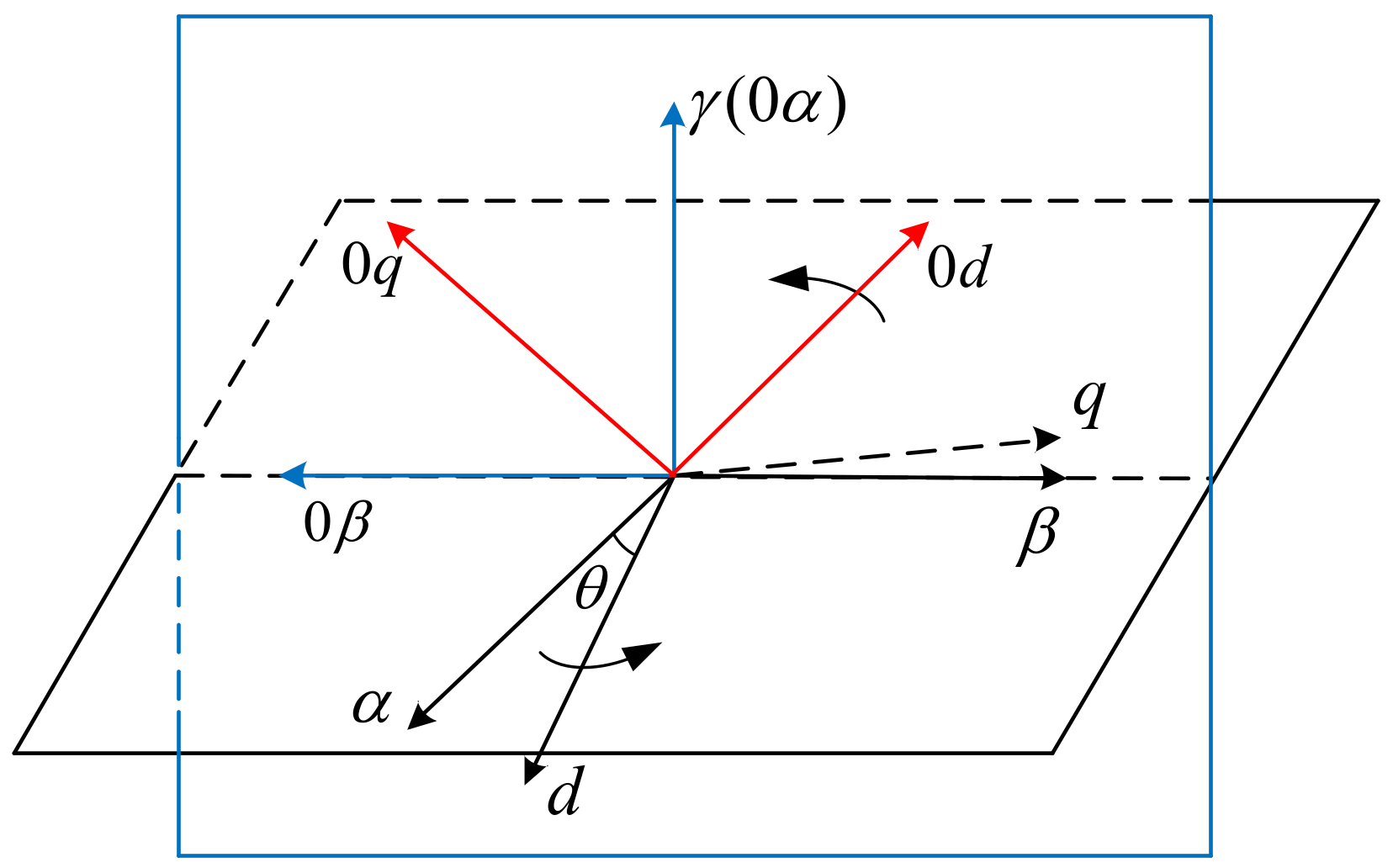
3.1. Design of the Virtual Zero-Axis Coordinate System
3.2. Complex Filter Matrixes Transformation
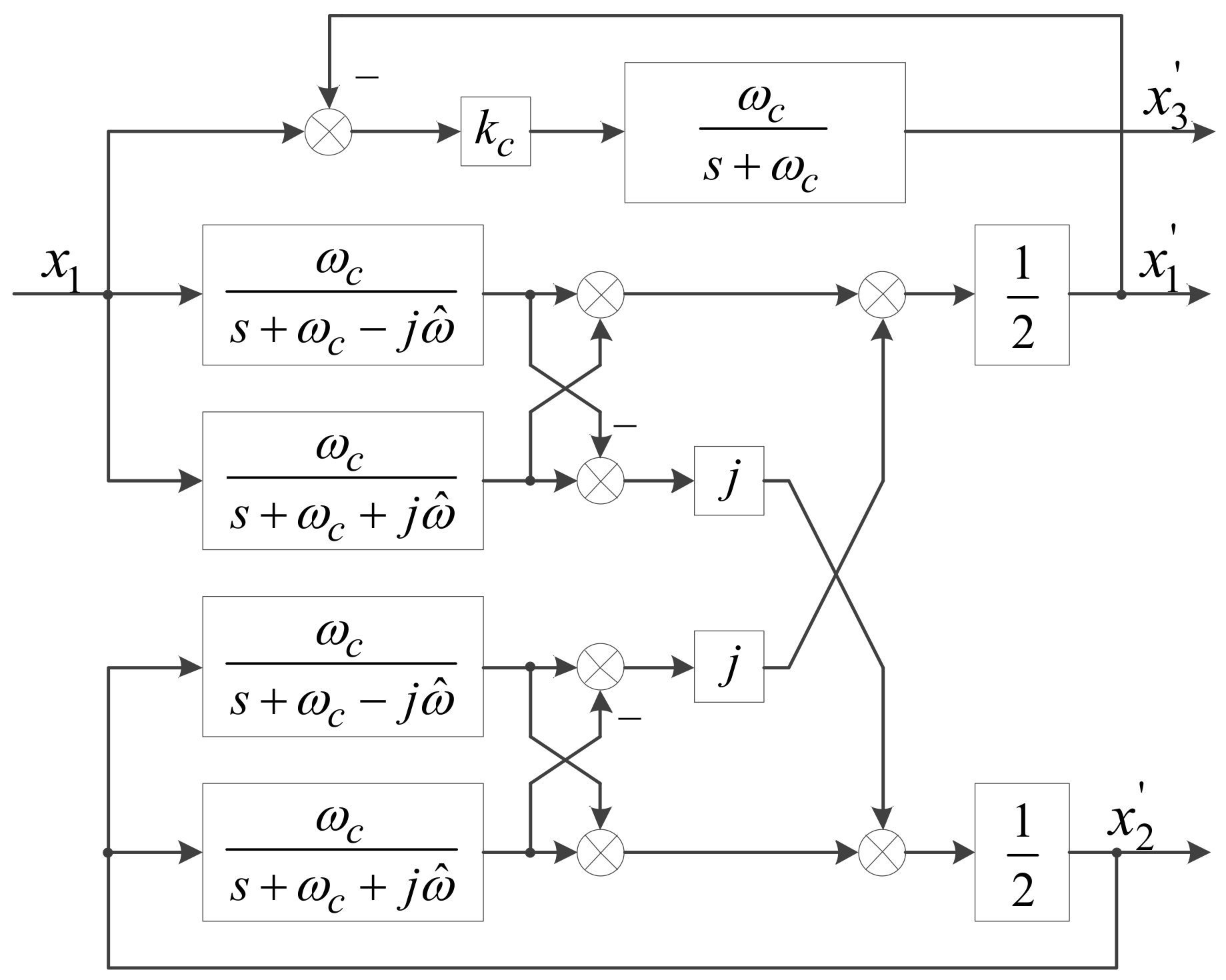
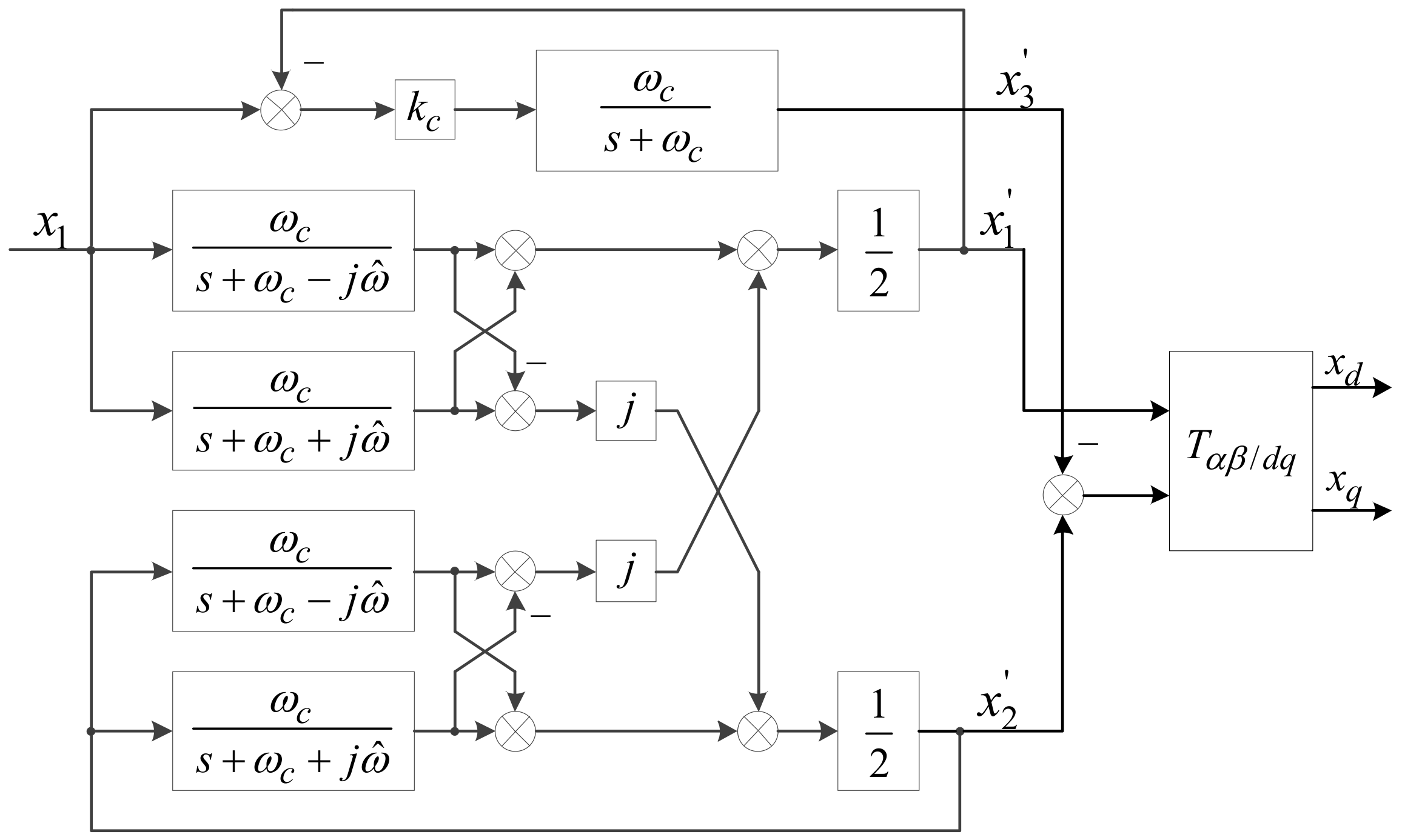
3.3. Design of Zero-Axis Control System
4. Analysis and Design of the Zero-Axis Control System
4.1. State Space Analysis of Zero-Axis Virtual Coordinate System
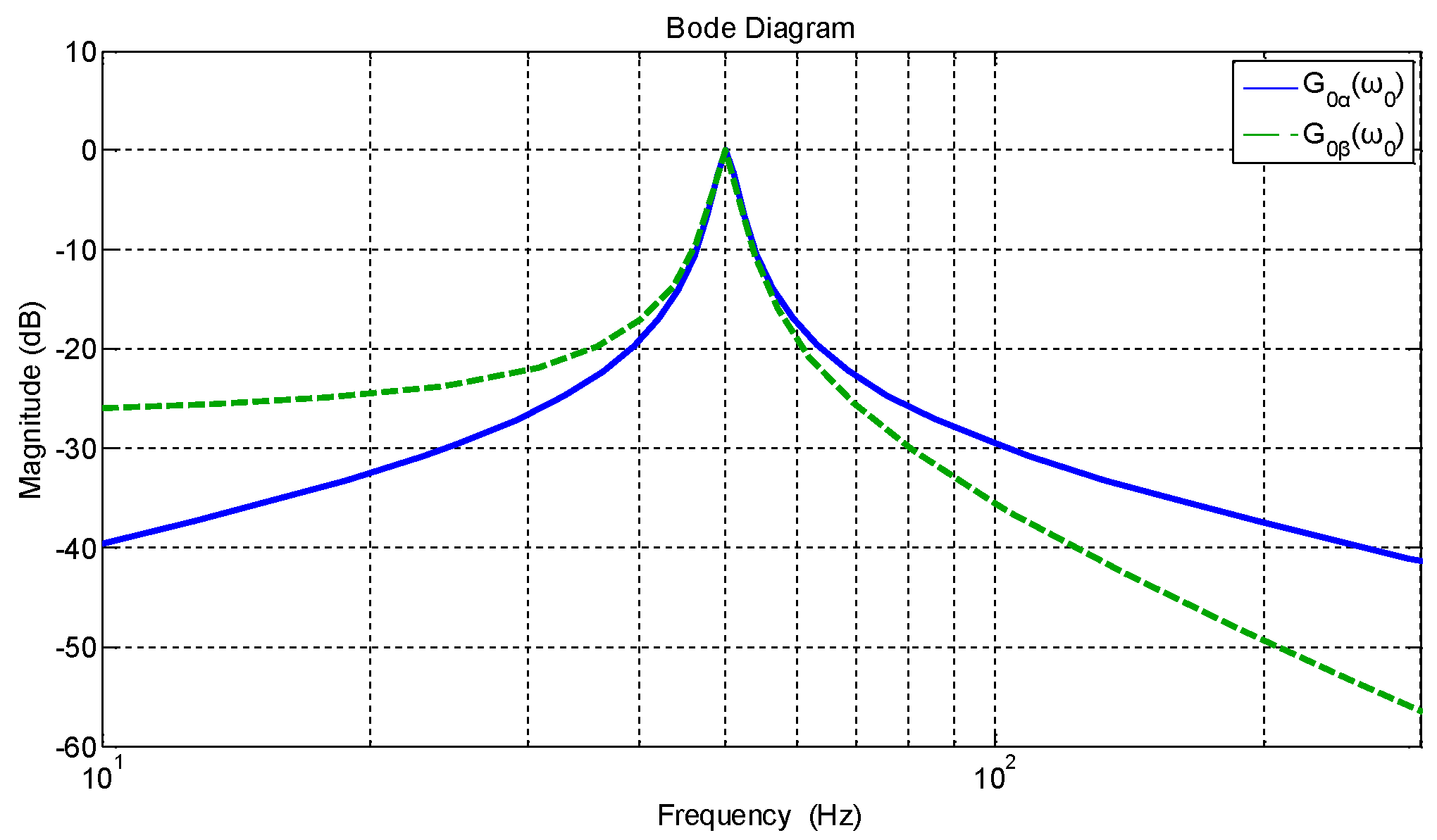
4.2. Parameter Analysis of Zero-Axis FTM-OSG
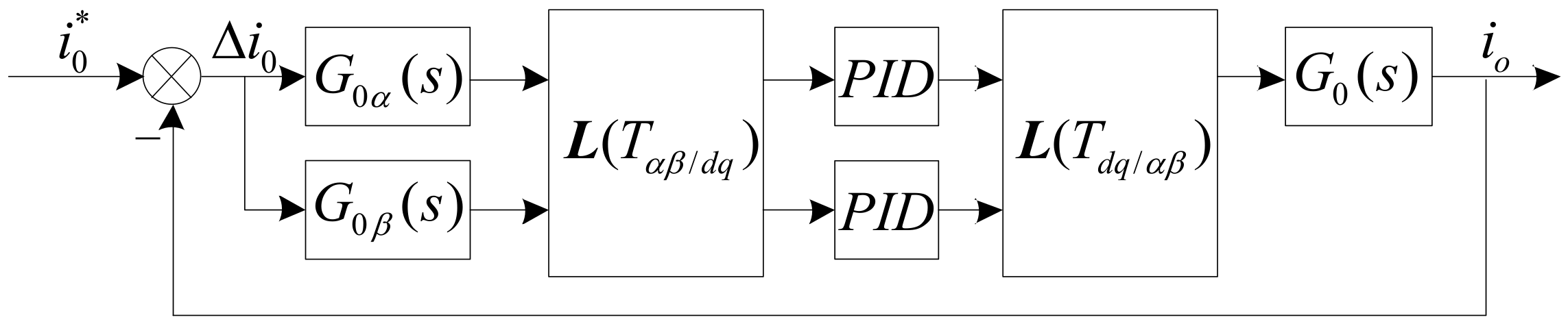
4.3. Coordinate Transformation Analysis of Control System
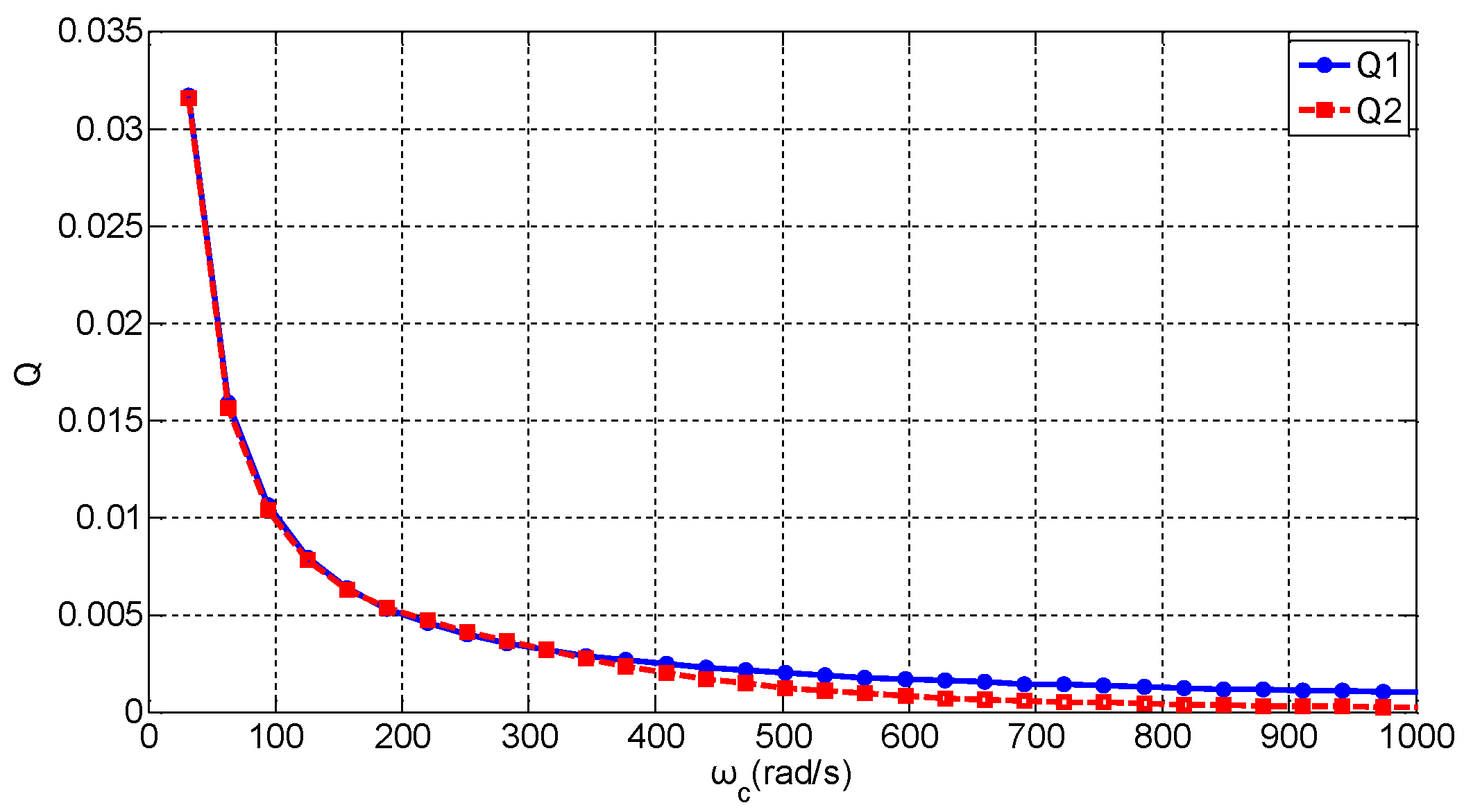
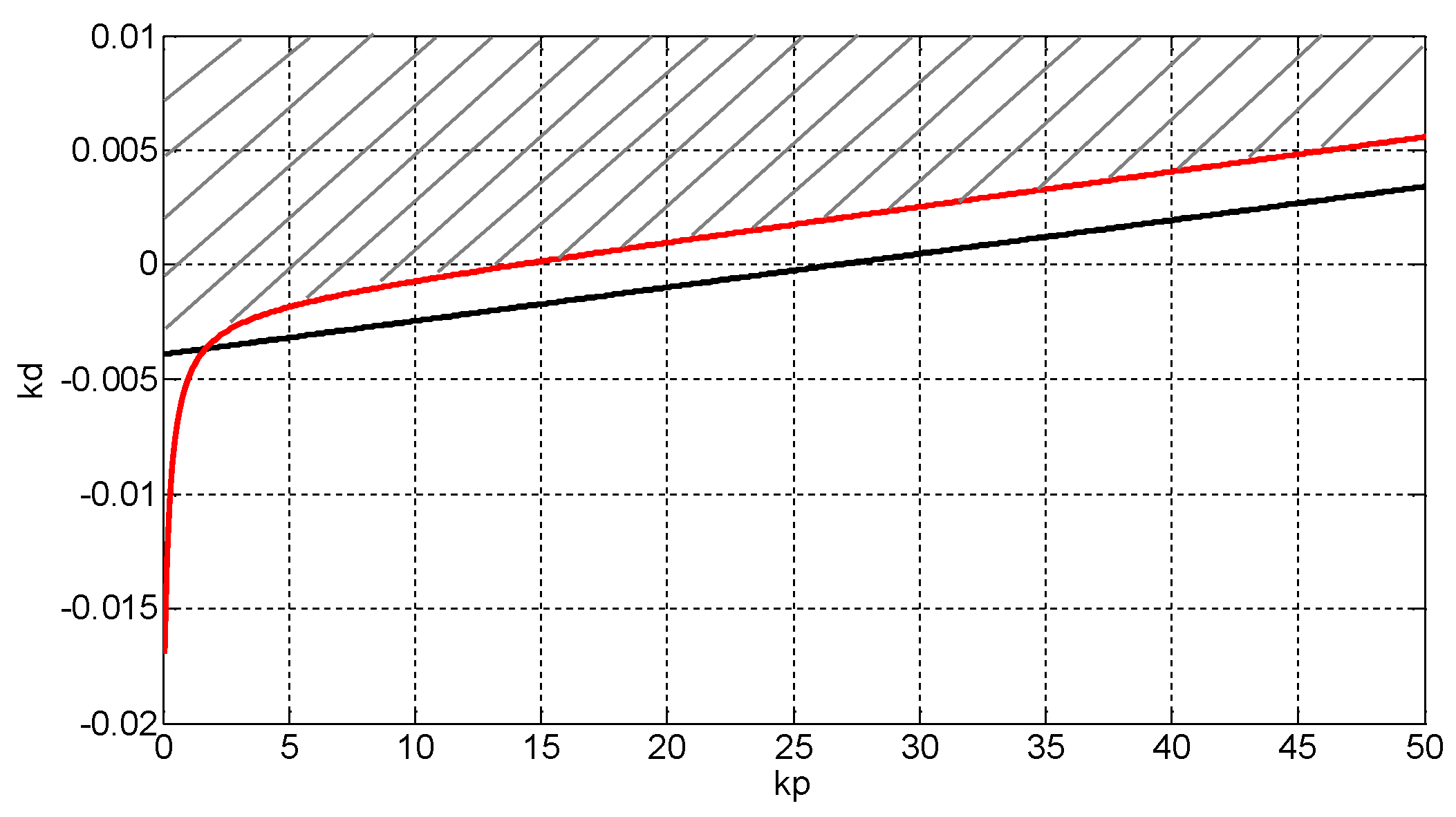
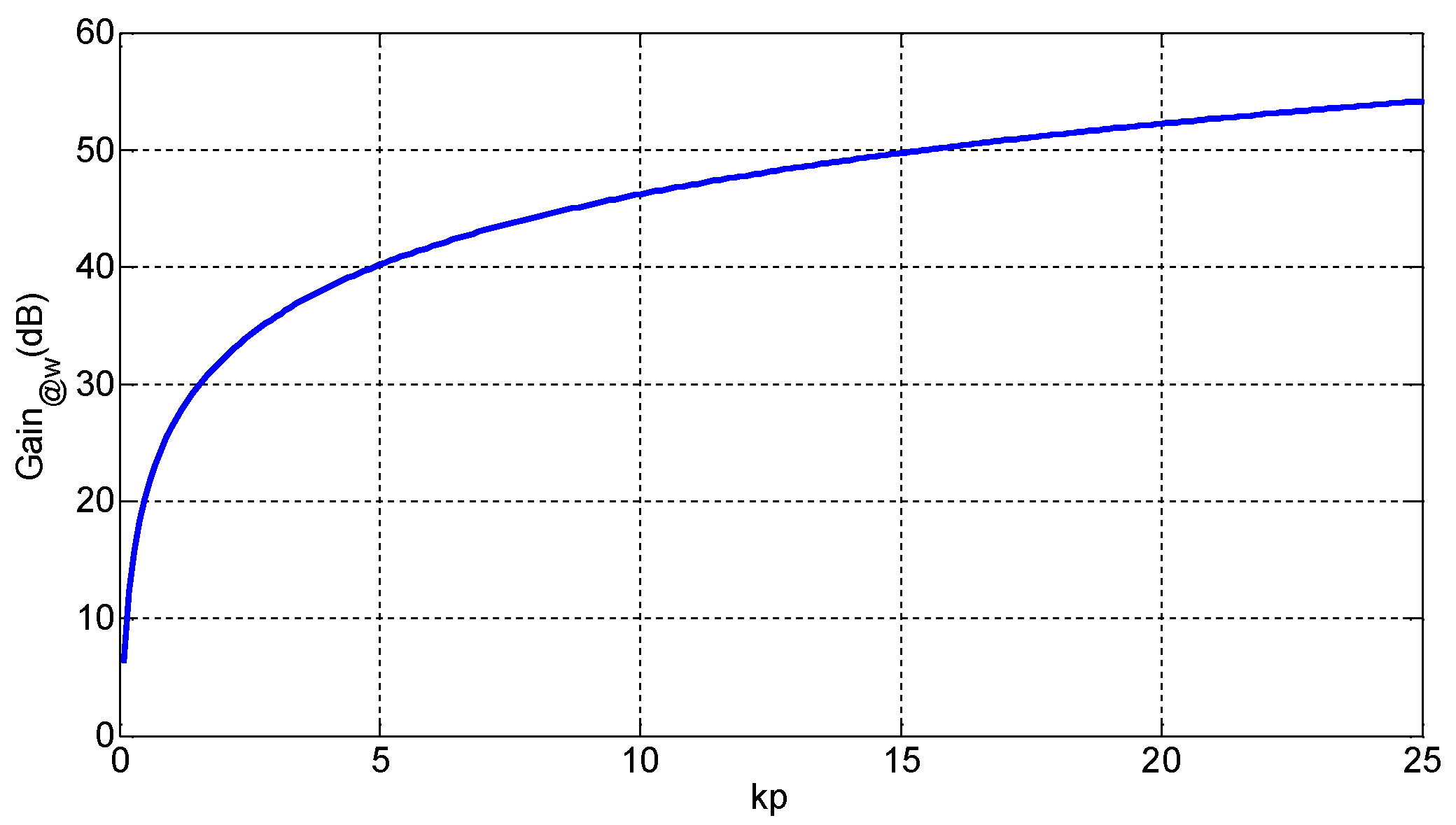
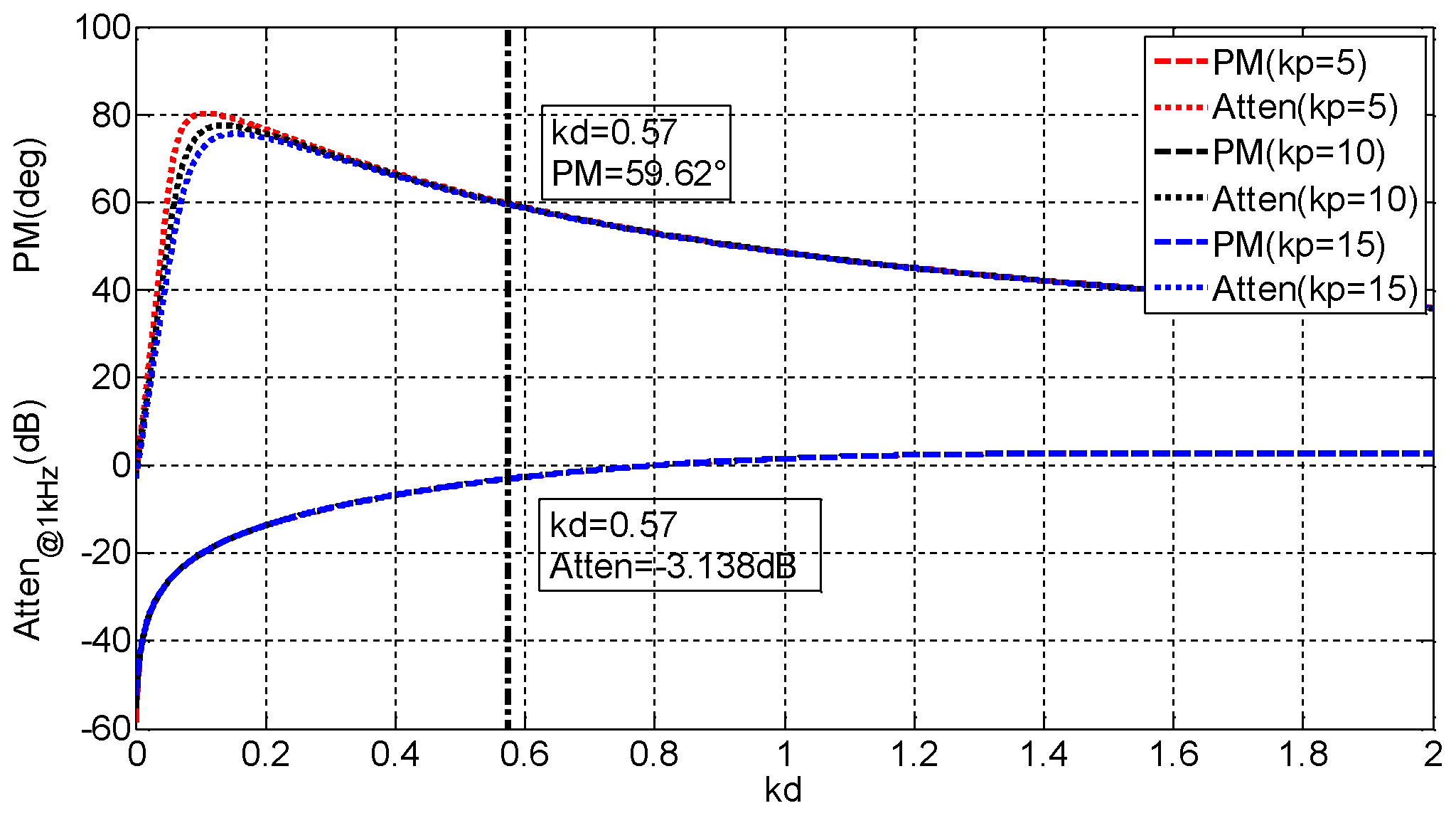
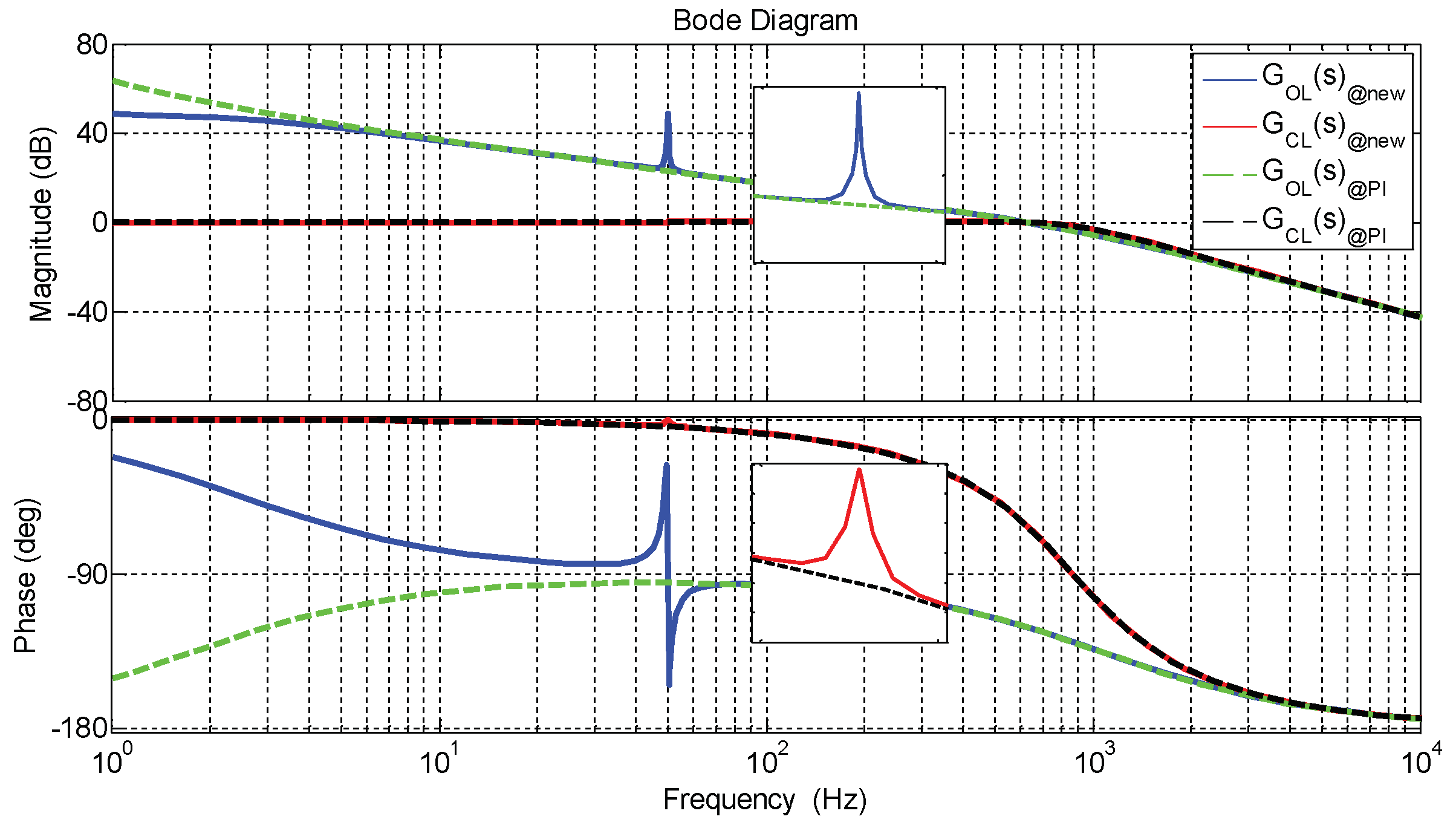
4.4. Design of Controller Parameters
5. Simulations and Experiments
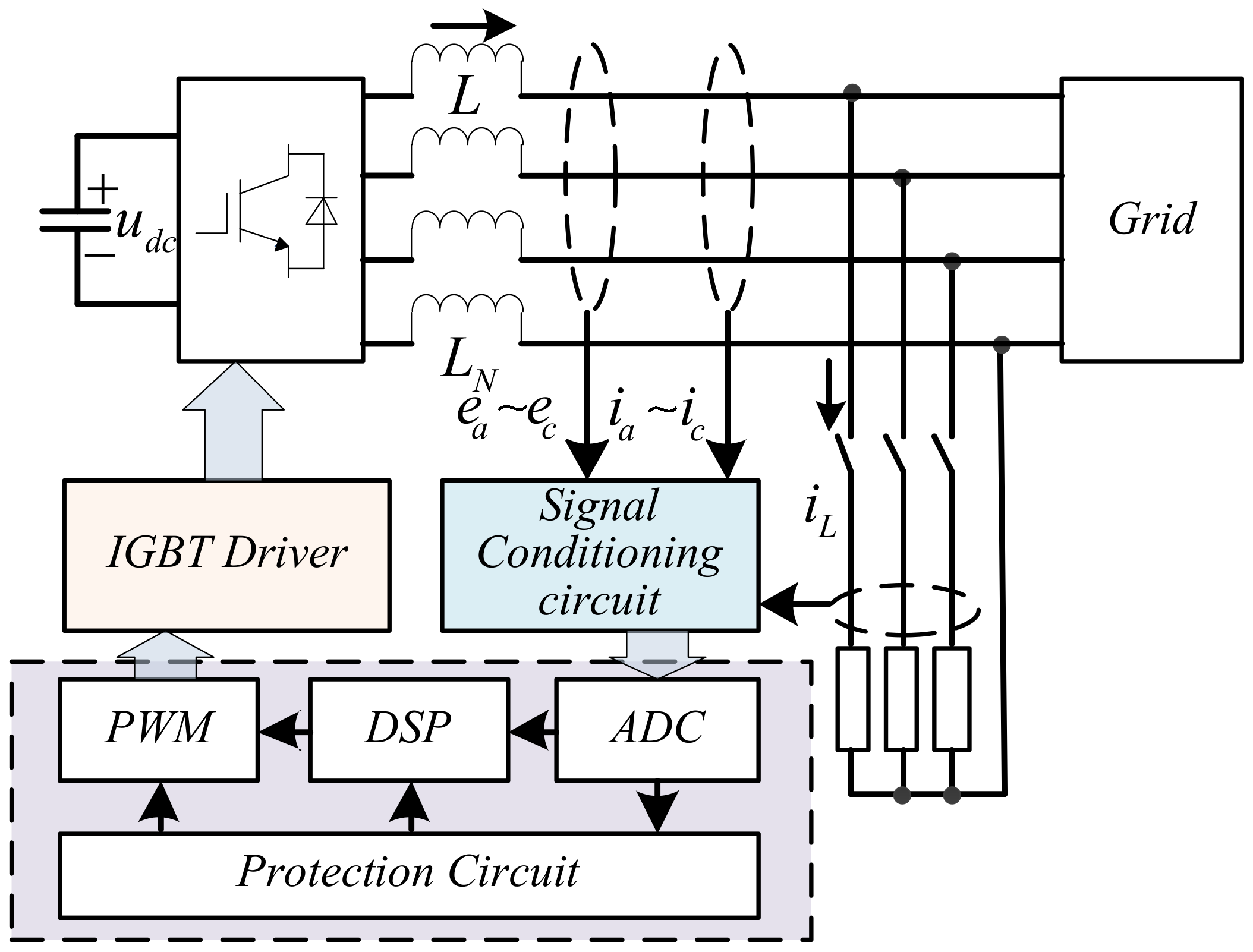
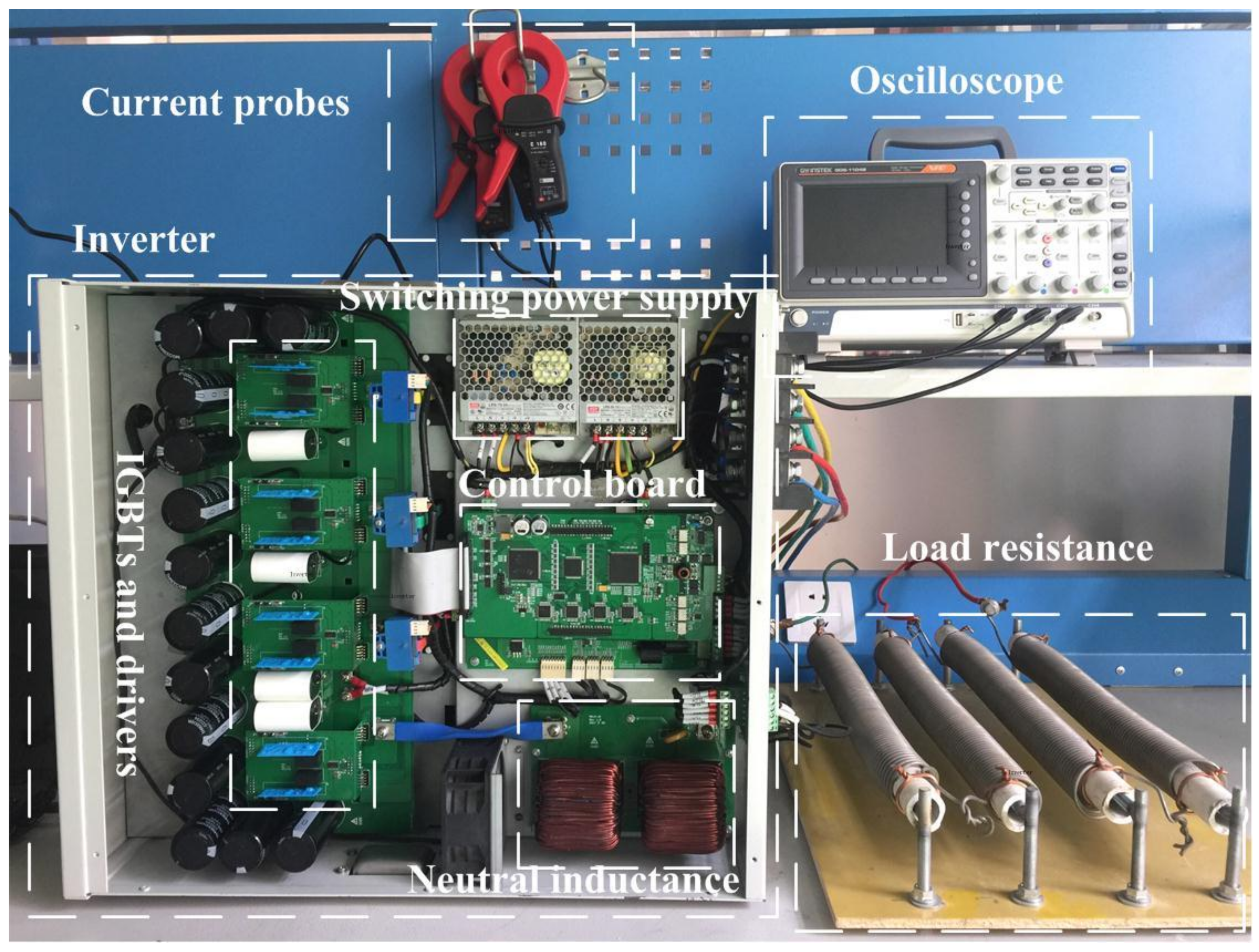
5.1. Simulation and Experimental Conditions
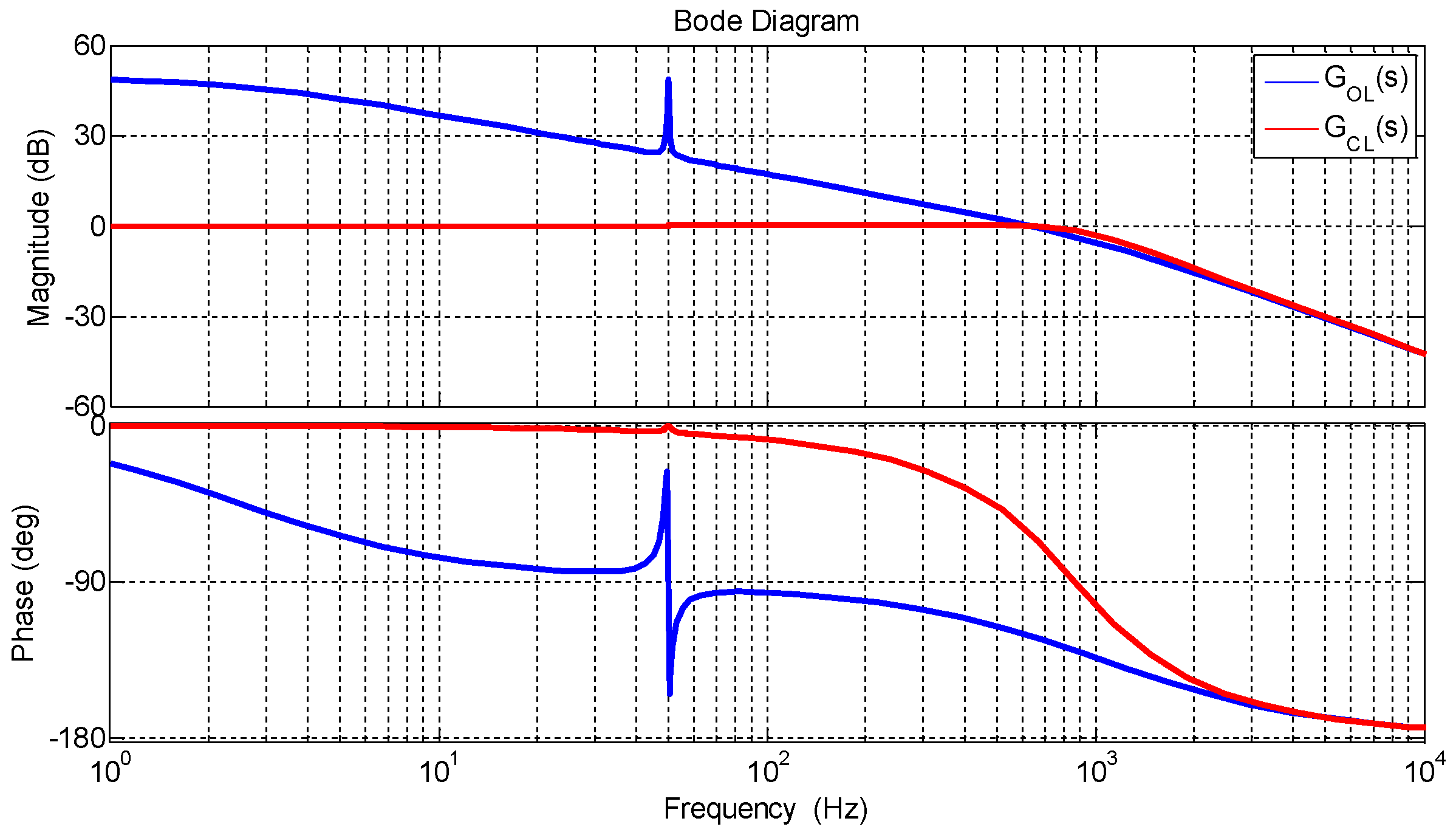
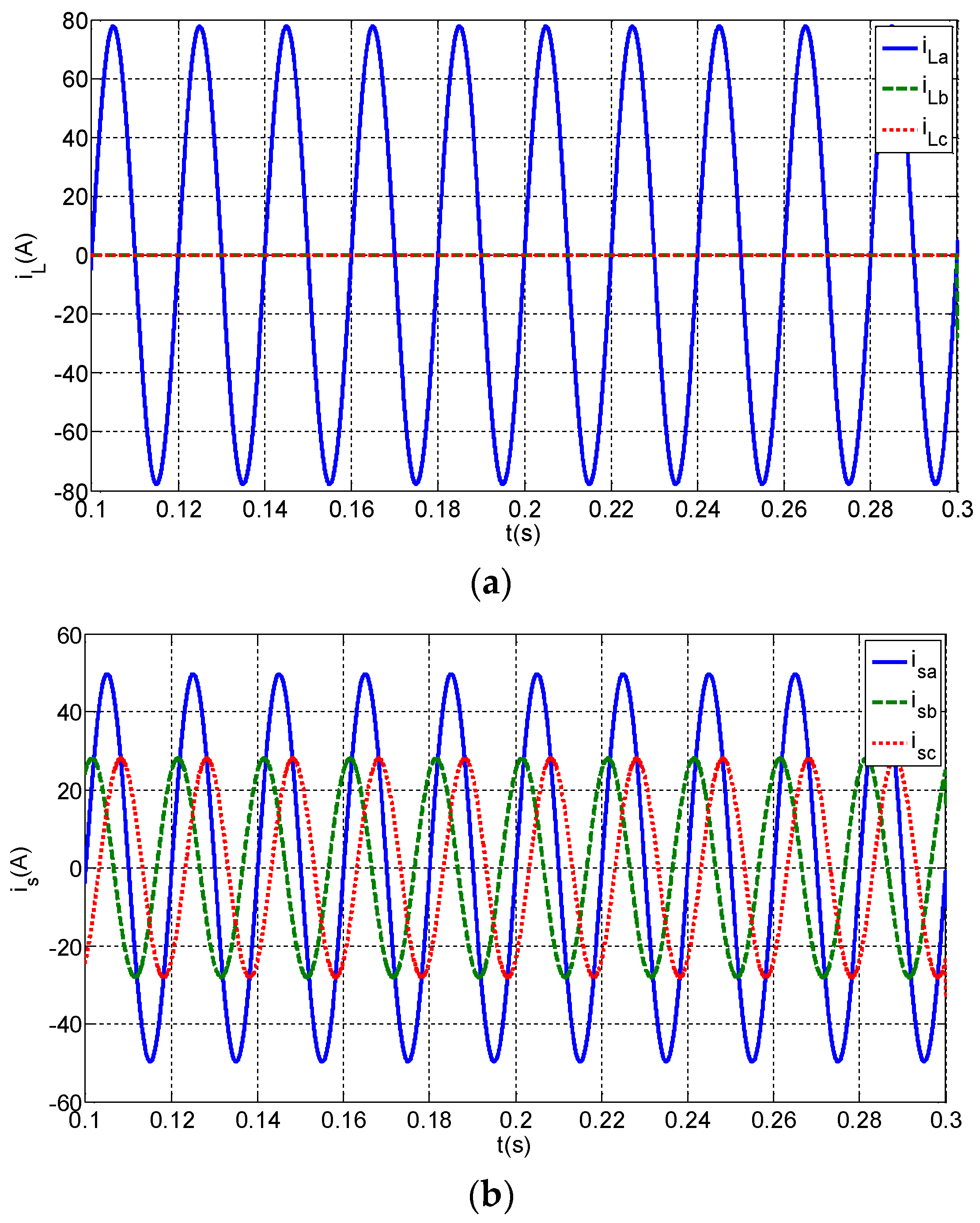
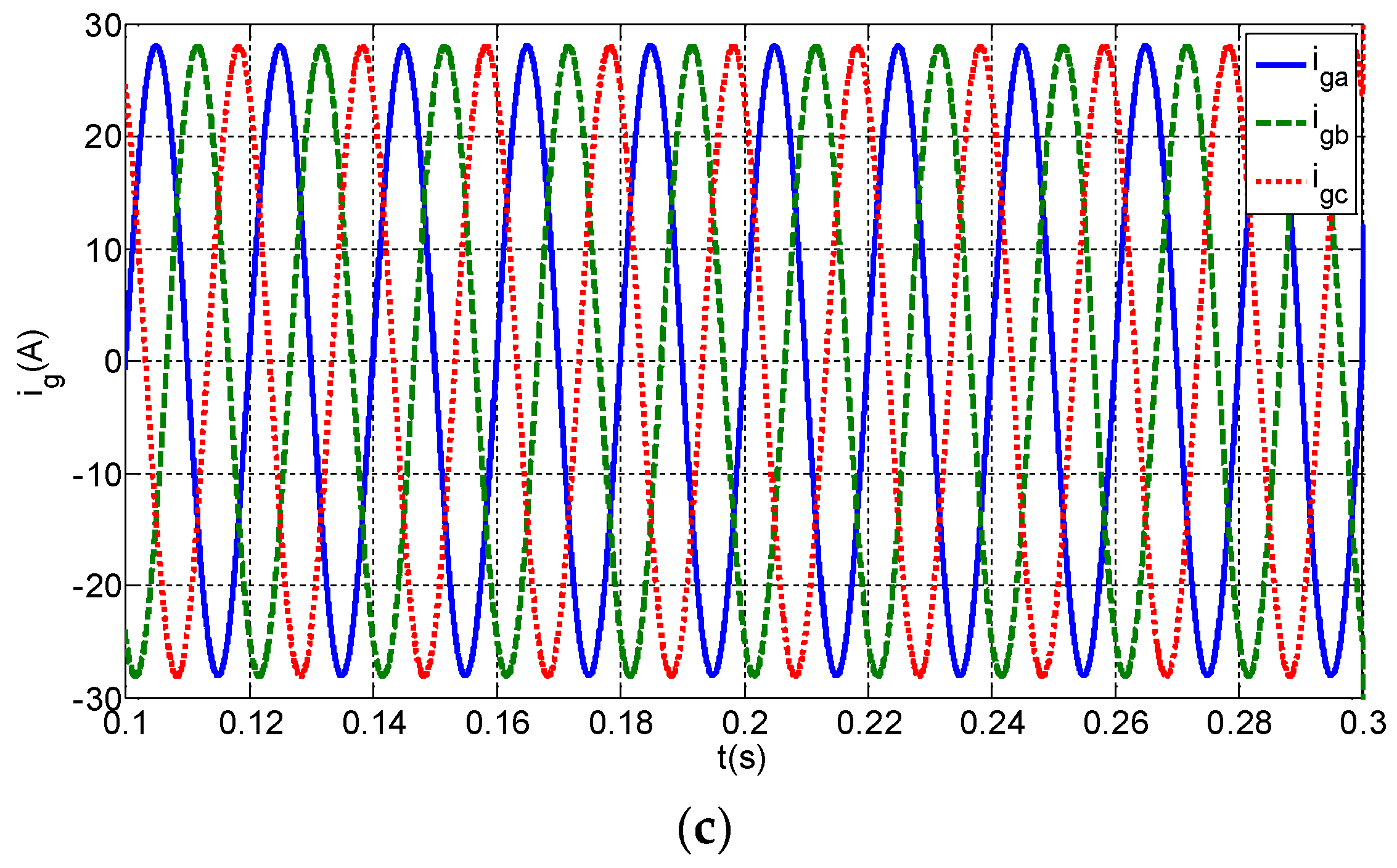
5.2. Steady State Analysis of System
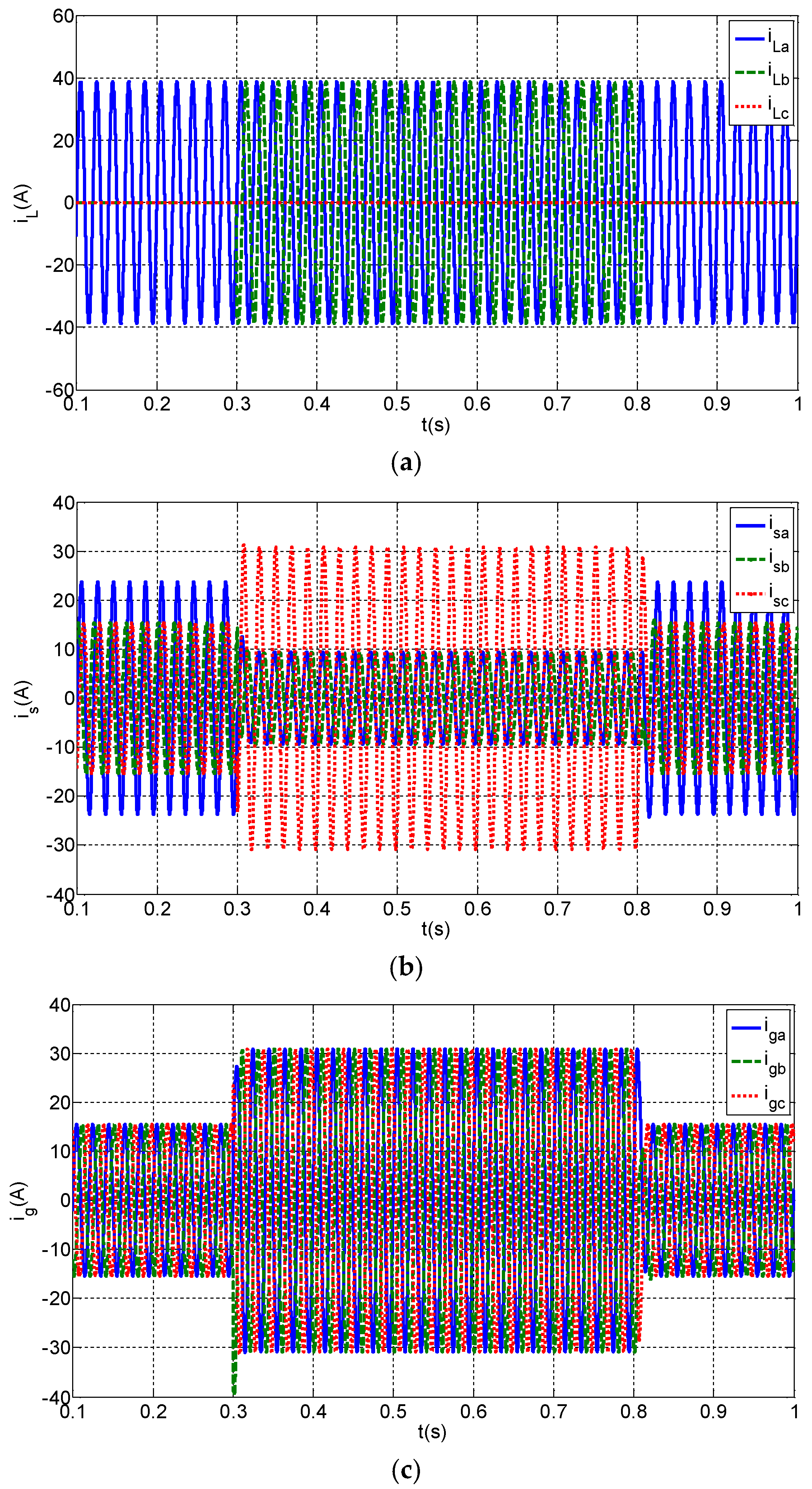
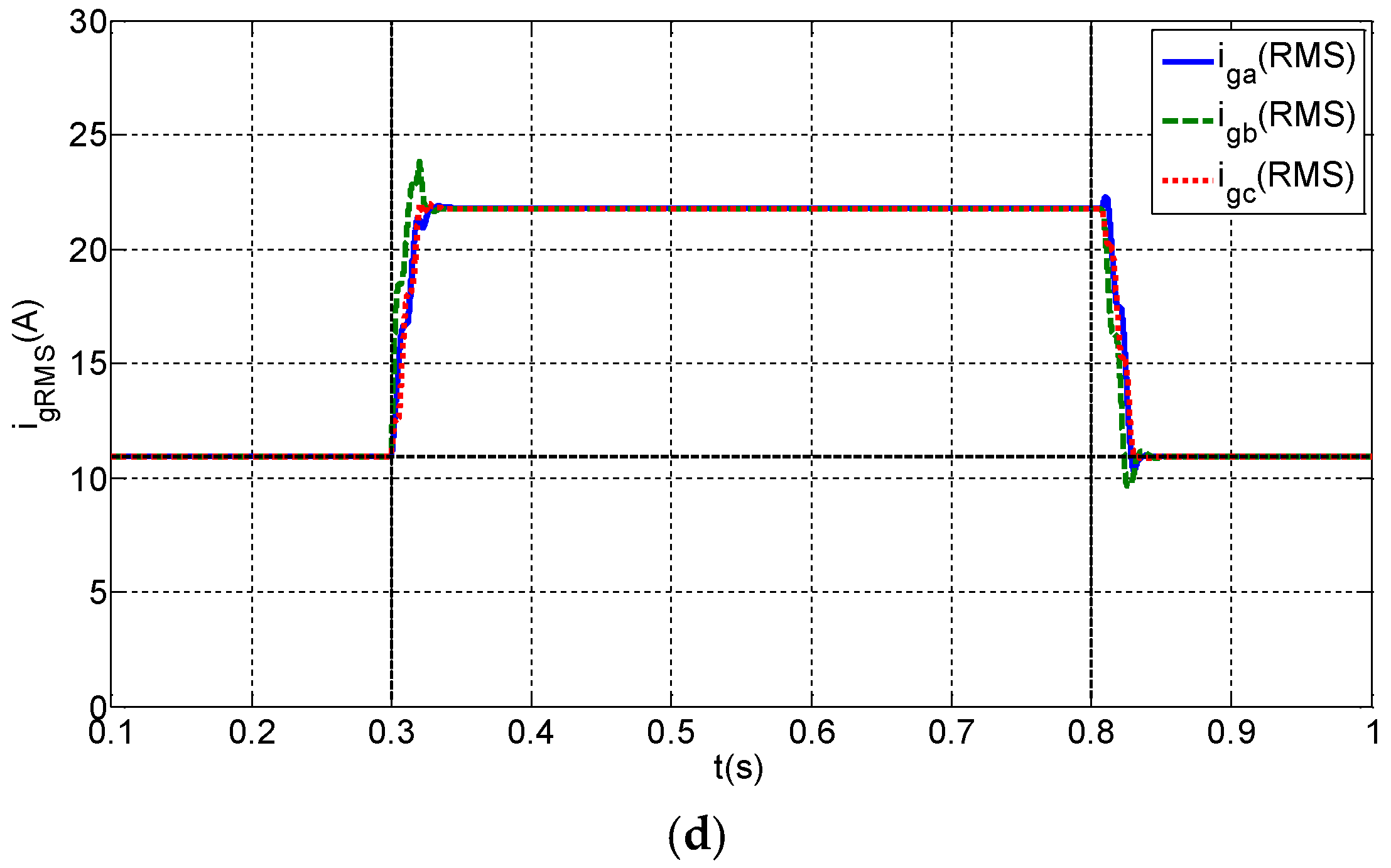
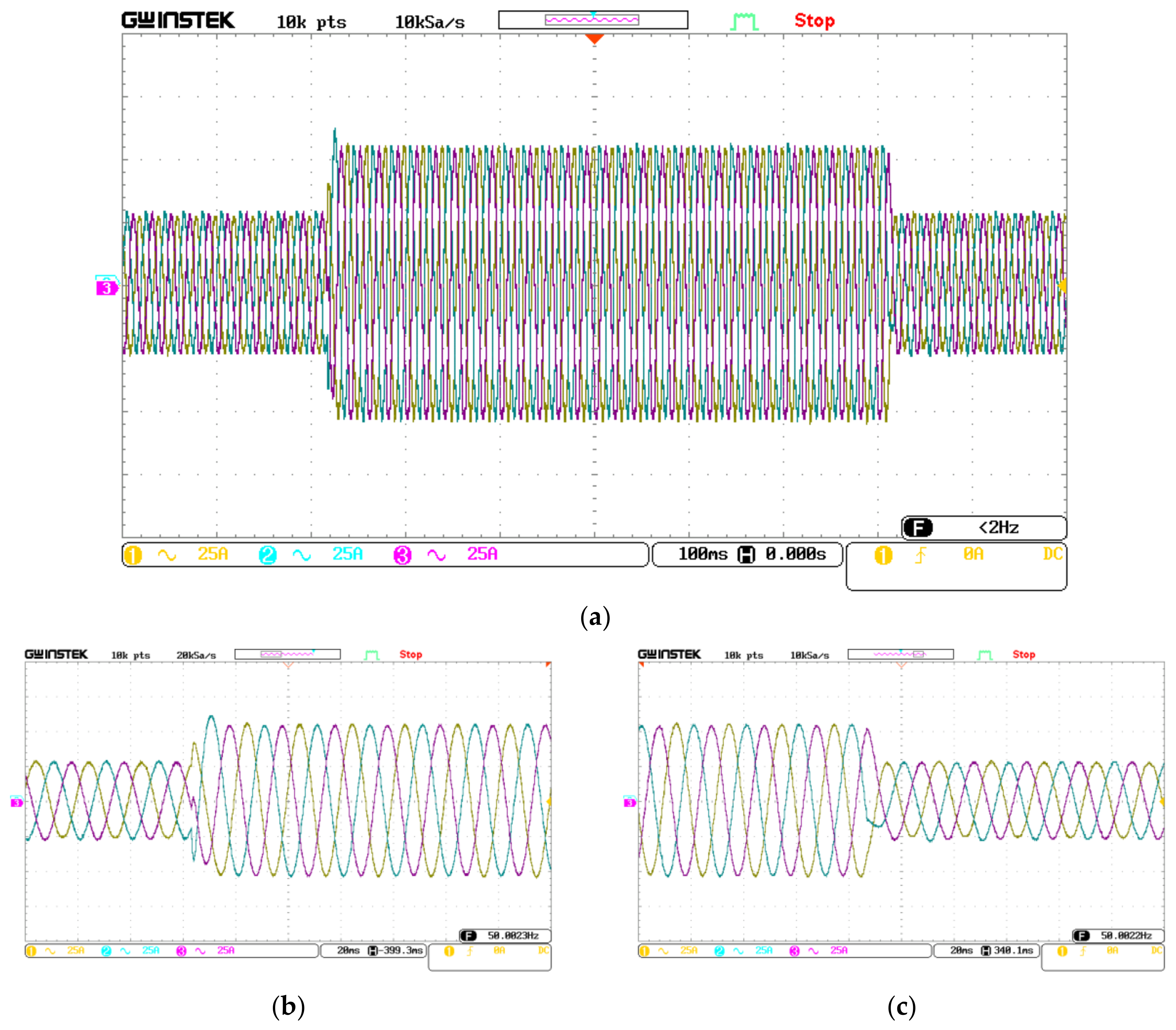
5.3. Dynamic Analysis of System
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
References
- Kong, W.; Ma, K.; Wu, Q. Three Phase Power Imbalance Decomposition into Systematic Imbalance and Random Imbalance. IEEE Trans. Power Syst. 2017, 33, 3001–3012. [Google Scholar] [CrossRef]
- Yan, S.; Tan, S.C.; Lee, C.K.; Chaudhuri, B.; Hui, S.Y.R. Electric Springs for Reducing Power Imbalance in Three-Phase Power Systems. IEEE Trans. Power Electron. 2015, 30, 3601–3609. [Google Scholar] [CrossRef] [Green Version]
- Hamajima, T.; Hu, N.; Ozcivan, N.; Soeda, S.; Yagai, T.; Tsuda, M. Balanced Three-Phase Distributions of Tri-Axial Cable for Transmission Line. IEEE Trans. Appl. Supercon. 2009, 19, 1748–1751. [Google Scholar] [CrossRef]
- Moses, P.S.; Masoum, M.A.S. Three-phase asymmetric transformer aging considering voltage-current harmonic interactions, unbalanced nonlinear loading, magnetic couplings, and hysteresis. IEEE Trans. Energy Conver. 2012, 27, 318–327. [Google Scholar] [CrossRef]
- Yousefi, B.; Soleymani, S.; Mozafari, B.; Gholamian, S.A.; Sciubba, E. Speed control of matrix converter-fed five-phase permanent magnet synchronous motors under unbalanced voltages. Energies 2017, 10, 1509. [Google Scholar] [CrossRef]
- Lopez, J.V.; Rodriguez, J.C.C.; Fernandez, S.M.; Garcia, S.M.; Garcia, M.A.P. Synthesis of fast onload multitap-changing clamped-hard-switching ac stabilizers. IEEE Trans. Power Deliv. 2006, 21, 862–872. [Google Scholar] [CrossRef]
- Gonzalez, D.; Hopfeld, M.; Berger, F.; Schaaf, P. Investigation on contact resistance behavior of switching contacts using a newly developed model switch. IEEE Trans. Compon. Packag. Manuf. Technol. 2018, 1–11. [Google Scholar] [CrossRef]
- Shateri, H.; Jamali, S. Measured impedance by distance relay for inter phase faults in presence of resistive Fault Current Limiter. In Proceedings of the IEEE International Conference on Power System Technology, Hangzhou, China, 24–28 October 2010. [Google Scholar] [CrossRef]
- Rawat, N.; Bhatt, A.; Aswal, P. A review on optimal location of FACTS devices in AC transmission system. In Proceedings of the IEEE International Conference on Power, Energy and Control, Sri Rangalatchum Dindigul, India, 6–8 February 2013. [Google Scholar] [CrossRef]
- Mangaraj, M.; Panda, A.K. NBP-based icosφ control strategy for DSTATCOM. IET Power Electron. 2017, 10, 1617–1625. [Google Scholar] [CrossRef]
- Fujun, M.; An, L.; Qiaopo, X.; Zhixing, H. Derivation of zero-sequence circulating current and the compensation of delta-connected static var generators for unbalanced load. IET Power Electron. 2016, 9, 576–588. [Google Scholar] [CrossRef]
- Kim, S.; Park, S.Y.; Kwak, S. Simplified model predictive control method for three-phase four-leg voltage source inverters. J. Power Electron. 2016, 16, 2231–2242. [Google Scholar] [CrossRef]
- Yaramasu, V.; Rodriguez, J.; Wu, B.; Rivera, M.; Wilson, A.; Rojas, C. A simple and effective solution for superior performance in two-level four-leg voltage source inverters: Predictive voltage control. In Proceedings of the IEEE International Symposium on Industrial Electronics, Bari, Italy, 4–7 July 2010; pp. 3127–3132. [Google Scholar] [CrossRef]
- Abronzini, U.; Attaianese, C.; D’Arpino, M.; Monaco, M.D.; Tomasso, G. Steady-state dead-time compensation in VSI. IEEE Trans. Ind. Electron. 2016, 63, 5858–5866. [Google Scholar] [CrossRef]
- Yepes, A.G.; Vidal, A.; López, O.; Doval-Gandoy, J. Evaluation of techniques for cross-coupling decoupling between orthogonal axes in double synchronous reference frame current control. IEEE Trans. Ind. Electron. 2014, 61, 3527–3531. [Google Scholar] [CrossRef]
- Dian, R.; Xu, W.; Mu, C. Improved negative sequence current detection and control strategy for h-bridge three-level active power filter. IEEE Trans. Appl. Supercond. 2016, 26, 1–5. [Google Scholar] [CrossRef]
- Perez-Estevez, D.; Doval-Gandoy, J.; Yepes, A.G.; Lopez, O.; Baneira, F. Enhanced resonant current controller for grid-connected converters with lcl filter. IEEE Trans. Power Electron. 2018, 33, 3765–3778. [Google Scholar] [CrossRef]
- Nian, H.; Shen, Y.; Yang, H.; Quan, Y. Flexible grid connection technique of voltage-source inverter under unbalanced grid conditions based on direct power control. IEEE Trans. Ind. Appl. 2015, 51, 4041–4050. [Google Scholar] [CrossRef]
- Kuperman, A. Proportional-resonant current controllers design based on desired transient performance. IEEE Trans. Power Electron. 2015, 30, 5341–5345. [Google Scholar] [CrossRef]
- Marati, N.; Prasad, D. A modified feedback scheme suitable for repetitive control of inverter with non-linear load. IEEE Trans. Power Electron. 2018, 33, 2588–2600. [Google Scholar] [CrossRef]
- Zhao, Q.; Ye, Y. A pimr-type repetitive control for a grid-tied inverter: Structure, analysis, and design. IEEE Trans. Power Electron. 2018, 33, 2730–2739. [Google Scholar] [CrossRef]
- Bayhan, S.; Abu-Rub, H.; Balog, R.S. Model predictive control of quasi-z-source four-leg inverter. IEEE Trans. Ind. Electron. 2016, 63, 4506–4516. [Google Scholar] [CrossRef]
- Pichan, M.; Rastegar, H.; Monfared, M. Deadbeat control of the stand-alone four-leg inverter considering the effect of the neutral line inductor. IEEE Trans. Ind. Electron. 2017, 64, 2592–2601. [Google Scholar] [CrossRef]
- Pichan, M.; Rastegar, H. Sliding mode control of four-leg inverter with fixed switching frequency for uninterruptible power supply applications. IEEE Trans. Ind. Electron. 2017, 64, 6805–6814. [Google Scholar] [CrossRef]
- Hamidi, A.; Ahmadi, A.; Feali, M.S.; Karimi, S. Implementation of digital fcs-mp controller for a three-phase inverter. Electr. Eng. 2015, 97, 25–34. [Google Scholar] [CrossRef]
- Yang, L.Y.; Wang, C.L.; Liu, J.H.; Jia, C.X. A novel phase locked loop for grid-connected converters under non-ideal grid conditions. J. Power Electron. 2015, 15, 216–226. [Google Scholar] [CrossRef]
- Wang, B.; San, G.; Guo, X.; Wu, W. Grid synchronization and PLL for distributed power generation systems. Proc. CSEE 2013, 33, 50–55. [Google Scholar]




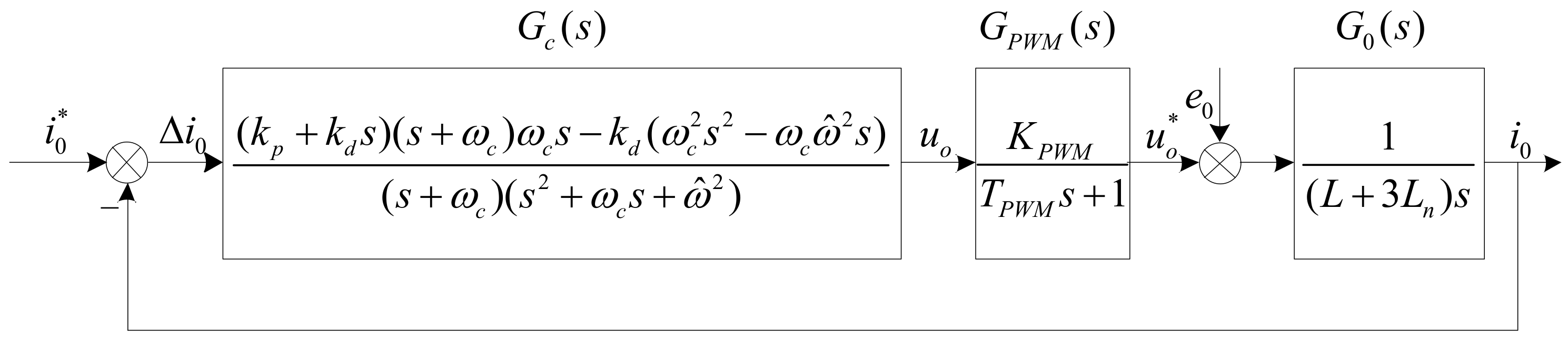



| Parameter | Symbol | Value |
|---|---|---|
| Voltage (line voltage) | u | 380 V |
| Three-phase inductor | L | 0.5 mH |
| Zero line inductor | Ln | 0.5 mH |
| Switching frequency | f | 10 kHz |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, L.; Feng, C.; Zhao, Y.; Liu, J. Zero-Axis Virtual Synchronous Coordinate Based Current Control Strategy for Grid-Connected Inverter. Energies 2018, 11, 1225. https://doi.org/10.3390/en11051225
Yang L, Feng C, Zhao Y, Liu J. Zero-Axis Virtual Synchronous Coordinate Based Current Control Strategy for Grid-Connected Inverter. Energies. 2018; 11(5):1225. https://doi.org/10.3390/en11051225
Chicago/Turabian StyleYang, Longyue, Chunchun Feng, Yan Zhao, and Jianhua Liu. 2018. "Zero-Axis Virtual Synchronous Coordinate Based Current Control Strategy for Grid-Connected Inverter" Energies 11, no. 5: 1225. https://doi.org/10.3390/en11051225




