1. Introduction
Rotaxanes have rightly received considerably attention from synthetic chemistry community [
1,
2,
3]. However, the material properties and physics of these systems are relatively unexplored. There are thus many novel problems and scenarios, with fascinating physics, which are yet to be solved. Some progress has however been made. Early work focussed on so-called slip-link [
4], or sliding polymer, layers [
5,
6] in which there is one ring per axle. More recent work has examined cases where there is more than one ring per axle, particularly for piston-rotaxanes for rod-like axles [
7]. In these systems, one of the rings is connected to an external piston, which controls its position, hence allowing the external agent to access the translational entropy of the rings along the axle. There has been some interest in the effect of flows and forces on these [
8] and their dynamics [
9]. Some work has also been done with flexible polymers as the backbone [
10] and on gels [
11]. What has been emphasised in recent work is the entropic effect of mobile rings on molecular behaviour. If the rings are mobile along the backbone, then the system represents something close to a one-dimensional gas [
7,
12,
13]. If one can change the “volume” of this gas,
i.e., the length of the axle accessible to the rings, by using a piston attached to one of the rings, one can generate novel materials that rely on the pressure of their mobile rings to resist imposed forces.
These systems also have a unique advantage to the physicist, in that their low-dimensionality means that they can be modelled simply and often “exactly”.
The simplest kind of system in this category is the piston-rotaxane (
Figure 1), where
n mobile rings are controlled by one ring attached to an external piston. The physics of this simple system was explored in [
7]. Here, we consider a similar, but slightly different, system in which the axle is not uniform and the rings interact with the axle via a potential,
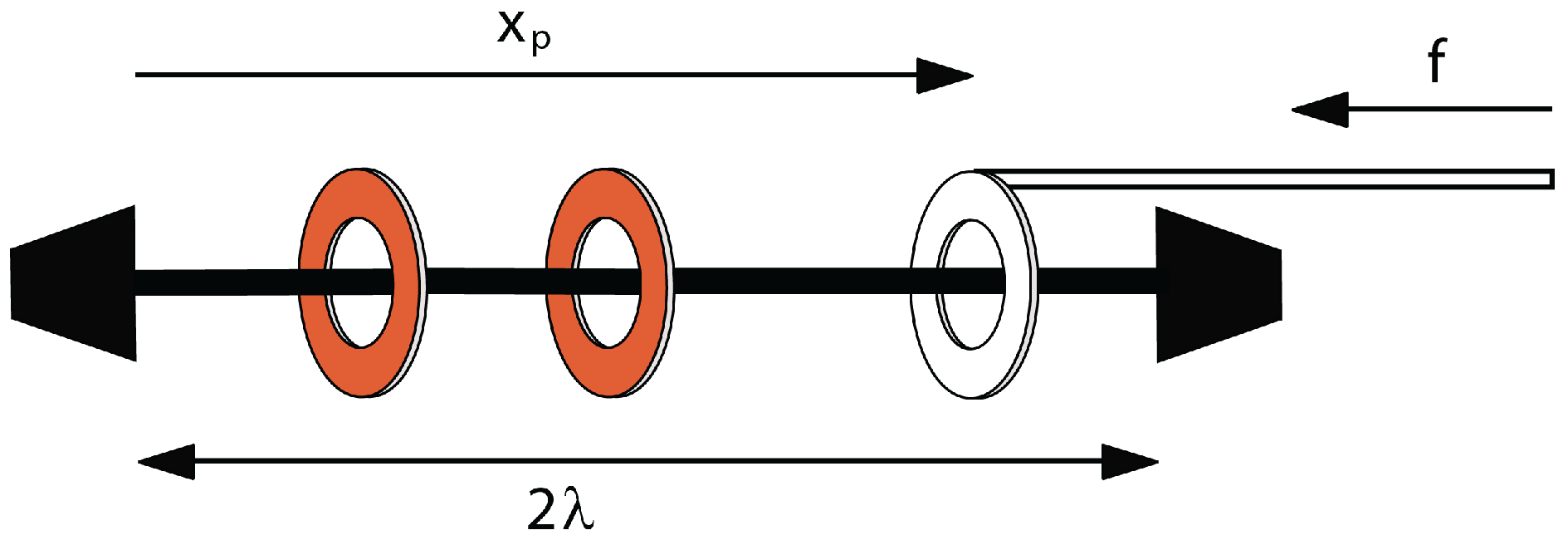
U, which varies along the axle. This is motivated by two things. First, in experiments, one is often confronted by an axle, which has binding stations along it. Second, we can show that this system exhibits some interesting physics. In particular, here, we look at a striped axle (
Figure 2), with only two equal-length stripes, one of high energy and the other of low energy. This system exhibits transitions as the piston is compressed and, in some cases, will show a yield stress in which the extension varies discontinuously as a function of the force.
Figure 1.
A simple piston-rotaxane. The axle has a length of . Here, two mobile rings on the left are compressed by the one piston ring on the right. The position of the piston ring is given by , and it has a force, f, applied to it. The system behaves approximately as a one-dimensional ideal gas.
Figure 1.
A simple piston-rotaxane. The axle has a length of . Here, two mobile rings on the left are compressed by the one piston ring on the right. The position of the piston ring is given by , and it has a force, f, applied to it. The system behaves approximately as a one-dimensional ideal gas.
Figure 2.
A piston-rotaxane with two potential stripes, each of a length of λ. The system behaves approximately as a one-dimensional gas, but the mobile rings now interact with the axle via a potential.
Figure 2.
A piston-rotaxane with two potential stripes, each of a length of λ. The system behaves approximately as a one-dimensional gas, but the mobile rings now interact with the axle via a potential.
A technical point needs to be emphasised at the start. We consider only the case of thermal equilibrium, so that ordinary equilibrium statistical mechanics can be used. This allows us to employ the machinery of partition functions and free energies to make predictions. If experiments are done on short time-scales, then non-equilibrium effects will appear. These are certainly interesting, but are beyond the scope of this paper (for an example of non-equilibrium effects in this piston-rotaxane with a uniform axle, see [
9]).
It should also be noted that there are two distinct kinds of experiments that can be performed when compressing a system. In one, the compression is a given independent variable, controlled from the outside, and what is measured is the average force. In the other, the force is the independent variable, and the average extension is measured. For some common macroscopic systems, like an ideal gas in a container, these two experiments would give identical results. We know that , irrespective of whether we control P or V. For other systems, and in particular, those that show instabilities or contain very few particles, the two kinds of experiments show very different results.
The paper is organised as follows. We first review some simple results for a piston-rotaxane without any backbone potential; this is a purely entropic piston-rotaxane. We then look at the case of a striped system (with two stripes), in which the piston displacement is the independent variable, first with only one mobile ring and, then, with
n mobile rings. Then, we examine the case in which we have force as the independent variable. Both these systems exhibit sharp transitions, but their nature is somewhat different. The detailed calculations can become somewhat involved and are relegated to the
Appendix.
2. The Simple Piston-Rotaxane
Consider a rigid straight axle of a length of
with
n mobile rings, all to one side of another ring, the piston ring, which is attached to another rod (
Figure 1). This is the piston-rotaxane [
7]. We will assume for simplicity that the rings slide freely along the axle and do not interact with it or with each other, except that they cannot pass through the axle or each other. We also assume that the rings take up no volume,
i.e., the excluded volume is zero. This assumption can be easily relaxed, as was done previously [
7,
12], but relaxing it changes the physics very little.
We let be the distance of the piston ring from the far end of the axle. This system can be analysed exactly by calculating the partition function, which is the sum over all possible states of the Boltzmann factor, , where and U is the energy of the system. As we assume no interactions between any of the rings and the axle, U can be set to zero.
For a system of only one mobile ring, the partition function can be written as:
If we have
n identical rings, the partition function is just [
12]:
The free energy is then
, and the average force felt by the piston in equilibrium is
which is just the one-dimensional equivalent of the ordinary ideal gas law,
.
If we allow the force,
f, imposed on the piston to be the independent variable, we have a slightly more complicated calculation. Such a force is equivalent to a potential
acting on the piston ring, which can now take up any position along the rod of a length of
. The partition function is then:
with
.
The average position of the piston ring is then:
Noting that
and
yields the average position
:
where:
This looks very different to the expression , valid when the piston position is the independent variable. There are two differences. The first is a minor factor of compared to n. This arises simply because when force is the independent variable, the piston ring itself is somewhat free to move and increases the effective number of rings by one. The second difference is the term, . This is large if the force is small, i.e., in practice, if , but is negligible at larger forces. This arises because of the constraint that cannot be larger than .
3. Two Stripes with Piston Position As the Independent Variable
The previous section showed that the simple piston-rotaxane system behaves approximately as an ideal gas. In many real rotaxane systems, there are potential stations along the axle that attract the rings. The potential seen by the rings is then a function of position, and the analysis of the simple piston-rotaxane needs to be modified. We start this here by examining the simplest system possible, with two potential stripes of equal size.
We divide the axle into two equal regions of a length of
λ, which interact differently with the mobile rings (
Figure 2). The one farthest from the piston, stripe 2, has a potential
, where
A is a constant. Each mobile ring in this region experiences an addition to its energy of
. In the other region (closest to the piston end), stripe 1, the addition to the energy is zero. In practice, since
A can be positive or negative, and since the energy origin is arbitrary, this covers all possible scenarios of a repulsive or attractive stripe. The major limitations are that the stripe widths are equal, and the piston ring itself experiences no potential. Both of these assumptions can be readily relaxed, but we keep them in order to keep the results as simple as possible.
The algorithm for calculating the average force felt by the piston is the same as for the non-striped rotaxane. The calculations appear in the
Appendix. The free energy is:
The average force is Equation (
16):
As the piston ring crosses between the stripes, there is a jump in the force of
. This jump (
Figure 3) is most easily understood in the case where
. Let us assume that the piston is gradually decompressed, beginning at
close to zero. As long as
, all the rings are confined to stripe 2, and we are just decompressing a gas in a uniform potential. The force is that for a simple gas
However, as soon as the piston crosses into stripe 1, some of the rings can jump into a region of much lower potential. As the piston ring crosses the stripe boundary, the free energy is continuous, but the force undergoes a discontinuous jump upwards, because the rings move from a region of a size of
λ to one which is of a size of
. Further increasing of
decreases the force.
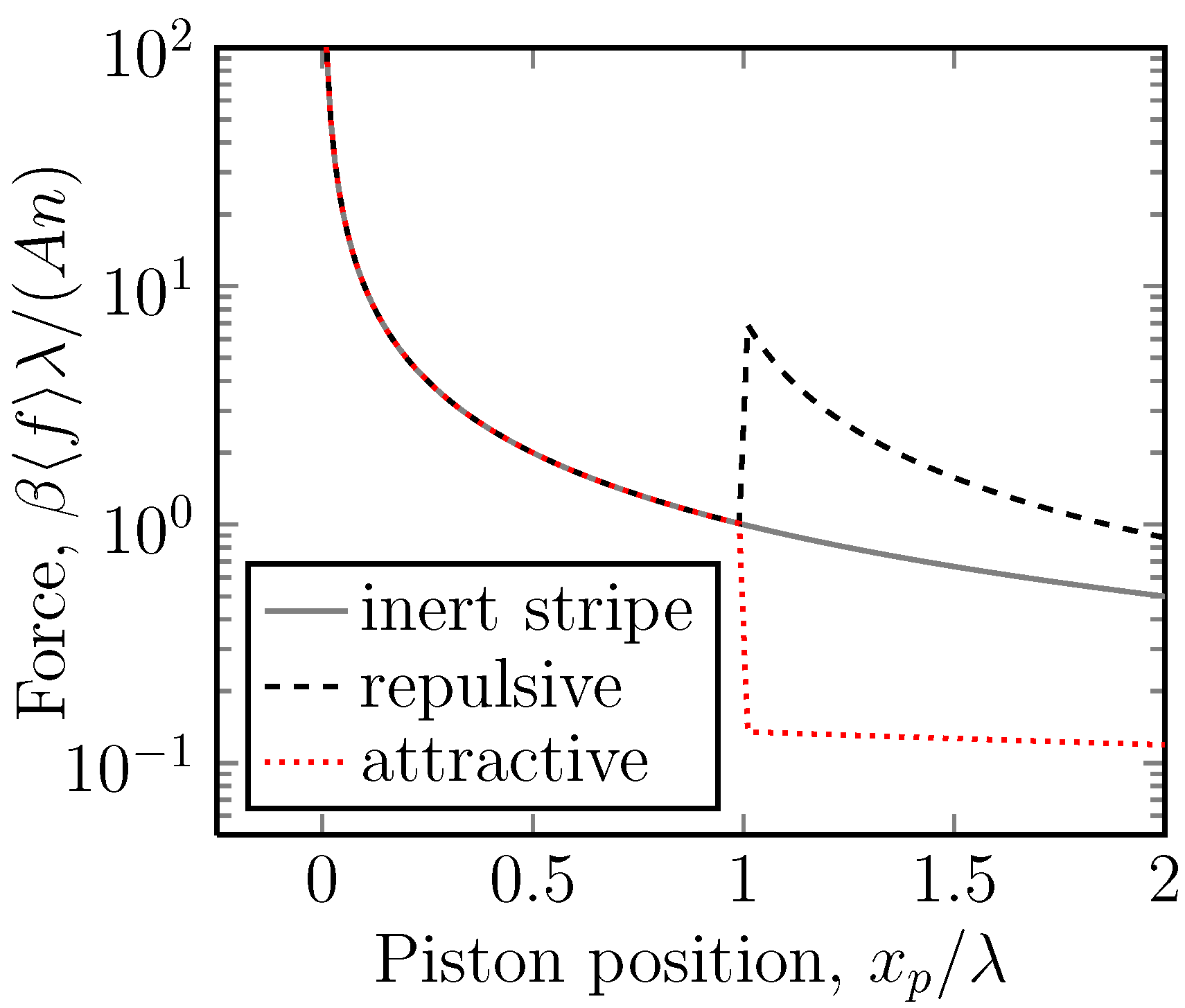
Figure 3.
A semi-log plot of the dimensionless mean force, , versus the position of the piston ring, , for the case of a potential (i.e., ) acting on stripe 2. Stripe 2 is thus repulsive. Here, the position of the piston ring, , is the independent variable and is imposed on the system by the piston. The average force, , of the mobile ring on this is the measured quantity. This is the case for one mobile ring. In the case of n mobile rings, the force is multiplied by a factor of n. Under gradual compression, the force gradually increases, until the interface between the stripes is reached, when a sudden decrease is observed.
Figure 3.
A semi-log plot of the dimensionless mean force, , versus the position of the piston ring, , for the case of a potential (i.e., ) acting on stripe 2. Stripe 2 is thus repulsive. Here, the position of the piston ring, , is the independent variable and is imposed on the system by the piston. The average force, , of the mobile ring on this is the measured quantity. This is the case for one mobile ring. In the case of n mobile rings, the force is multiplied by a factor of n. Under gradual compression, the force gradually increases, until the interface between the stripes is reached, when a sudden decrease is observed.
What is slightly counterintuitive about this problem is that under compression, the free energy always increases, but the force, which is the negative of the slope of the free energy, is not monotonic and undergoes a discontinuous jump.
There are in fact two cases to consider. The most interesting case is when
, so that under compression, one is first compressing a gas in the lower potential region. There is then a negative jump in the force under compression. In the opposite case in which
(
Figure 4), the initial compression is easy, since there are few particles in stripe 1, and the eventual jump in the force is positive.
Figure 4.
A semi-log plot of the dimensionless mean force, , versus the position of the piston ring, , for the case of a potential (i.e., ) acting on stripe 2. Stripe 2 is then attractive. Here, the position of the piston ring, , is the independent variable and is imposed on the system by the piston. The average force, , of the mobile ring on this is the measured quantity. This is the case for one mobile ring. In the case of n mobile rings, the force is multiplied by a factor of n. Under gradual compression, the force gradually increases until the interface between the stripes is reached, when a sudden increase is observed.
Figure 4.
A semi-log plot of the dimensionless mean force, , versus the position of the piston ring, , for the case of a potential (i.e., ) acting on stripe 2. Stripe 2 is then attractive. Here, the position of the piston ring, , is the independent variable and is imposed on the system by the piston. The average force, , of the mobile ring on this is the measured quantity. This is the case for one mobile ring. In the case of n mobile rings, the force is multiplied by a factor of n. Under gradual compression, the force gradually increases until the interface between the stripes is reached, when a sudden increase is observed.
Figure 5.
A semi-log plot of the dimensionless mean force,
,
versus the position of the piston ring,
, for potentials
(stripe 2 attractive),
(both stripes equal, inert) and
(stripe 2 repulsive). Here, the position of the piston ring,
, is the independent variable and is imposed on the system by the piston. The average force,
, of the mobile ring on this is the measured quantity. This is the case for one mobile ring. In the case of
n mobile rings, the force is multiplied by a factor of
n. The inert system is the simple piston-rotaxane of
Figure 1.
Figure 5.
A semi-log plot of the dimensionless mean force,
,
versus the position of the piston ring,
, for potentials
(stripe 2 attractive),
(both stripes equal, inert) and
(stripe 2 repulsive). Here, the position of the piston ring,
, is the independent variable and is imposed on the system by the piston. The average force,
, of the mobile ring on this is the measured quantity. This is the case for one mobile ring. In the case of
n mobile rings, the force is multiplied by a factor of
n. The inert system is the simple piston-rotaxane of
Figure 1.
The case in which there is more than one mobile ring is relatively straightforward (see
appendix). For
n mobile rings, the average force is multiplied by
n (
Figure 5), so that Equation (
21):
4. Two Stripes with Force as the Independent Variable
We now keep the system the same as in the previous section, but apply a given force,
f, to the piston. As shown in the
Appendix, for one mobile ring, the partition function is Equation (
24):
where
is a dimensionless measure of the force.
Again, one can get the average position by differentiating the partition function with respect to the force, Equation (
25):
For
, this produces an extension
versus the force curve, like that shown in
Figure 6. The most notable feature is a sudden drop in the extension at a critical force,
i.e., the system shows a yield stress. Also notable is the great difference between this curve and the equivalent curve when
is the independent variable (
Figure 3).
Figure 6.
A linear plot of the dimensionless piston position, , versus the dimensionless applied force on the piston ring, , for the case of different potentials acting on stripe 2. Stripe 2 ranges from attractive to repulsive. Here, the position of the piston ring, , is the measured quantity, and the applied force on the piston, f, is the independent variable. This is the case for one mobile ring. Even though we only have one mobile ring, for positive A, i.e., repulsive potentials, we have a sudden drop in the displacement at a critical force. The system thus exhibits yield stress behaviour.
Figure 6.
A linear plot of the dimensionless piston position, , versus the dimensionless applied force on the piston ring, , for the case of different potentials acting on stripe 2. Stripe 2 ranges from attractive to repulsive. Here, the position of the piston ring, , is the measured quantity, and the applied force on the piston, f, is the independent variable. This is the case for one mobile ring. Even though we only have one mobile ring, for positive A, i.e., repulsive potentials, we have a sudden drop in the displacement at a critical force. The system thus exhibits yield stress behaviour.
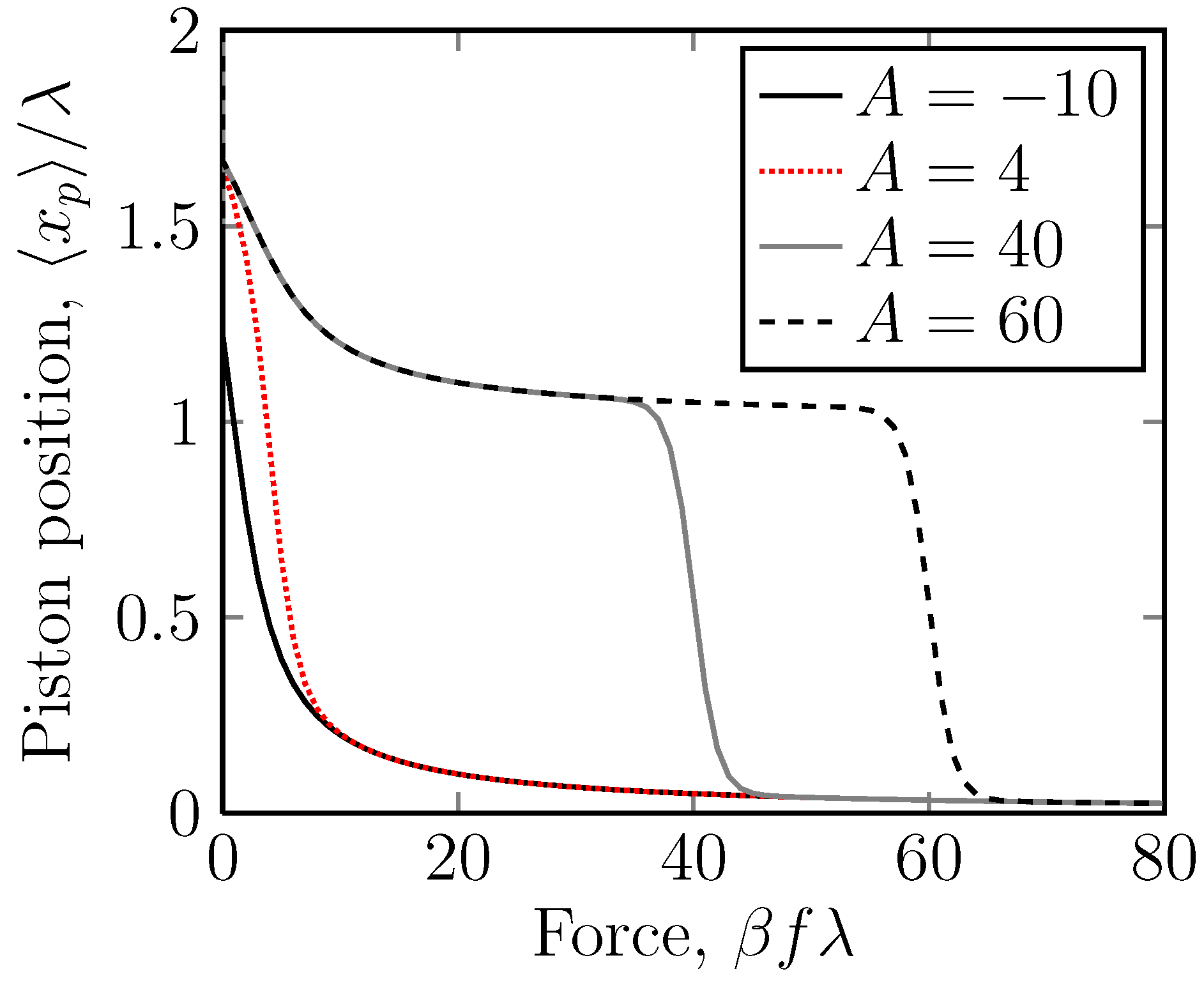
For a system with
n mobile rings, the calculation is even more involved, but following the
Appendix, we can draw curves of
versus f for different
n (
Figure 7). As
n gets larger, the transition becomes sharper. From
Figure 7 and
Figure 8, it is clear that for large
n or
A, the transition obeys the scaling
Figure 7.
A linear plot of the dimensionless piston position, , versus the dimensionless applied force on the piston ring, , for the case of a potential acting on stripe 2. Stripe 2 is thus repulsive. Here, the position of the piston ring, , is the measured quantity and the applied force on the piston, f, is the independent variable. The number of rings, is varied. Again, we have a sudden drop in the displacement at a critical force. Note, also, that the scaling, , shows that the critical force always lies at
Figure 7.
A linear plot of the dimensionless piston position, , versus the dimensionless applied force on the piston ring, , for the case of a potential acting on stripe 2. Stripe 2 is thus repulsive. Here, the position of the piston ring, , is the measured quantity and the applied force on the piston, f, is the independent variable. The number of rings, is varied. Again, we have a sudden drop in the displacement at a critical force. Note, also, that the scaling, , shows that the critical force always lies at
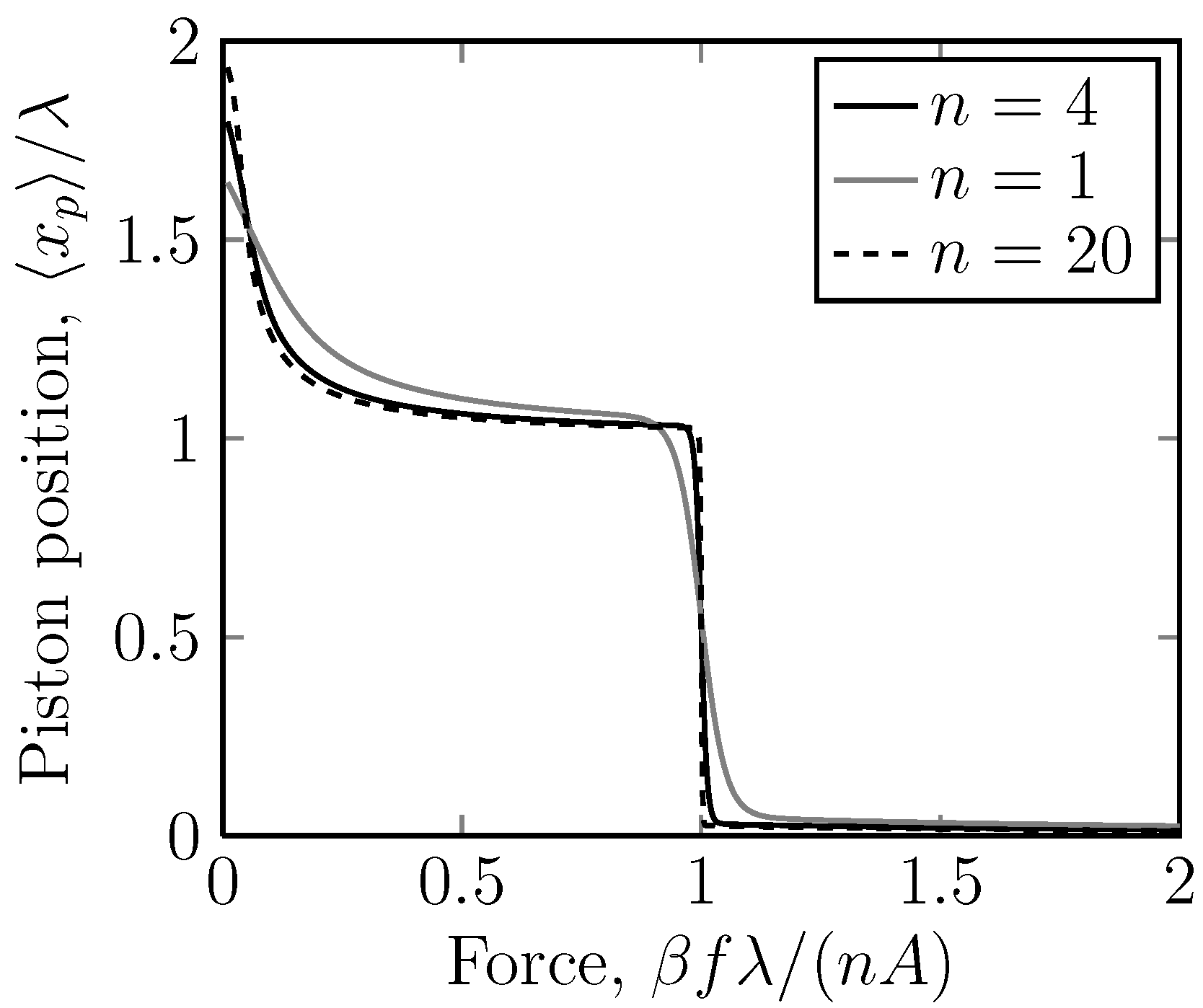
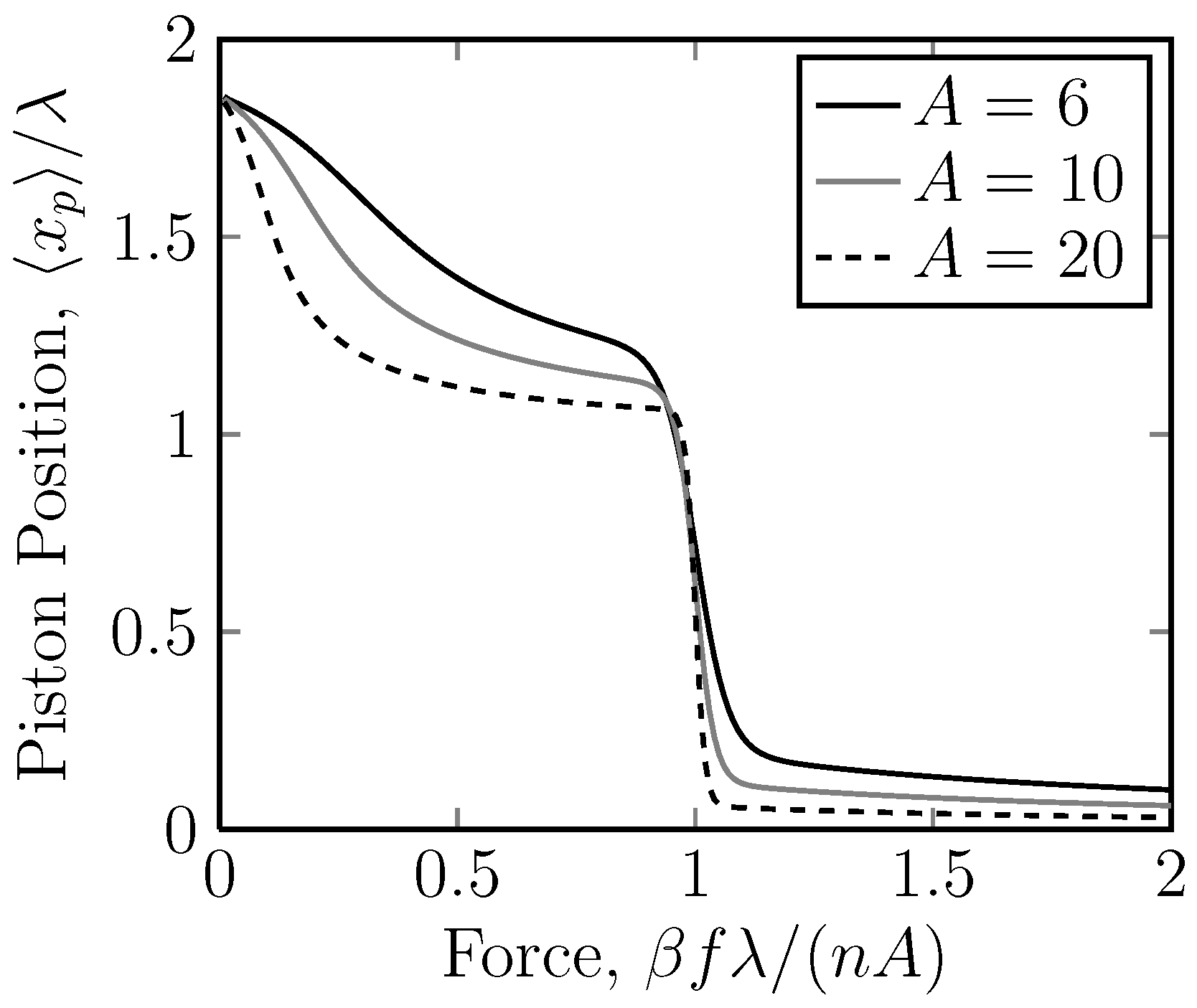
Figure 8.
A linear plot of the dimensionless piston position, , versus the dimensionless applied force on the piston ring, , for the case of different potentials acting on stripe 2. Stripe 2 is thus repulsive. Here, the position of the piston ring, , is the measured quantity and the applied force on the piston, and f is the independent variable. The number of rings is . Again, we have a sudden drop in the displacement at a critical force. Note, also, that the scaling, , shows that the critical force always lies at
Figure 8.
A linear plot of the dimensionless piston position, , versus the dimensionless applied force on the piston ring, , for the case of different potentials acting on stripe 2. Stripe 2 is thus repulsive. Here, the position of the piston ring, , is the measured quantity and the applied force on the piston, and f is the independent variable. The number of rings is . Again, we have a sudden drop in the displacement at a critical force. Note, also, that the scaling, , shows that the critical force always lies at
The case of
(
Figure 6) is, as expected, less interesting. There is no sharp transition, and the extension gradually decreases as the force is increased.
5. Conclusions
We have reviewed some previous results for piston-rotaxanes in which the physics can be approximated by that of a one-dimensional ideal gas. We then studied what happens in the presence of a potential acting on the rings. If the potential is in the form of stripes, we can obtain sudden jumps in either the force or the displacement, depending on what is chosen as the independent variable. In particular, for the case of a given applied force, the system can exhibit a yield stress, whereby, below a critical force, the system is only weakly compressible, but for larger forces, it collapses suddenly.
Our system consists of two stripes. However, it can readily be applied to a rotaxane with many attractive stations along the backbone, provided the rings can hop between stations during the course of the experiment. There are some obvious extensions of this work to more than two stripes or to more gradually varying potentials. There would also be some interest in non-equilibrium effects.